What is the slope of the line?
Understand the Problem
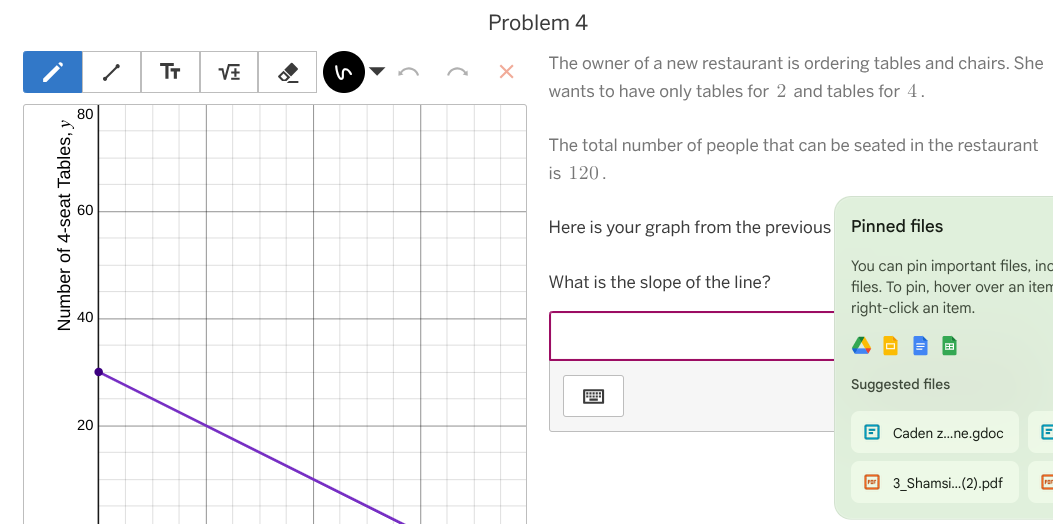
The problem describes a scenario where a restaurant owner wants to order tables for 2 and tables for 4, with a total seating capacity of 120. A graph related to this scenario is provided, and the question asks to find the slope of the line on this graph.
Answer
$ -\frac{1}{2} $
Answer for screen readers
$ -\frac{1}{2} $
Steps to Solve
-
Identify two points on the line From the graph, we can identify two points where the line intersects grid lines. These points appear to be $(0, 30)$ and $(60, 0)$.
-
Apply the slope formula The slope $m$ of a line passing through two points $(x_1, y_1)$ and $(x_2, y_2)$ is given by the formula: $$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
-
Substitute the coordinates of the points into the slope formula Using the points $(0, 30)$ and $(60, 0)$, we have: $$ m = \frac{0 - 30}{60 - 0} $$
-
Simplify the expression to find the slope $$ m = \frac{-30}{60} = -\frac{1}{2} $$
$ -\frac{1}{2} $
More Information
The slope of the line is -1/2, which indicates that for every increase of 2-seat tables by one, the number of 4-seat tables decreases by 1/2 * 1 = 0.5 tables.
Tips
A common mistake that can be made when finding the slope, is to mix up the order of the x and y coordinates when applying the slope formula. Ensure that you subtract the y-coordinates in the numerator and the x-coordinates in the denominator, maintaining consistency in the order of subtraction. Another mistake is made when reading the coordinates off the graph: make sure to read coordinates accurately.
AI-generated content may contain errors. Please verify critical information