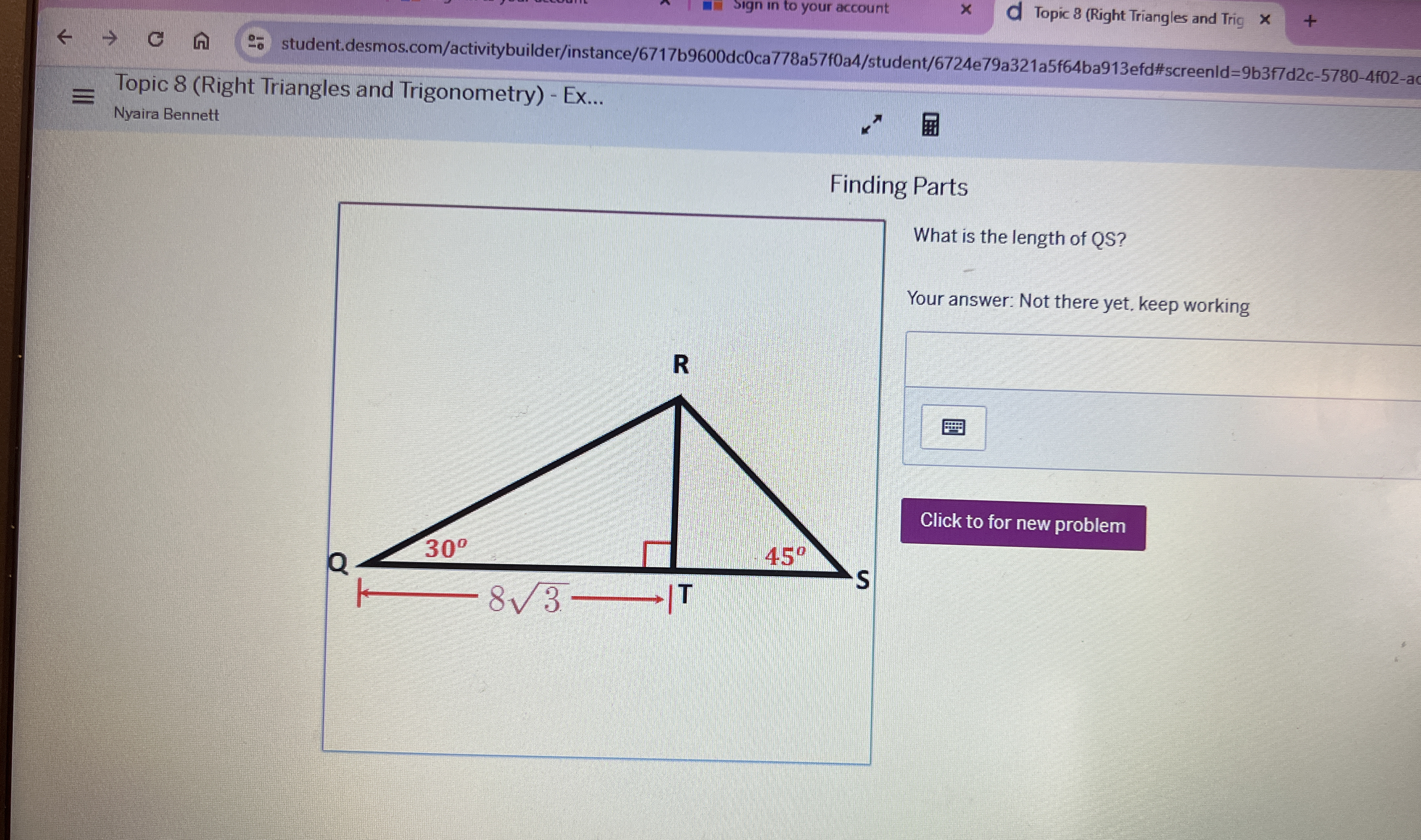
What is the length of QS?
Understand the Problem
The question is asking for the length of side QS in a right triangle where two angles and one side length are known. We will apply trigonometric principles to solve for the unknown length.
Answer
The length of side \( QS \) is \( 4\sqrt{3} \).
Answer for screen readers
The length of side ( QS ) is ( 4\sqrt{3} ).
Steps to Solve
-
Identify known values and angles The triangle has one side ( ST ) with length ( 8\sqrt{3} ) and angles ( 30^\circ ) and ( 45^\circ ) at points ( R ) and ( S ) respectively.
-
Use trigonometric relationships We can use the sine function for angle ( Q ) to find the length of side ( QS ). According to the sine rule: $$ \sin(30^\circ) = \frac{\text{opposite}}{\text{hypotenuse}} $$ Here, ( QS ) is the opposite side and ( ST ) is the hypotenuse.
-
Substitute known values First, note that: $$ \sin(30^\circ) = \frac{1}{2} $$ Thus we have: $$ \frac{QS}{8\sqrt{3}} = \frac{1}{2} $$
-
Solve for ( QS ) Multiply both sides by ( 8\sqrt{3} ) to isolate ( QS ): $$ QS = \frac{1}{2} \times 8\sqrt{3} $$ $$ QS = 4\sqrt{3} $$
The length of side ( QS ) is ( 4\sqrt{3} ).
More Information
In the context of trigonometry, a ( 30^\circ-60^\circ-90^\circ ) triangle typically has side lengths in the ratio ( 1:\sqrt{3}:2 ). The calculations were also based on the sine function which is fundamental in trigonometry.
Tips
- Forgetting to use the correct side-length relationships for different angles can lead to incorrect values.
- Misapplying trigonometric ratios can also produce wrong results, so it's important to double-check which side corresponds to the angle given.
AI-generated content may contain errors. Please verify critical information