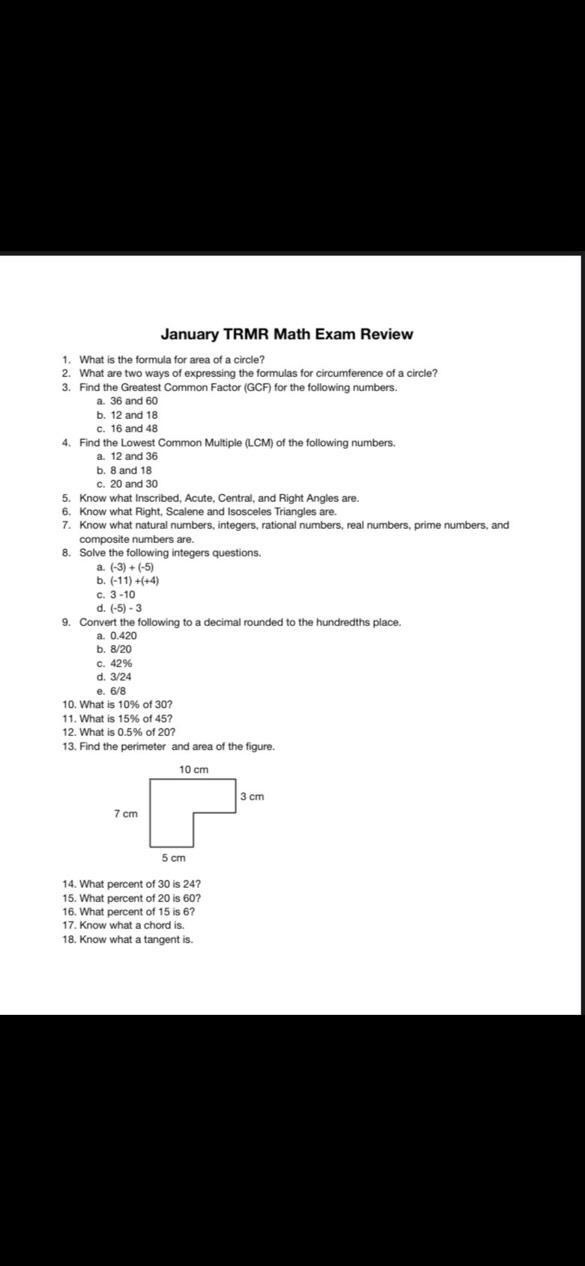
What is the formula for area of a circle? What are two ways of expressing the formulas for circumference of a circle? Find the Greatest Common Factor (GCF) for the following number... What is the formula for area of a circle? What are two ways of expressing the formulas for circumference of a circle? Find the Greatest Common Factor (GCF) for the following numbers: 36 and 60; 12 and 18; 16 and 48. Find the Lowest Common Multiple (LCM) of the following numbers: 12 and 36; 8 and 18; 20 and 30. Know what Inscribed, Acute, Central, and Right Angles are. Know what Right, Scalene and Isosceles Triangles are. Know what natural numbers, integers, rational numbers, real numbers, prime numbers, and composite numbers are. Solve the following integers questions: (3) - (-5); (-1) + (+4); 3 - 10; (-5) - 3. Convert the following to a decimal rounded to the hundredths place: 0.420; 8/20; 62%. What is 15% of 30? What is 15% of 45? What is 0.5% of 207? Find the perimeter and area of the figure (dimensions given). What percent of 30 is 24? What percent of 20 is 60? What percent of 15 is 6? Know what a chord is. Know what a tangent is. Read more

Steps to Solve

1. Formula for Area of a Circle The formula for the area $A$ of a circle is given by: $$ A = \pi r^2 $$ where $r$ is the radius of the circle.

2. Circumference of a Circle Two ways to express the circumference $C$ of a circle are:

• $C = 2\pi r$ (using the radius)
• $C = \pi d$ (using the diameter, where $d = 2r$)

3. Calculate GCF of 36 and 60 To find GCF:

• List the factors:
  • Factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36
  • Factors of 60: 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, 60
• The common factors: 1, 2, 3, 4, 6, 12
• GCF is the greatest common factor: 12

4. Calculate GCF of 12 and 18 Factors of 12: 1, 2, 3, 4, 6, 12
   Factors of 18: 1, 2, 3, 6, 9, 18
   Common factors: 1, 2, 3, 6
   GCF: 6

5. Calculate GCF of 16 and 48 Factors of 16: 1, 2, 4, 8, 16
   Factors of 48: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48
   Common factors: 1, 2, 4, 8, 16
   GCF: 16

6. Calculate LCM of 12 and 36 List multiples:

• Multiples of 12: 12, 24, 36, 48, ...
• Multiples of 36: 36, 72, 108, ... LCM is the smallest common multiple: 36

7. Calculate LCM of 8 and 18 Multiples of 8: 8, 16, 24, 32, 40, 48, ...
   Multiples of 18: 18, 36, 54, ...
   LCM: 72

8. Calculate LCM of 20 and 30 Multiples of 20: 20, 40, 60, ...
   Multiples of 30: 30, 60, 90, ...
   LCM: 60

9. Angles Knowledge

• Inscribed angle: An angle formed by two chords.
• Central angle: An angle whose vertex is at the center of the circle.
• Right angle: An angle of 90 degrees.
• Acute angle: An angle less than 90 degrees.
• Obtuse angle: An angle greater than 90 degrees but less than 180 degrees.

10. Triangles Knowledge

• Right triangle: One angle is 90 degrees.
• Scalene triangle: All sides are of different lengths.
• Isosceles triangle: Two sides are of equal length.

11. Solve Integer Expressions a. $(-3) - (-5) = -3 + 5 = 2$
    b. $(-1) + 4 = 3$
    c. $-3 - 10 = -13$
    d. $-5 - 3 = -8$

12. Convert Decimals a. Convert 0.420 to hundredths: 0.42
    b. Convert 8/20 to decimal: $8 \div 20 = 0.4$
    c. Convert 42% to decimal: $0.42$
    d. Convert 3/24 to decimal: $3 \div 24 = 0.125$
    e. Convert 6/8 to decimal: $6 \div 8 = 0.75$

13. Calculate Percentages a. $10%$ of $30$:
    $$ 0.10 \times 30 = 3 $$

b. $15%$ of $45$:
$$ 0.15 \times 45 = 6.75 $$

c. $0.5%$ of $207$:
$$ 0.005 \times 207 = 1.035 $$

14. Find Perimeter and Area of Figure Perimeter = $10 + 7 + 5 + 3 + 3 = 28 \text{ cm}$
    Area: Break it into rectangles and apply:

• Rectangle 1: $10 \times 7 = 70 \text{ cm}^2$
• Rectangle 2: $3 \times 5 = 15 \text{ cm}^2$
  Total Area = $70 - 15 = 55 \text{ cm}^2$

15. Percent Calculations a. What percent of 30 is 24?
    $$ \text{Percent} = \left( \frac{24}{30} \right) \times 100 = 80% $$

b. What percent of 20 is 60?
$$ \text{Percent} = \left( \frac{60}{20} \right) \times 100 = 300% $$

c. What percent of 15 is 6?
$$ \text{Percent} = \left( \frac{6}{15} \right) \times 100 = 40% $$

16. Definitions

• Chord: A line segment joining two points on the circumference of a circle.
• Tangent: A line that touches a circle at exactly one point.