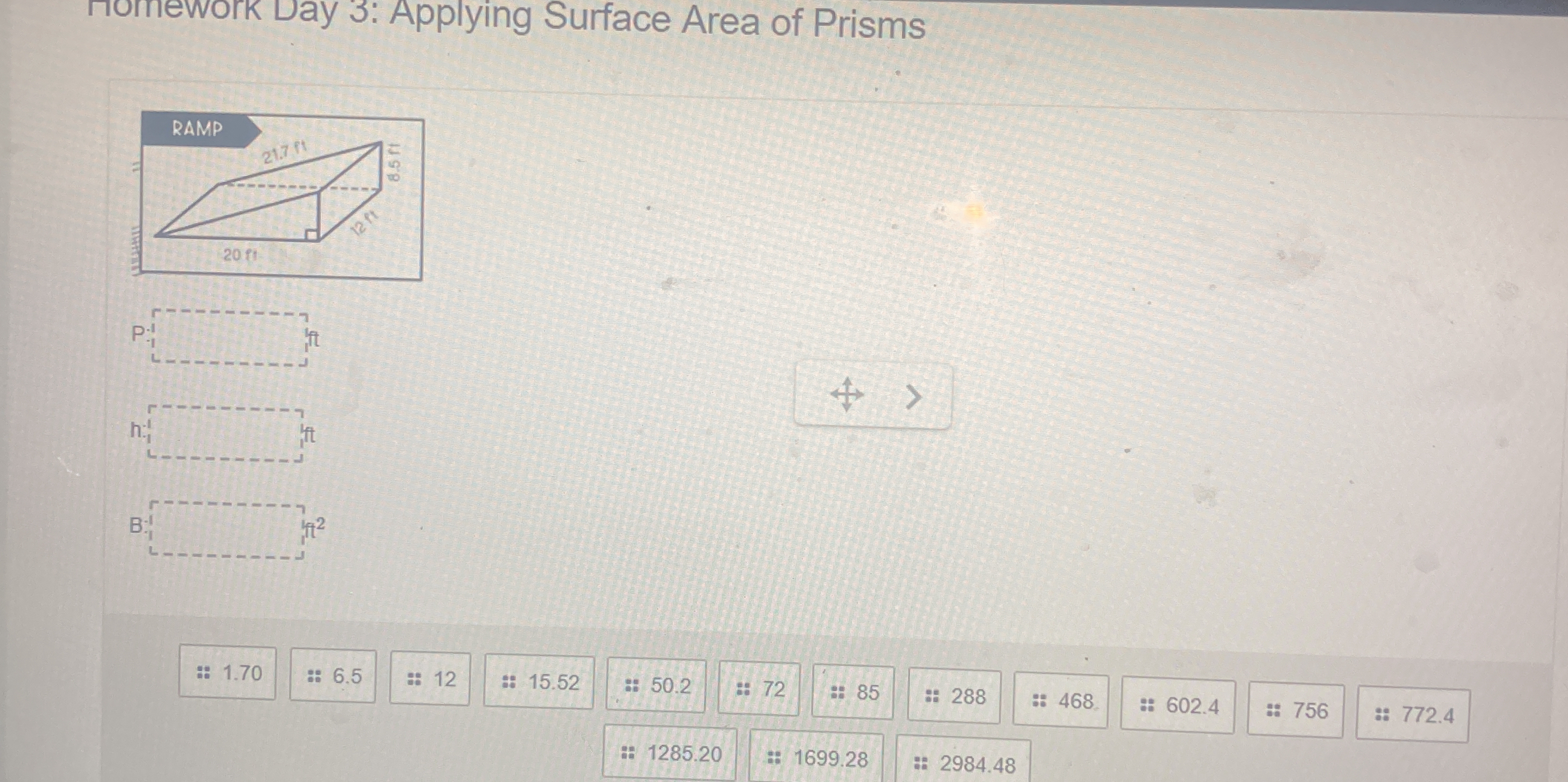
What are the perimeter, height, and base area of the prism depicted in the image?
Understand the Problem
The question involves calculating the perimeter, height, and base area of a prism based on the provided dimensions in the image.
Answer
$P = 118.7 \, \text{ft}, \, h = 12 \, \text{ft}, \, B = 250.2 \, \text{ft}^2$
Answer for screen readers
- Perimeter (P): $P = 118.7 , \text{ft}$
- Height (h): $h = 12 , \text{ft}$
- Base Area (B): $B = 250.2 , \text{ft}^2$
Steps to Solve
- Calculate the Perimeter (P)
To find the perimeter of the base of the prism, we will sum the lengths of all the sides of the base. The base is a trapezoid.
The lengths of the sides are:
- 20 ft
- 12 ft
- 21.7 ft
- 65 ft
So, the formula for the perimeter is: $$ P = 20 + 12 + 21.7 + 65 $$
- Calculate the Height (h)
The height of the prism is the vertical distance between the two bases. The height is given in the problem:
$$ h = 12 , \text{ft} $$
- Calculate the Base Area (B)
The base area of the trapezoidal base can be calculated using the formula: $$ B = \frac{(b_1 + b_2) \cdot h}{2} $$
Where ( b_1 ) and ( b_2 ) are the lengths of the two parallel sides (20 ft and 21.7 ft), and ( h ) is the height of the trapezoid, which in this case is also 12 ft:
$$ B = \frac{(20 + 21.7) \cdot 12}{2} $$
- Perform the Calculations
Now, we can replace the terms and perform the calculations from previous steps.
For Perimeter: $$ P = 20 + 12 + 21.7 + 65 = 118.7 , \text{ft} $$
For Base Area: $$ B = \frac{(20 + 21.7) \cdot 12}{2} = \frac{41.7 \cdot 12}{2} = \frac{500.4}{2} = 250.2 , \text{ft}^2 $$
- Perimeter (P): $P = 118.7 , \text{ft}$
- Height (h): $h = 12 , \text{ft}$
- Base Area (B): $B = 250.2 , \text{ft}^2$
More Information
The perimeter represents the total length around the base of the prism. The height is the vertical length supporting the prism, while the base area provides the amount of space contained in the base itself. This is useful in architectural designs and constructions.
Tips
- Forgetting to add all sides when calculating perimeter can result in an incorrect answer. Ensure all sides are included.
- Misidentifying the height of the prism; it's given as part of the problem, and should not be estimated.
- Mixing up dimensions when calculating the base area of a trapezoid can lead to wrong conclusions.
AI-generated content may contain errors. Please verify critical information