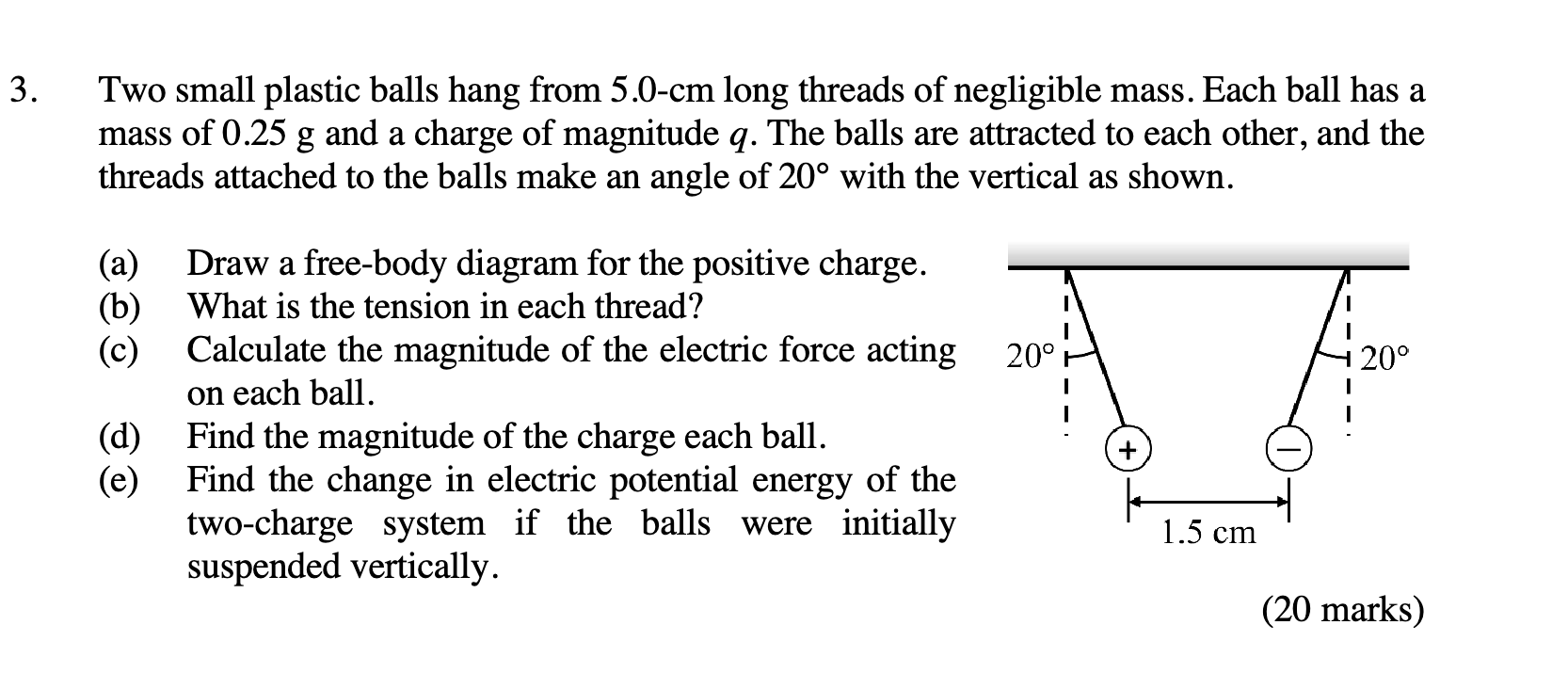
Two small plastic balls hang from 5.0-cm long threads of negligible mass. Each ball has a mass of 0.25 g and a charge of magnitude q. The balls are attracted to each other, and the... Two small plastic balls hang from 5.0-cm long threads of negligible mass. Each ball has a mass of 0.25 g and a charge of magnitude q. The balls are attracted to each other, and the threads attached to the balls make an angle of 20 degrees with the vertical as shown. (a) Draw a free-body diagram for the positive charge. (b) What is the tension in each thread? (c) Calculate the magnitude of the electric force acting on each ball. (d) Find the magnitude of the charge each ball. (e) Find the change in electric potential energy of the two-charge system if the balls were initially suspended vertically. Read more

Steps to Solve

1. Draw the free-body diagram

The forces acting on the positive charge are:

• Tension $T$ in the string, acting at an angle of $20^\circ$ with the vertical
• Gravitational force $mg$ acting downwards
• Electric force $F_e$ acting horizontally towards the negative charge

2. Calculate the tension in the thread

Since the ball is in equilibrium, the net force in both the horizontal and vertical directions must be zero. Vertical components: $T\cos(20^\circ) = mg$ $T = \frac{mg}{\cos(20^\circ)}$ Given $m = 0.25 , \text{g} = 0.25 \times 10^{-3} , \text{kg}$ and $g = 9.8 , \text{m/s}^2$, $T = \frac{0.25 \times 10^{-3} , \text{kg} \times 9.8 , \text{m/s}^2}{\cos(20^\circ)}$ $T \approx \frac{0.00245}{0.9397} \approx 0.0026 , \text{N}$

3. Calculate the electric force

Horizontal components: $F_e = T\sin(20^\circ)$ $F_e = 0.0026 , \text{N} \times \sin(20^\circ)$ $F_e \approx 0.0026 \times 0.3420 \approx 0.00089 , \text{N}$

4. Calculate the magnitude of the charge

The distance between the charges is $r = 1.5 , \text{cm} = 0.015 , \text{m}$. Using Coulomb's law: $F_e = k \frac{q^2}{r^2}$, where $k = 8.99 \times 10^9 , \text{N m}^2/\text{C}^2$ $q^2 = \frac{F_e r^2}{k}$

$q^2 = \frac{0.00089 , \text{N} \times (0.015 , \text{m})^2}{8.99 \times 10^9 , \text{N m}^2/\text{C}^2}$ $q^2 = \frac{0.00089 \times 0.000225}{8.99 \times 10^9} = \frac{2.0025 \times 10^{-7}}{8.99 \times 10^9} \approx 2.227 \times 10^{-17} , \text{C}^2$ $q = \sqrt{2.227 \times 10^{-17}} \approx 4.72 \times 10^{-9} , \text{C}$

5. Calculate the change in electric potential energy

The initial potential energy when the balls are vertically aligned is undefined as the distance $r$ tends to zero, making $U$ tend to $-\infty$.

When the balls are at $20^\circ$: $U = \frac{kq_1q_2}{r}$ Since the charges are opposite, $U = -k \frac{q^2}{r}$ $U = -8.99 \times 10^9 \frac{(4.72 \times 10^{-9})^2}{0.015} = -8.99 \times 10^9 \frac{2.227 \times 10^{-17}}{0.015}$ $U = -8.99 \times 10^9 \times 1.485 \times 10^{-15} = -1.335 \times 10^{-5} , \text{J}$

The vertical height when the balls are at $20^\circ$: $h = L\cos(20^\circ) = 5 , \text{cm} \times \cos(20^\circ) = 0.05 , \text{m} \times 0.9397 = 0.046985 , \text{m}$

When the balls are suspended vertically, the distance $r$ is 0. When the balls are suspended vertically, the distance between them is $L$: $\Delta y = L - L\cos(20^\circ) = 0.05 - 0.046985 = 0.003015 , \text{m}$

$U_i = -\infty$ $U_f = -1.335 \times 10^{-5} , \text{J}$

Therefore $\Delta U = U_f - U_i = -1.335 \times 10^{-5} - (-\infty)$ Because the intial potential energy is undefined we can calculate the change assuming the balls are now separated by a large distance:

$\Delta U \approx -1.335 \times 10^{-5} , \text{J}$