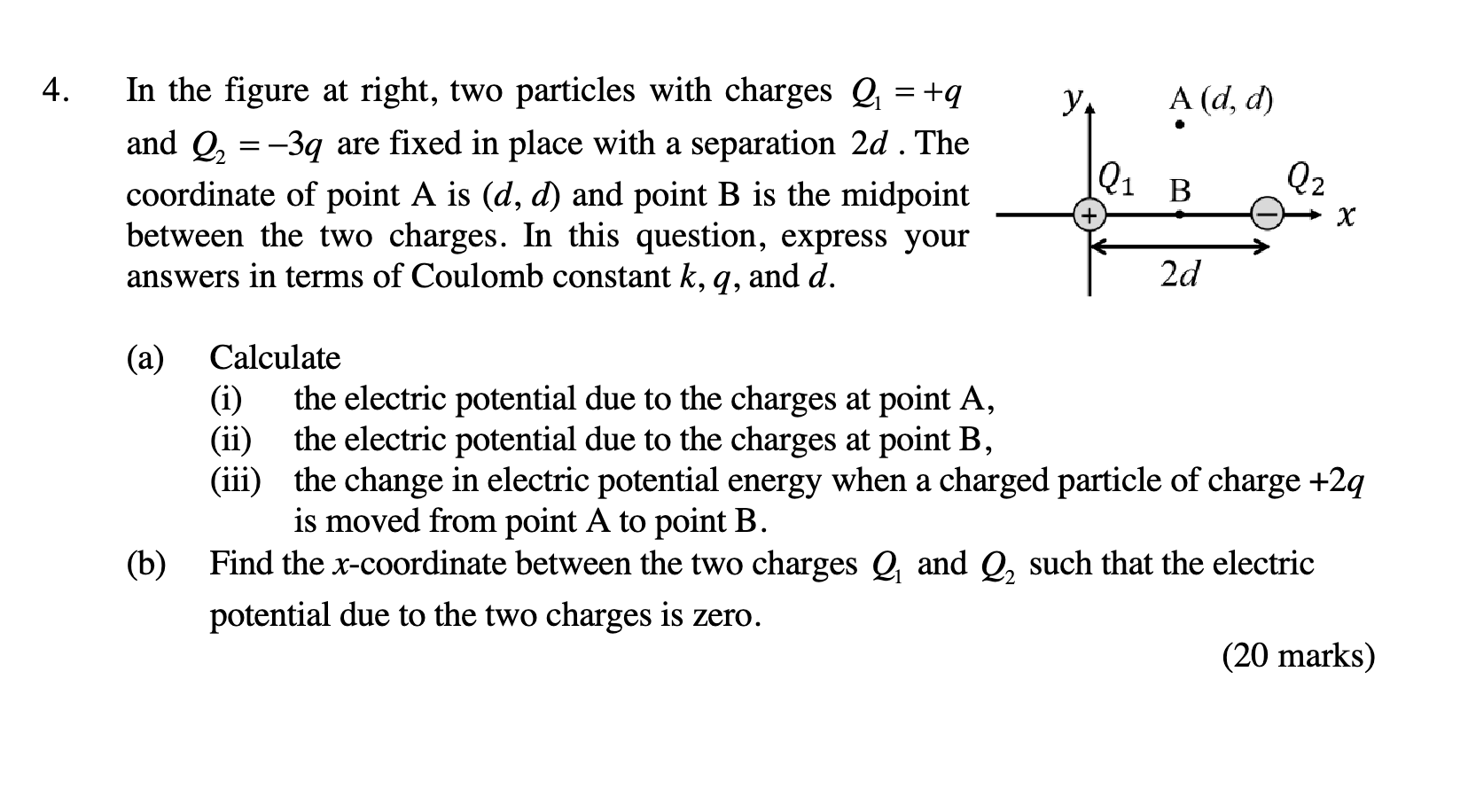
Two particles with charges Q1 = +q and Q2 = -3q are fixed in place with a separation 2d. The coordinate of point A is (d, d) and point B is the midpoint between the two charges. Ex... Two particles with charges Q1 = +q and Q2 = -3q are fixed in place with a separation 2d. The coordinate of point A is (d, d) and point B is the midpoint between the two charges. Express your answers in terms of Coulomb constant k, q, and d. (a) Calculate: (i) the electric potential due to the charges at point A, (ii) the electric potential due to the charges at point B, (iii) the change in electric potential energy when a charged particle of charge +2q is moved from point A to point B. (b) Find the x-coordinate between the two charges Q1 and Q2 such that the electric potential due to the two charges is zero.
Understand the Problem
The question involves electrostatics, specifically calculating electric potential and potential energy due to point charges. Part (a) requires finding the electric potential at two points (A and B) and the change in electric potential energy when a charge is moved between them. Part (b) asks for the location where the electric potential is zero due to the two charges.
Answer
(a) (i) $V_A = \frac{-\sqrt{2}kq}{d}$ (ii) $V_B = \frac{-2kq}{d}$ (iii) $\Delta U = \frac{(-4 + 2\sqrt{2})kq^2}{d}$ (b) $x = \frac{d}{2}$
Answer for screen readers
(a) (i) $V_A = \frac{-\sqrt{2}kq}{d}$ (ii) $V_B = \frac{-2kq}{d}$ (iii) $\Delta U = \frac{(-4 + 2\sqrt{2})kq^2}{d}$
(b) $x = \frac{d}{2}$
Steps to Solve
- (a)(i) Electric potential at point A
The electric potential $V$ due to a point charge $Q$ at a distance $r$ is given by $V = \frac{kQ}{r}$. We need to calculate the potential at point A due to both $Q_1$ and $Q_2$ and sum them up. Point A has coordinates $(d, d)$.
Distance from $Q_1$ to A: $r_1 = \sqrt{(d-0)^2 + (d-0)^2} = \sqrt{d^2 + d^2} = \sqrt{2d^2} = d\sqrt{2}$. Distance from $Q_2$ to A: $Q_2$ is at $x=2d$ so $r_2 = \sqrt{(d-2d)^2 + (d-0)^2} = \sqrt{(-d)^2 + d^2} = \sqrt{d^2 + d^2} = \sqrt{2d^2} = d\sqrt{2}$. We know that $Q_1 = +q$ and $Q_2 = -3q$.
The total potential at point A is: $V_A = \frac{kQ_1}{r_1} + \frac{kQ_2}{r_2} = \frac{kq}{d\sqrt{2}} + \frac{k(-3q)}{d\sqrt{2}} = \frac{kq}{d\sqrt{2}} - \frac{3kq}{d\sqrt{2}} = \frac{-2kq}{d\sqrt{2}} = \frac{-\sqrt{2}kq}{d}$
- (a)(ii) Electric potential at point B
Point B is the midpoint between the two charges. The charges are separated by a distance $2d$, so B is a distance $d$ from each charge. The coordinate of $Q_1$ is $0$ and $Q_2$ is $2d$. Therefore the midpoint should be $d$.
Distance from $Q_1$ to B: $r_1 = d$. Distance from $Q_2$ to B: $r_2 = d$.
The total potential at point B is: $V_B = \frac{kQ_1}{r_1} + \frac{kQ_2}{r_2} = \frac{kq}{d} + \frac{k(-3q)}{d} = \frac{kq}{d} - \frac{3kq}{d} = \frac{-2kq}{d}$
- (a)(iii) Change in potential energy
The change in potential energy $\Delta U$ when moving a charge $q'$ from A to B is given by $\Delta U = q'(V_B - V_A)$. In our case, $q' = +2q$.
$\Delta U = (2q) (V_B - V_A) = (2q) \left( \frac{-2kq}{d} - \frac{-\sqrt{2}kq}{d} \right) = (2q) \left( \frac{-2kq}{d} + \frac{\sqrt{2}kq}{d} \right) = \frac{2kq^2}{d} (-2 + \sqrt{2}) = \frac{(-4 + 2\sqrt{2})kq^2}{d}$
- (b) Location of zero electric potential
Let $x$ be the coordinate where the potential is zero. The distance from $Q_1$ to this point is $x$, and the distance from $Q_2$ to this point is $2d - x$. We want to find $x$ such that $V = 0$.
$V = \frac{kQ_1}{x} + \frac{kQ_2}{2d-x} = 0$ $\frac{kq}{x} + \frac{k(-3q)}{2d-x} = 0$ $\frac{1}{x} - \frac{3}{2d-x} = 0$ $\frac{1}{x} = \frac{3}{2d-x}$ $2d - x = 3x$ $2d = 4x$ $x = \frac{2d}{4} = \frac{d}{2}$
(a) (i) $V_A = \frac{-\sqrt{2}kq}{d}$ (ii) $V_B = \frac{-2kq}{d}$ (iii) $\Delta U = \frac{(-4 + 2\sqrt{2})kq^2}{d}$
(b) $x = \frac{d}{2}$
More Information
The electric potential is a scalar quantity, meaning that the total potential at a point due to multiple charges is simply the algebraic sum of the potentials due to each individual charge.
Tips
- Forgetting to include the sign of the charges when calculating the electric potential.
- Incorrectly calculating the distances between the charges and the points where the potential is being evaluated.
- Making algebraic errors when solving for the x-coordinate where the electric potential is zero.
AI-generated content may contain errors. Please verify critical information