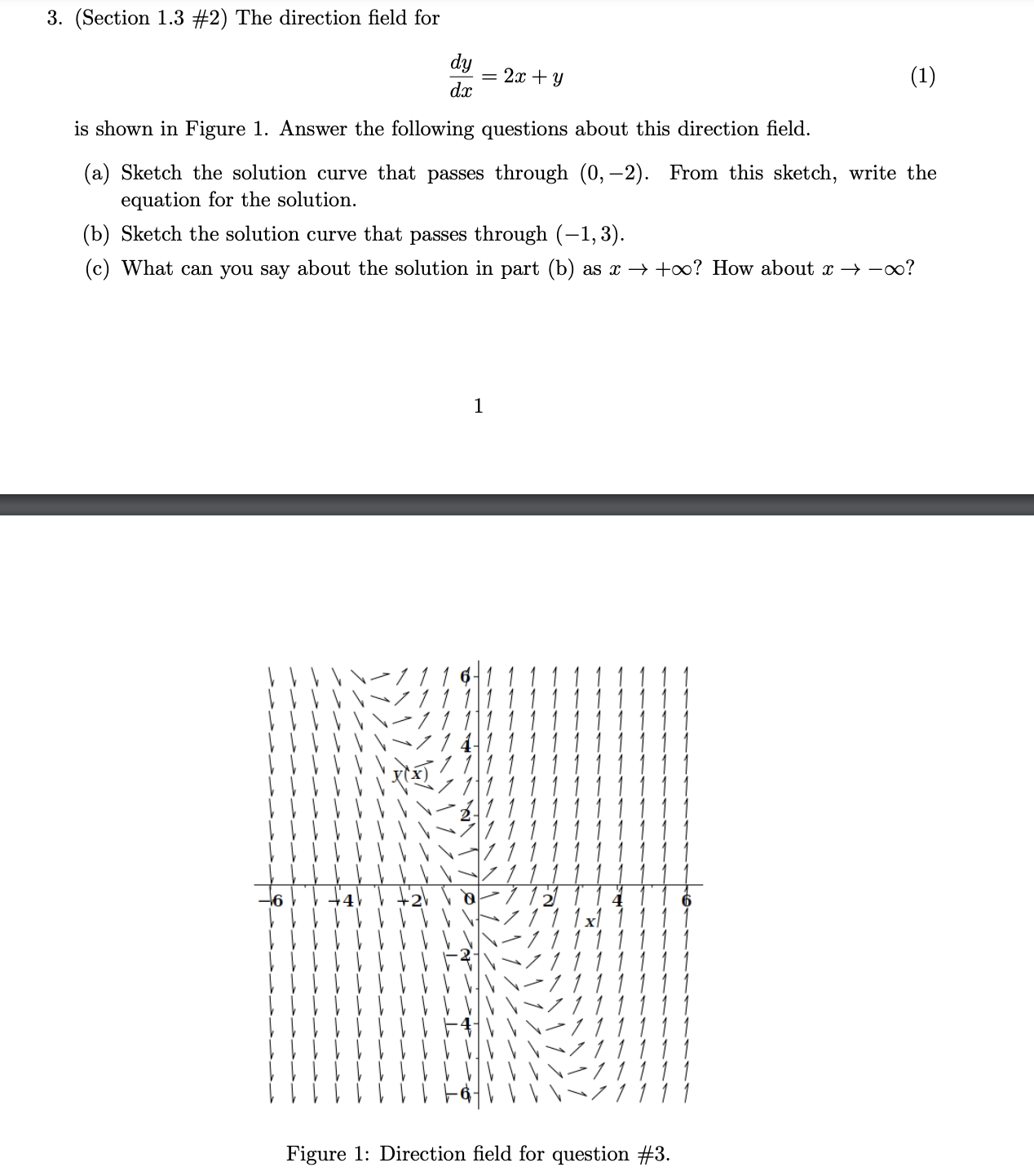
The direction field for dy/dx = 2x + y is shown in Figure 1. Answer the following questions about this direction field. (a) Sketch the solution curve that passes through (0, -2). F... The direction field for dy/dx = 2x + y is shown in Figure 1. Answer the following questions about this direction field. (a) Sketch the solution curve that passes through (0, -2). From this sketch, write the equation for the solution. (b) Sketch the solution curve that passes through (-1, 3). (c) What can you say about the solution in part (b) as x → +∞? How about x → -∞?
Understand the Problem
The question involves analyzing a direction field of a differential equation, which requires sketching solution curves based on given points and discussing long-term behavior. The objective is to understand the dynamics of the solutions represented in the field.
Answer
(a) $y = 2x - 2$; (b) $y = 7e^{x} - 2x - 2$; (c) As $x \to +\infty$, $y \to +\infty$; as $x \to -\infty$, $y \to -2$.
Answer for screen readers
(a) The specific solution curve through (0, -2) is
$$ y = 2x - 2 $$
(b) The specific solution curve through (-1, 3) is
$$ y = 7e^{x} - 2x - 2 $$
(c) As ( x \rightarrow +\infty ), ( y \rightarrow +\infty ) and as ( x \rightarrow -\infty ), ( y \rightarrow -2 ).
Steps to Solve
- Identify the Equation and Direction Field
The differential equation given is
$$ \frac{dy}{dx} = 2x + y $$
The direction field illustrates the slope defined by this equation at various points.
- Find the General Solution
To find the general solution, we can rewrite the equation in a standard linear form:
$$ \frac{dy}{dx} - y = 2x $$
This is a linear first-order differential equation.
- Solve the Homogeneous Equation
First, we solve the associated homogeneous equation:
$$ \frac{dy}{dx} - y = 0 $$
The solution is:
$$ y_h = Ce^x $$
where (C) is a constant.
- Find a Particular Solution
Next, we find a particular solution (y_p). We can use the method of undetermined coefficients. Assuming a solution of the form:
$$ y_p = Ax + B $$
Substituting (y_p) into the equation gives us:
$$ A = 2 \quad \text{and} \quad B = -2 $$
Thus, the particular solution is:
$$ y_p = 2x - 2 $$
- Combine Solutions
Now, we combine the homogeneous and particular solutions:
$$ y = Ce^x + 2x - 2 $$
- Sketch the Solutions
(a) Sketch the solution curve through (0, -2)
To find (C), substitute the point ((0, -2)):
$$ -2 = Ce^0 + 2(0) - 2 \rightarrow C = 0 $$
Thus, the specific solution is:
$$ y = 2x - 2 $$
(b) Sketch the solution curve through (-1, 3)
Substituting ((-1, 3)):
$$ 3 = Ce^{-1} + 2(-1) - 2 \rightarrow 3 = Ce^{-1} - 4 \rightarrow C = 7e $$
Thus, the specific solution is:
$$ y = 7e^{x} - 2x - 2 $$
(c) Discussing Long-term Behavior
As ( x \rightarrow +\infty ):
- The term ( 7e^{x} ) dominates, so ( y \rightarrow +\infty ).
As ( x \rightarrow -\infty ):
- The term ( 7e^{x} ) tends to zero, leading to ( y \rightarrow -2 ).
(a) The specific solution curve through (0, -2) is
$$ y = 2x - 2 $$
(b) The specific solution curve through (-1, 3) is
$$ y = 7e^{x} - 2x - 2 $$
(c) As ( x \rightarrow +\infty ), ( y \rightarrow +\infty ) and as ( x \rightarrow -\infty ), ( y \rightarrow -2 ).
More Information
The equation $\frac{dy}{dx} = 2x + y$ is a linear first-order differential equation. The direction field demonstrates the behavior of solutions with different initial conditions, highlighting their long-term trends.
Tips
- Forgetting to consider both the homogeneous and particular solutions.
- Not correctly applying initial conditions to find constants.
- Misinterpreting the direction field, leading to incorrect long-term behavior conclusions.
AI-generated content may contain errors. Please verify critical information