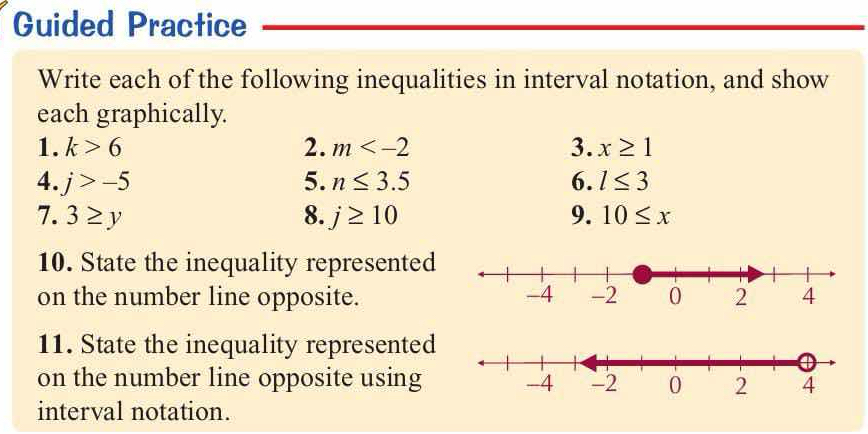
State the inequality represented on the number line opposite for questions 10 and 11.
Understand the Problem
The question is asking to identify and express the inequalities represented on two number lines and to write them in interval notation. Specifically, it requires recognizing the ranges indicated visually.
Answer
1. $k \leq -2$: $(-\infty, -2]$ 2. $j > 1$: $(1, 4)$
Answer for screen readers
- $k \leq -2$: Interval notation is $(-\infty, -2]$.
- $j > 1$: Interval notation is $(1, 4)$.
Steps to Solve
-
Identify the range for the first number line The first number line shows a closed dot at $-2$ and extends to the left towards $-\infty$. This indicates that the inequality includes $-2$ and all values less than it. Thus, the inequality can be expressed as: $$ k \leq -2 $$
-
State the interval notation for the first inequality The interval notation for the first inequality, which includes all values less than or equal to $-2$, is: $$ (-\infty, -2] $$
-
Identify the range for the second number line The second number line has an open dot at $1$ and extends to the right towards $4$. This means that $1$ is not included in the set of values while all values greater than $1$ up to $4$ are included. The corresponding inequality is: $$ j > 1 $$
-
State the interval notation for the second inequality The interval notation for the second inequality, which includes all values greater than $1$ up to $4$, is: $$ (1, 4) $$
- $k \leq -2$: Interval notation is $(-\infty, -2]$.
- $j > 1$: Interval notation is $(1, 4)$.
More Information
Interval notation is a way of representing a range of values along the number line. A closed dot indicates inclusion of the endpoint, while an open dot indicates exclusion.
Tips
- Misinterpreting closed or open dots; remember, closed dots mean "includes" the number, while open dots mean "excludes" it.
- Not considering the direction of arrows; arrows indicate whether the range goes to negative or positive infinity.
AI-generated content may contain errors. Please verify critical information