Solve the system of equations using elimination: -3x + 2y = 8 -6x + 7y = -8
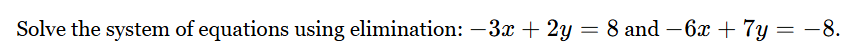
Understand the Problem
The question asks to solve a system of two linear equations for the variables x and y using the elimination method. The system consists of the equations -3x + 2y = 8 and -6x + 7y = -8.
Answer
$x = -8$ $y = -8$ $(-8, -8)$
Answer for screen readers
$x = -8$ $y = -8$ The solution to the system of equations is $(-8, -8)$.
Steps to Solve
- Multiply the first equation by -2 Multiply the first equation, $-3x + 2y = 8$, by $-2$ to make the coefficient of $x$ in the first equation equal to the coefficient of $x$ in the second equation. This gives us:
$(-2) \cdot (-3x) + (-2) \cdot (2y) = (-2) \cdot (8)$ $6x - 4y = -16$
-
Write the modified system of equations The original second equation is $-6x + 7y = -8$. The modified system is now: $6x - 4y = -16$ $-6x + 7y = -8$
-
Add the two equations to eliminate $x$ Add the two equations together. Notice that the $x$ terms will cancel out: $(6x - 4y) + (-6x + 7y) = -16 + (-8)$ $6x - 6x - 4y + 7y = -24$ $3y = -24$
-
Solve for $y$ Divide both sides of the equation $3y = -24$ by $3$ to solve for $y$: $y = \frac{-24}{3}$ $y = -8$
-
Substitute the value of $y$ into one of the original equations Substitute $y = -8$ into the first original equation, $-3x + 2y = 8$: $-3x + 2(-8) = 8$ $-3x - 16 = 8$
-
Solve for $x$ Add 16 to both sides of the equation: $-3x = 8 + 16$ $-3x = 24$ Divide both sides by -3: $x = \frac{24}{-3}$ $x = -8$
-
Write the solution as an ordered pair The solution is $x = -8$ and $y = -8$, which can be written as the ordered pair $(-8, -8)$.
$x = -8$ $y = -8$ The solution to the system of equations is $(-8, -8)$.
More Information
We can check the answer by substituting $x = -8$ and $y = -8$ into both original equations:
Equation 1: $-3x + 2y = 8$ $-3(-8) + 2(-8) = 24 - 16 = 8$. This is correct.
Equation 2: $-6x + 7y = -8$ $-6(-8) + 7(-8) = 48 - 56 = -8$. This is also correct.
Tips
A common mistake is to make an error when multiplying the equation by a constant. This means multiplying only one term and forgetting to multiply all the terms in the equation.
Another mistake is to make a sign error when adding or subtracting the equations. Misinterpreting negative signs is easy, so it is important to be careful.
AI-generated content may contain errors. Please verify critical information