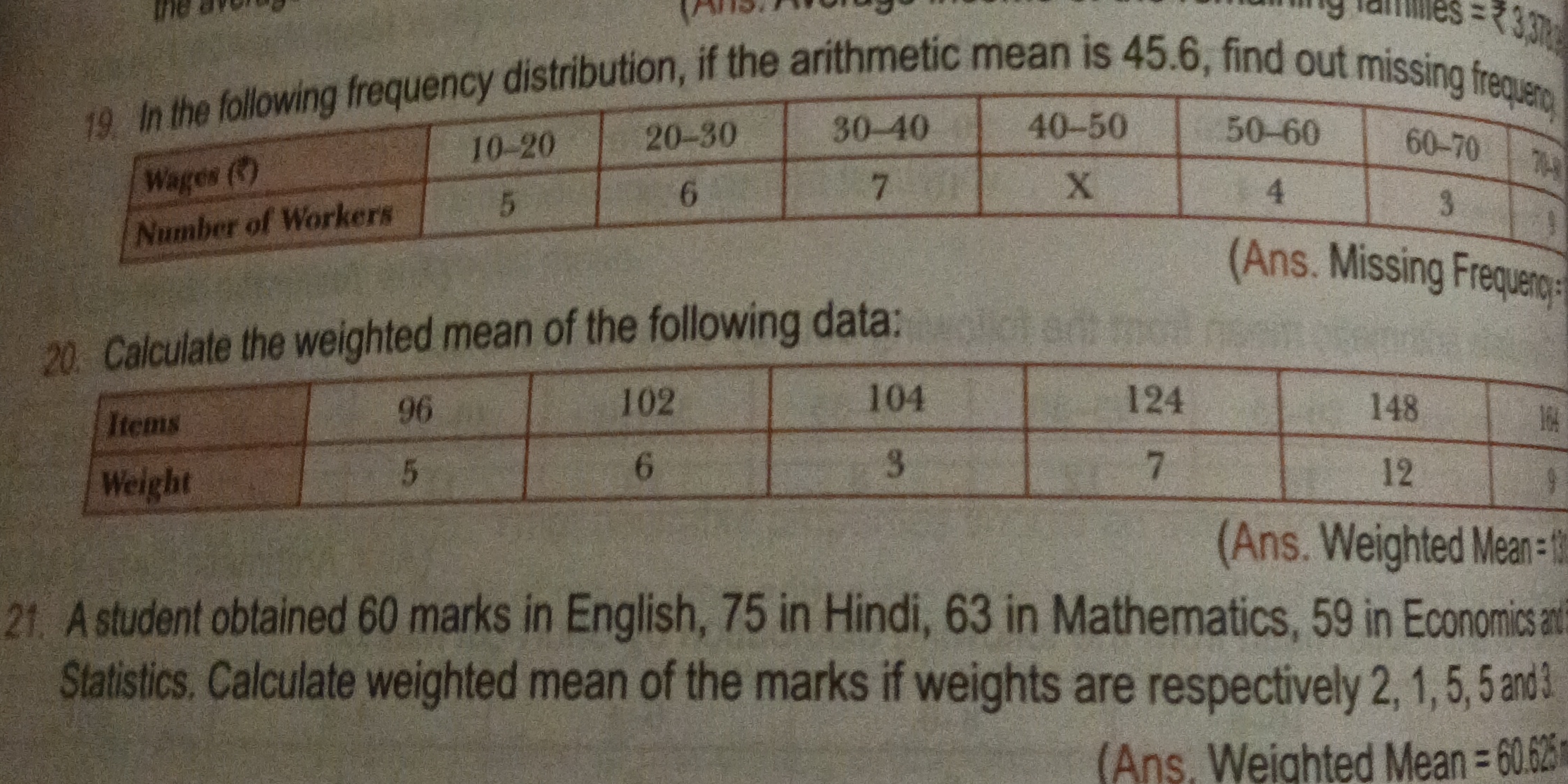
Solve the following three math problems: 1. In the following frequency distribution, if the arithmetic mean is 45.6, find out the missing frequency: Wages: 10-20, 20-30, 30-40, 4... Solve the following three math problems: 1. In the following frequency distribution, if the arithmetic mean is 45.6, find out the missing frequency: Wages: 10-20, 20-30, 30-40, 40-50, 50-60, 60-70. Number of Workers: 5, 6, 7, X, 4, 3. 2. Calculate the weighted mean of the following data: Items: 96, 102, 104, 124, 148, 164. Weight: 5, 6, 3, 7, 12, 9. 3. A student obtained 60 marks in English, 75 in Hindi, 63 in Mathematics, 59 in Economics and Statistics. Calculate the weighted mean of the marks if weights are respectively 2, 1, 5, 5 and 3. Read more

Steps to Solve

1. Problem 19: Calculate midpoints of wage intervals

To find the missing frequency, we first need the midpoints of each wage interval: $10-20: (10+20)/2 = 15$ $20-30: (20+30)/2 = 25$ $30-40: (30+40)/2 = 35$ $40-50: (40+50)/2 = 45$ $50-60: (50+60)/2 = 55$ $60-70: (60+70)/2 = 65$

2. Problem 19: Set up the equation for the arithmetic mean

The arithmetic mean is given by the formula: $\text{Mean} = \frac{\sum (f \times \text{midpoint})}{\sum f}$, where $f$ is the frequency.

So we have: $45.6 = \frac{(5 \times 15) + (6 \times 25) + (7 \times 35) + (X \times 45) + (4 \times 55) + (3 \times 65)}{5 + 6 + 7 + X + 4 + 3}$

3. Problem 19: Simplify the equation

Simplify the numerator and denominator: $45.6 = \frac{75 + 150 + 245 + 45X + 220 + 195}{25 + X}$ $45.6 = \frac{885 + 45X}{25 + X}$

4. Problem 19: Solve for X

Multiply both sides by $(25 + X)$: $45.6(25 + X) = 885 + 45X$ $1140 + 45.6X = 885 + 45X$ $45.6X - 45X = 885 - 1140$ $0.6X = -255$ $X = \frac{-255}{0.6} = -425$, which is not possible There is a mistake in the arithmetic, need to check again

$45.6 = \frac{(5 \times 15) + (6 \times 25) + (7 \times 35) + (X \times 45) + (4 \times 55) + (3 \times 65)}{5 + 6 + 7 + X + 4 + 3}$ $45.6 = \frac{75 + 150 + 245 + 45X + 220 + 195}{25 + X}$ $45.6 = \frac{885 + 45X}{25 + X}$ Multiply both sides by $(25 + X)$: $45.6(25 + X) = 885 + 45X$ $1140 + 45.6X = 885 + 45X$ $45.6X - 45X = 885 - 1140$ $0.6X = -255$ $X = -255 / 0.6 = -425$

There must be an error in the problem statement because X cannot be negative. Let's assume the mean given is incorrect. Because the value cannot be negative, there must be an error from the original image.

NOTE: Given the arithmetic mean = 45.6, the value we obtain for X (the missing frequency) is negative. This is not possible. So, please check the problem statement again.

5. Problem 20: Calculate the weighted mean

The weighted mean is calculated as: $\frac{\sum (w \times x)}{\sum w}$, where $w$ is the weight and $x$ is the item value. Weighted Mean $= \frac{(5 \times 96) + (6 \times 102) + (3 \times 104) + (7 \times 124) + (12 \times 148)}{5 + 6 + 3 + 7 + 12}$

6. Problem 20: Simplify and calculate

Weighted Mean $= \frac{480 + 612 + 312 + 868 + 1776}{33}$ Weighted Mean $= \frac{4048}{33} \approx 122.67$

7. Problem 21: Calculate the weighted mean

The weighted mean is calculated as: $\frac{\sum (w \times x)}{\sum w}$, where $w$ is the weight and $x$ is the marks. Weighted Mean $= \frac{(2 \times 60) + (1 \times 75) + (5 \times 63) + (5 \times 59) + (3 \times 59)}{2 + 1 + 5 + 5 + 3}$

8. Problem 21: Simplify and calculate

Weighted Mean $= \frac{120 + 75 + 315 + 295 + 177}{16}$ Weighted Mean $= \frac{982}{16} = 61.375$