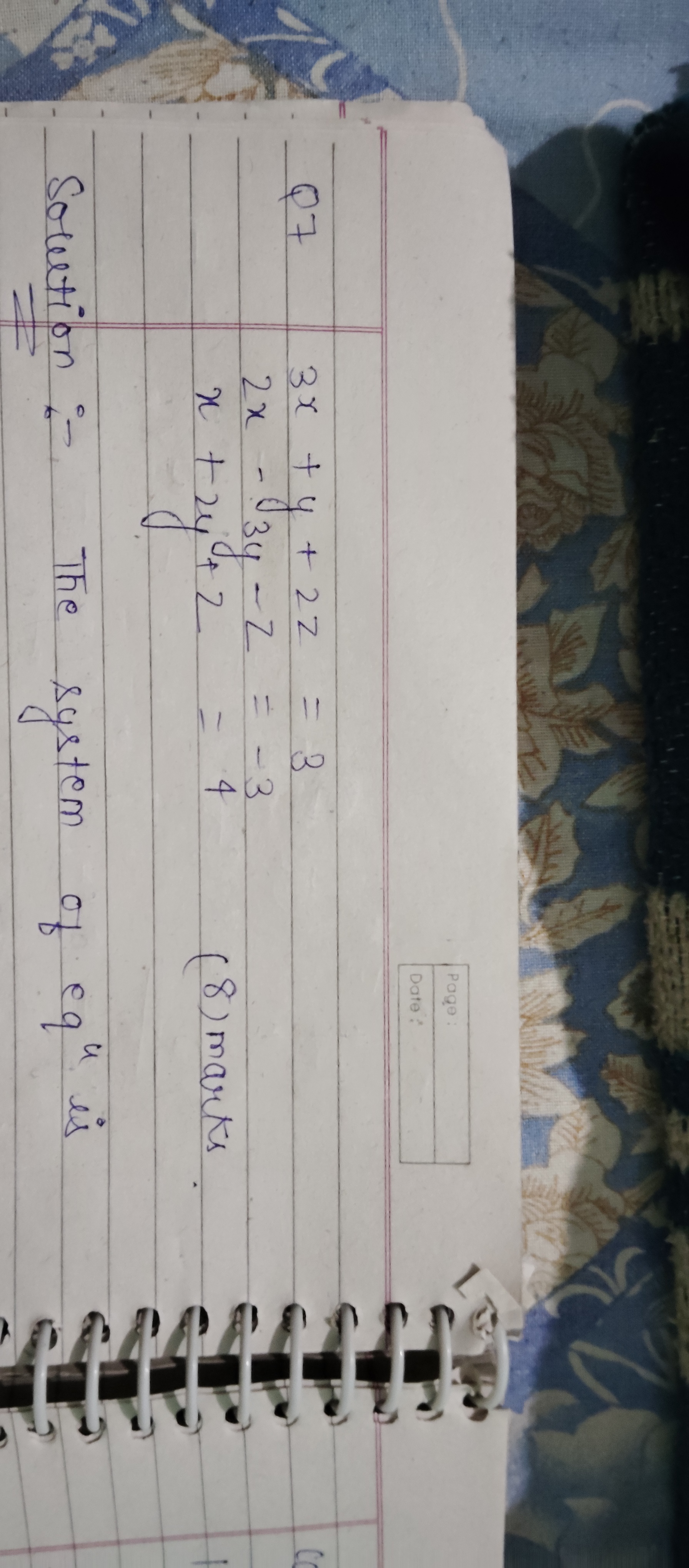
Solve the following system of equations: 3x + y + 2z = 8, 2x - 3y - z = -3, x + 2y - z = 4.

Steps to Solve

1. Write down the system of equations We are given three equations: [ 3x + y + 2z = 8 \tag{1} ] [ 2x - 3y - z = -3 \tag{2} ] [ x + 2y - z = 4 \tag{3} ]

2. Isolate one variable Start with equation (3) to express $z$ in terms of $x$ and $y$: [ z = x + 2y - 4 \tag{4} ]

3. Substitute for z Substitute equation (4) into equations (1) and (2): For equation (1): [ 3x + y + 2(x + 2y - 4) = 8 ] This simplifies to: [ 3x + y + 2x + 4y - 8 = 8 \Rightarrow 5x + 5y = 16 \Rightarrow x + y = \frac{16}{5} \tag{5} ]

For equation (2): [ 2x - 3y - (x + 2y - 4) = -3 ] This simplifies to: [ 2x - 3y - x - 2y + 4 = -3 \Rightarrow x - 5y + 4 = -3 \Rightarrow x - 5y = -7 \tag{6} ]

4. Solve the new system of equations Now we have: [ x + y = \frac{16}{5} \tag{5} ] [ x - 5y = -7 \tag{6} ]

We can solve this system. From equation (5): [ x = \frac{16}{5} - y ] Substituting into equation (6): [ \frac{16}{5} - y - 5y = -7 ] Combining like terms gives: [ \frac{16}{5} - 6y = -7 ] To eliminate the fraction, multiply through by 5: [ 16 - 30y = -35 ] Thus, [ 30y = 51 \Rightarrow y = \frac{51}{30} = \frac{17}{10} ]

5. Find x and z using y Substituting $y = \frac{17}{10}$ back into equation (5): [ x + \frac{17}{10} = \frac{16}{5} \Rightarrow x = \frac{16}{5} - \frac{17}{10} ] Finding a common denominator (10): [ x = \frac{32}{10} - \frac{17}{10} = \frac{15}{10} = \frac{3}{2} ]

Now substitute $x$ and $y$ back into equation (4) to find $z$: [ z = \frac{3}{2} + 2 \cdot \frac{17}{10} - 4 ] Calculating: [ z = \frac{3}{2} + \frac{34}{10} - 4 = \frac{3}{2} + \frac{17}{5} - 4 ] Convert to the same denominator: [ z = \frac{15}{10} + \frac{34}{10} - \frac{40}{10} = \frac{9}{10} ]

6. Final values The solutions are: [ x = \frac{3}{2}, \quad y = \frac{17}{10}, \quad z = \frac{9}{10} ]