Solve the equation x^2 + 10x + 21 = 0
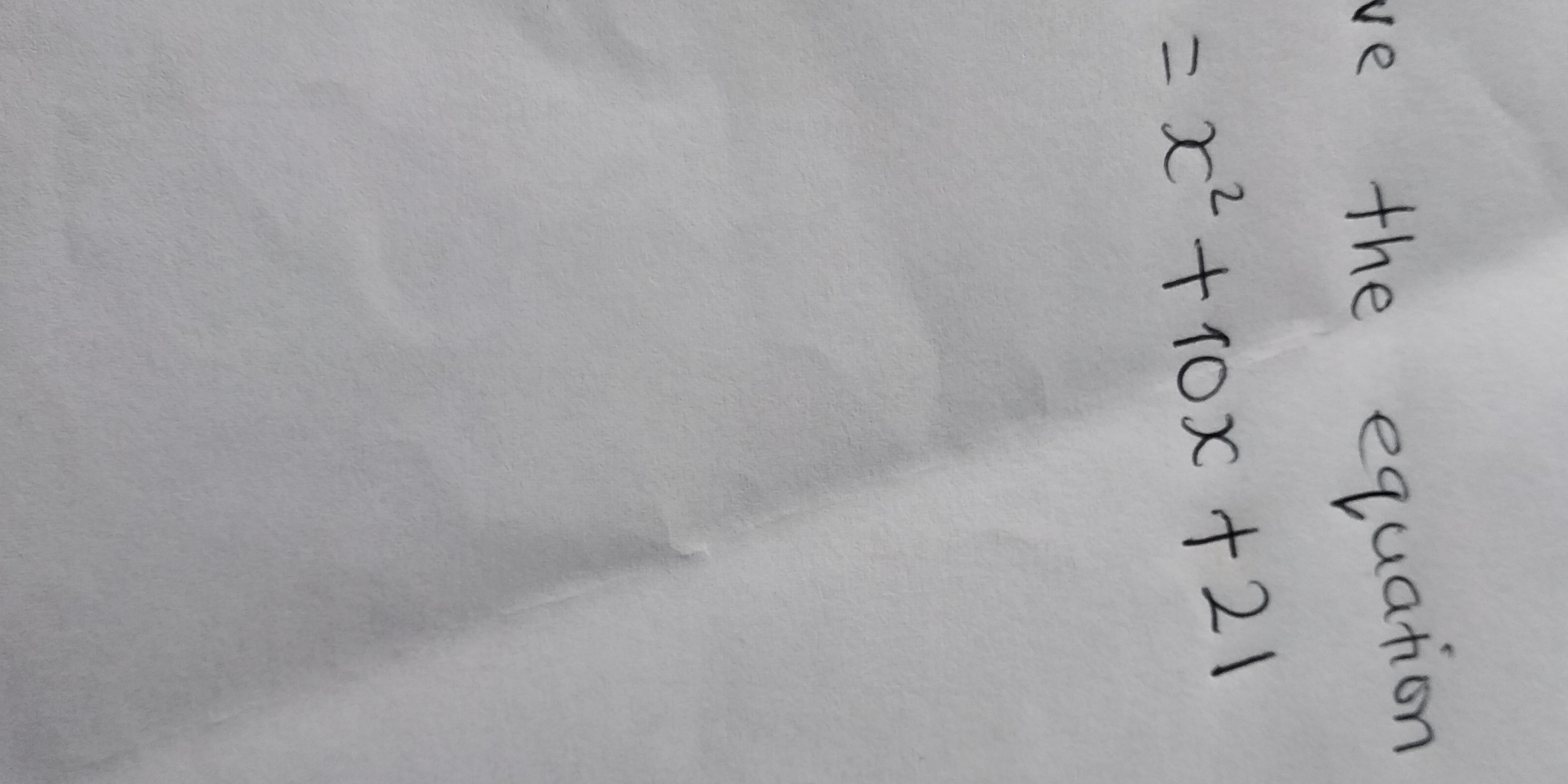
Understand the Problem
The question is asking to solve the quadratic equation x^2 + 10x + 21 = 0. This involves finding the values of x that satisfy the equation. We can solve this by factoring, completing the square, or using the quadratic formula.
Answer
$x = -3, -7$
Answer for screen readers
$x = -3, -7$
Steps to Solve
-
Factor the quadratic equation We need to find two numbers that multiply to 21 and add up to 10. These numbers are 3 and 7. $x^2 + 10x + 21 = (x+3)(x+7) = 0$
-
Set each factor equal to zero This gives us two separate equations to solve: $x + 3 = 0$ and $x + 7 = 0$
-
Solve for x in each equation Solving $x + 3 = 0$, we subtract 3 from both sides: $x = -3$ Solving $x + 7 = 0$, we subtract 7 from both sides: $x = -7$
$x = -3, -7$
More Information
The solutions to the quadratic equation $x^2 + 10x + 21 = 0$ are $x = -3$ and $x = -7$. These are the x-intercepts of the parabola represented by the equation.
Tips
A common mistake is to incorrectly factor the quadratic equation. Always double-check that the factors multiply back to the original equation. Also, forgetting to set each factor to zero and solve for $x$ is a frequent error.
AI-generated content may contain errors. Please verify critical information