Solve for x: 4 = 8(7x + 5)
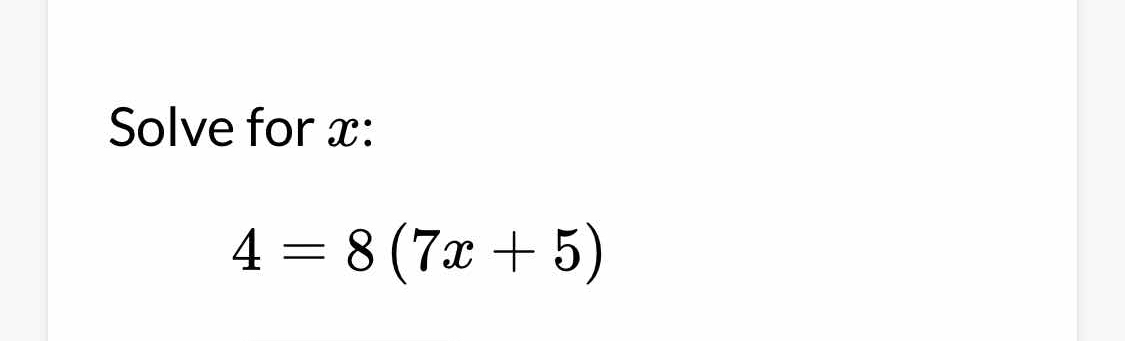
Understand the Problem
The question asks us to solve the equation for the variable 'x'. We will need to use the distributive property and isolate 'x' using algebraic manipulations.
Answer
$x = -\frac{9}{14}$
Answer for screen readers
$x = -\frac{9}{14}$
Steps to Solve
- Distribute the 8
Multiply 8 by both terms inside the parentheses.
$4 = 8(7x) + 8(5)$
$4 = 56x + 40$
- Isolate the term with x
Subtract 40 from both sides of the equation to isolate the term with $x$.
$4 - 40 = 56x + 40 - 40$
$-36 = 56x$
- Solve for x
Divide both sides by 56 to solve for $x$.
$\frac{-36}{56} = \frac{56x}{56}$
$x = \frac{-36}{56}$
- Simplify the fraction
Simplify the fraction by dividing both the numerator and the denominator by their greatest common divisor, which is 4.
$x = \frac{-36 \div 4}{56 \div 4}$
$x = \frac{-9}{14}$
$x = -\frac{9}{14}$
More Information
The solution to the equation $4 = 8(7x + 5)$ is $x = -\frac{9}{14}$. This value of $x$ makes the equation true.
Tips
A common mistake is not distributing the 8 correctly to both terms inside the parenthesis. Remember to multiply the 8 by both the $7x$ and the 5. Another common mistake is making an arithmetic error when subtracting or dividing.
AI-generated content may contain errors. Please verify critical information