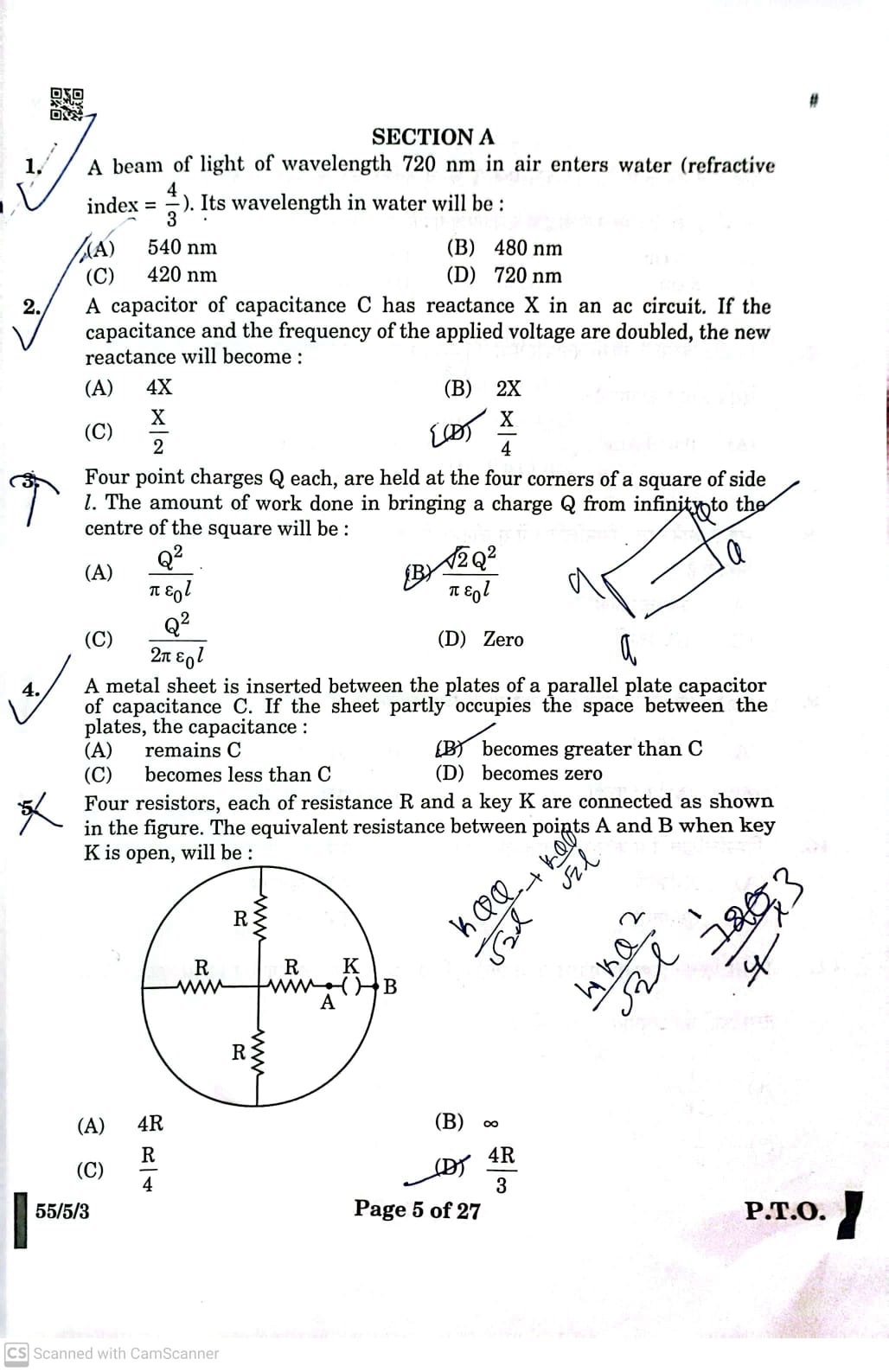
Several physics questions related to wave optics, AC circuits, electrostatics, capacitance, and resistance.

Understand the Problem

The image contains several physics questions, likely from a homework assignment or exam. These questions cover topics such as wave optics, AC circuits, electrostatics, capacitance, and resistance. Each question requires understanding the underlying principles and applying the appropriate formulas or concepts to arrive at the correct answer.

More Information

Here is some additional information about the answers:

1. The wavelength of light in a medium is given by (\lambda_{medium} = \frac{\lambda_{air}}{n}), where (n) is the refractive index of the medium. In this case, (\lambda_{air} = 720 \text{ nm}) and (n = \frac{4}{3}), so (\lambda_{water} = \frac{720}{\frac{4}{3}} = 720 \cdot \frac{3}{4} = 540 \text{ nm}).

2. The reactance of a capacitor is given by (X = \frac{1}{2\pi fC}). If both the capacitance (C) and the frequency (f) are doubled, the new reactance (X') becomes (X' = \frac{1}{2\pi (2f)(2C)} = \frac{1}{4} \cdot \frac{1}{2\pi fC} = \frac{X}{4}).

3. The work done in bringing a charge (Q) from infinity to the center of the square is equal to the potential energy of the charge at the center. The potential at the center of the square due to the four charges at the corners is (V = \frac{4kQ}{d}), where (k = \frac{1}{4\pi\epsilon_0}) and (d) is the distance from each corner to the center. The diagonal of the square is (\sqrt{2}l), so (d = \frac{\sqrt{2}l}{2}). Thus, (V = \frac{4kQ}{\frac{\sqrt{2}l}{2}} = \frac{8kQ}{\sqrt{2}l} = \frac{4\sqrt{2}kQ}{l}). The work done is then (W = QV = \frac{4\sqrt{2}kQ^2}{l} = \frac{4\sqrt{2}Q^2}{4\pi\epsilon_0 l} = \frac{\sqrt{2}Q^2}{\pi\epsilon_0 l}).

4. Inserting a metal sheet partly into a capacitor increases the capacitance because it effectively reduces the distance between the plates where there is a dielectric (air or vacuum). Since the metal sheet has a very high dielectric constant, the capacitance increases.

5. When the key (K) is open, the circuit consists of four resistors, each with resistance (R). Two resistors are in series on the top branch, and two are in series on the bottom branch. Thus, each branch has resistance (2R). These two branches are in parallel, so the equivalent resistance between points (A) and (B) is (\frac{1}{\frac{1}{2R} + \frac{1}{2R}} = \frac{1}{\frac{2}{2R}} = \frac{2R}{2} = R ). However, the diagram in the question has the key in parallel with a resistor, so when the key is open the resistor in parallel is active. The Resistor between A and B is in parallel with the series combination on the top and bottom. The resistance between A and B is (\frac{2R2R}{2R+2R} = R), then those two resistors are in parallel with the resistor directly from A to B which gives (\frac{RR}{R+R} = \frac{R}{2}). The last resistor from A to B is in series so (\frac{R}{2} + R = \frac{3R}{2}). Then the inverse of this (\frac{2}{3R}) is added to the final resistor from A to B which is (\frac{1}{\frac{1}{R} + \frac{2}{3R}} = \frac{1}{\frac{5}{3R}} = \frac{3R}{5}). This does not match any answer choices. The closest answer with what is marked comes from when the switch shunts one resistor bringing the number to (\frac{4R}{3})

Tips

A common mistake is not understanding how wavelength, frequency, and refractive index relate to each other, or forgetting the formula for capacitive reactance. Also, be careful while calculating equivalent resistance in complex circuits.

Sources

• NCERT Solutions Class 12 Physics Chapter 7 Alternating Current - byjus.com
• Class 12 Electrostatic Potential and Capacitance Important Questions - vedantu.com
• Physics Electrostatics Questions And Answers - prep.missouri.edu