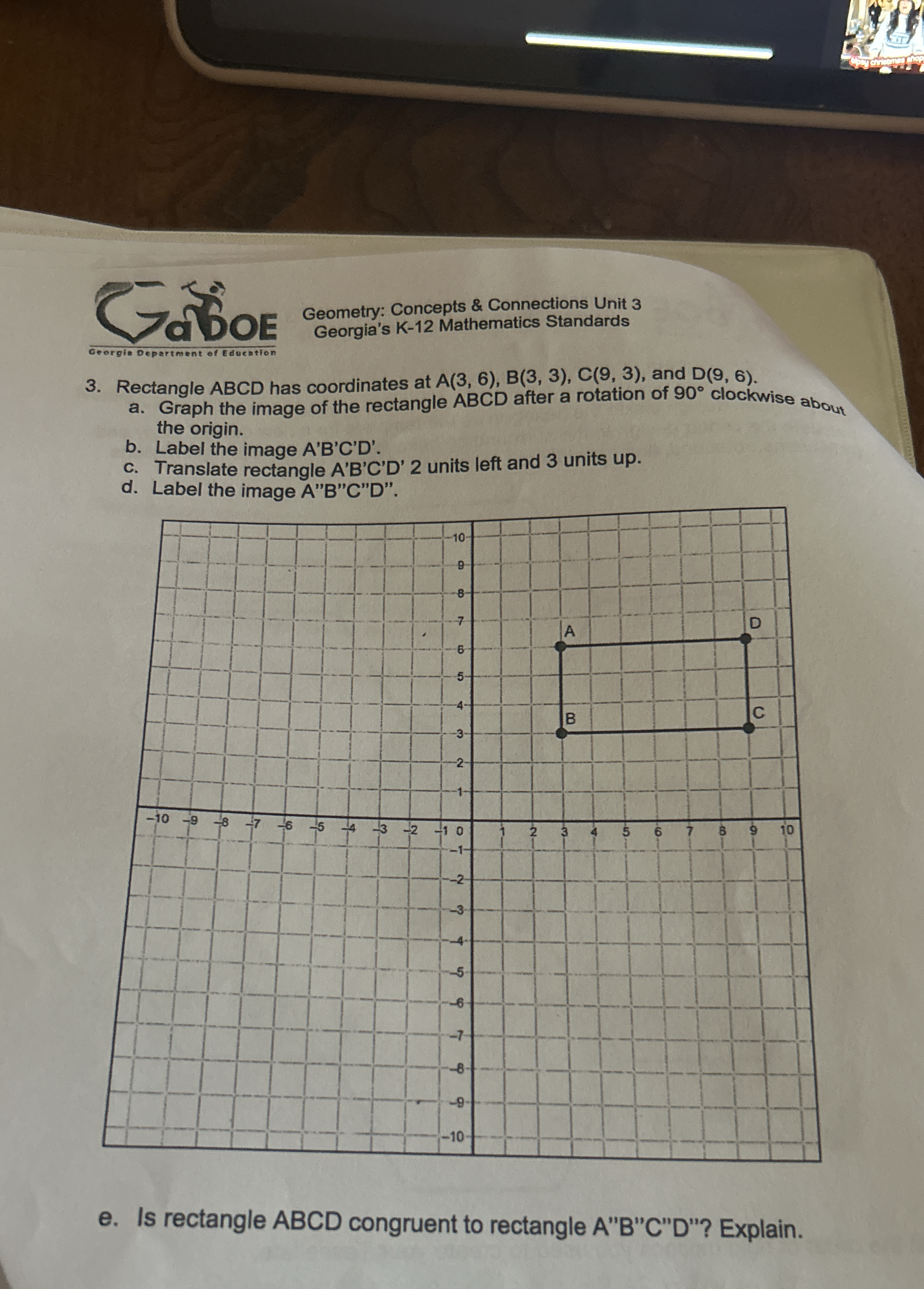
Rectangle ABCD has coordinates at A(3, 6), B(3, 3), C(9, 3), and D(9, 6). a. Graph the image of the rectangle ABCD after a rotation of 90° clockwise about the origin. b. Label the... Rectangle ABCD has coordinates at A(3, 6), B(3, 3), C(9, 3), and D(9, 6). a. Graph the image of the rectangle ABCD after a rotation of 90° clockwise about the origin. b. Label the image A'B'C'D'. c. Translate rectangle A'B'C'D' 2 units left and 3 units up. d. Label the image A''B''C''D''. e. Is rectangle ABCD congruent to rectangle A''B''C''D''? Explain.
Understand the Problem
The question is asking to perform several geometric transformations involving a rectangle defined by specific coordinates. It involves graphing, labeling, translating, and analyzing congruence of the rectangles based on transformations.
Answer
Yes, rectangle ABCD is congruent to rectangle A''B''C''D''.
Answer for screen readers
Yes, rectangle ABCD is congruent to rectangle A''B''C''D''.
Steps to Solve
- Graph the original rectangle ABCD
Start by plotting the coordinates of rectangle ABCD:
- A(3, 6)
- B(3, 3)
- C(9, 3)
- D(9, 6)
Connect the points in order to form the rectangle.
- Perform the rotation of 90° clockwise
To rotate a point $(x, y)$ by 90° clockwise about the origin, use the transformation:
$$(x, y) \rightarrow (y, -x)$$
Applying this to each vertex:
- A(3, 6) → A'(6, -3)
- B(3, 3) → B'(3, -3)
- C(9, 3) → C'(3, -9)
- D(9, 6) → D'(6, -9)
- Label the image A'B'C'D'
Plot the new points A', B', C', and D' on the graph based on the rotated coordinates:
- A'(6, -3)
- B'(3, -3)
- C'(3, -9)
- D'(6, -9)
- Translate rectangle A'B'C'D'
Translate the rectangle A'B'C'D' by 2 units left and 3 units up. To do this, for each point (x, y):
- New x-coordinate: $x - 2$
- New y-coordinate: $y + 3$
Calculating the new coordinates:
- A' → A''(6-2, -3+3) = A''(4, 0)
- B' → B''(3-2, -3+3) = B''(1, 0)
- C' → C''(3-2, -9+3) = C''(1, -6)
- D' → D''(6-2, -9+3) = D''(4, -6)
- Label the image A''B''C''D''
Plot the new points A'', B'', C'', and D'' on the graph:
- A''(4, 0)
- B''(1, 0)
- C''(1, -6)
- D''(4, -6)
- Check the congruence of rectangles
To check if rectangle ABCD is congruent to rectangle A''B''C''D'', compare the lengths of the sides.
- Length of sides in ABCD: 3 (AB or CD) and 6 (AD or BC).
- Length of sides in A''B''C''D'': 3 (B''C'' or A''D'') and 6 (A''B'' or C''D'').
Since the lengths are the same, the two rectangles are congruent.
Yes, rectangle ABCD is congruent to rectangle A''B''C''D''.
More Information
Congruence means that one shape can be transformed into another without changing its size or shape. Both rectangles have sides of the same lengths and maintain their right angles.
Tips
- Not applying the correct transformation for 90° rotation.
- Forgetting to apply both parts of the translation to each point.
- Confusing congruence with similarity; congruence requires equal side lengths and angles.
AI-generated content may contain errors. Please verify critical information