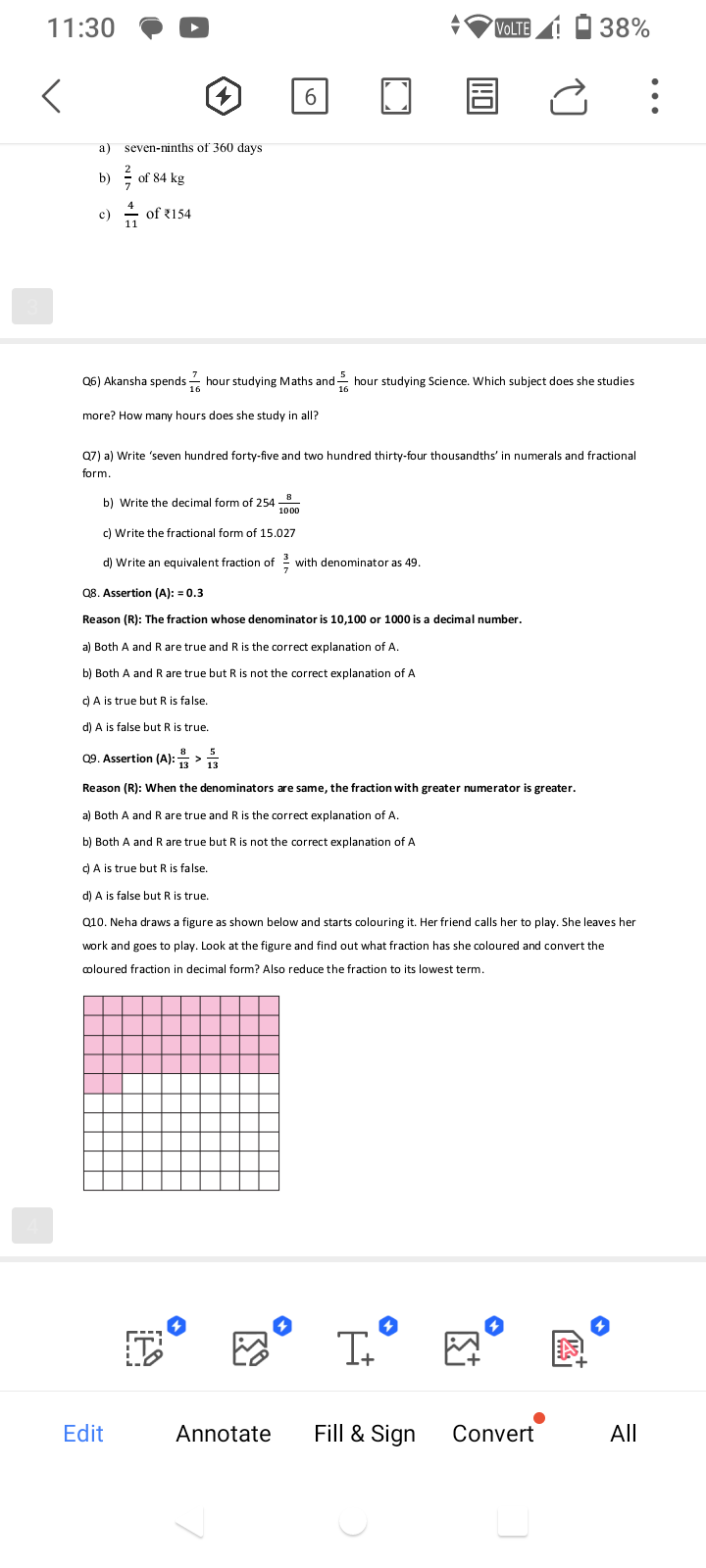
Q6) Akansha spends 7/16 hour studying Maths and 5/16 hour studying Science. Which subject does she studies more? How many hours does she study in all? Q7) a) Write ‘seven hundred f... Q6) Akansha spends 7/16 hour studying Maths and 5/16 hour studying Science. Which subject does she studies more? How many hours does she study in all? Q7) a) Write ‘seven hundred forty-five and two hundred thirty-four thousandths’ in numerals and fractional form. b) Write the decimal form of 254/1000. c) Write the fractional form of 15.027. d) Write an equivalent fraction of 3/7 with denominator 49. Q8) Assertion (A): 0.3. Reason (R): The fraction whose denominator is 10,100 or 1000 is a decimal number. a) Both A and R are true and R is the correct explanation of A. b) Both A and R are true but R is not the correct explanation of A. c) A is true but R is false. d) A is false but R is true. Q9) Assertion (A): 8/13 > 5/13. Reason (R): When the denominators are the same, the fraction with greater numerator is greater. a) Both A and R are true and R is the correct explanation of A. b) Both A and R are true but R is not the correct explanation of A. c) A is true but R is false. d) A is false but R is true. Q10) Neha draws a figure as shown below and starts colouring it. Her friend calls her to play. She leaves her work and goes to play. Look at the figure and find out what fraction has she coloured and convert the coloured fraction in decimal form? Also, reduce the fraction to its lowest term. Read more

Steps to Solve

1. Calculating Seven-Ninths of 360 Days

To find seven-ninths of 360 days, calculate: $$ \frac{7}{9} \times 360 = \frac{7 \times 360}{9} $$ Divide 360 by 9: $$ 360 \div 9 = 40 $$ Then multiply by 7: $$ 40 \times 7 = 280 $$ So, seven-ninths of 360 days is 280 days.

2. Calculating Two-Sevenths of 84 kg

For two-sevenths of 84 kg: $$ \frac{2}{7} \times 84 = \frac{2 \times 84}{7} $$ Divide 84 by 7: $$ 84 \div 7 = 12 $$ Then multiply by 2: $$ 12 \times 2 = 24 $$ So, two-sevenths of 84 kg is 24 kg.

3. Calculating Four Elevenths of ₹154

For four-elevenths of ₹154: $$ \frac{4}{11} \times 154 = \frac{4 \times 154}{11} $$ Divide 154 by 11: $$ 154 \div 11 = 14 $$ Then multiply by 4: $$ 14 \times 4 = 56 $$ So, four-elevenths of ₹154 is ₹56.

4. Calculating Total Study Hours for Akansha

Akansha studies: Maths: $$\frac{7}{16}$$ hour
Science: $$\frac{5}{16}$$ hour

To find out which is more, compare: $$ \frac{7}{16} \text{ (Maths)} > \frac{5}{16} \text{ (Science)} $$ So she studies more Maths.

Calculating total hours: $$ \frac{7}{16} + \frac{5}{16} = \frac{12}{16} = \frac{3}{4} \text{ hour} $$

5. Writing 745,234 in Numerals and Fractional Form

In numerals, it is 745,234.

In fractional form, it is: $$ 745234 = \frac{745234}{1} $$

6. Writing Decimal Form of 254 8/1000

Convert: $$ 254 + \frac{8}{1000} = 254.008 $$

7. Writing Fractional Form of 15.027

Convert: $$ 15.027 = \frac{15027}{1000} $$

8. Equivalent Fraction of 3/7 with Denominator 49

To find an equivalent fraction, set up: $$ \frac{3}{7} = \frac{x}{49} $$ Cross multiply to solve for x: $$ 3 \times 49 = 7 \times x \Rightarrow x = \frac{147}{7} = 21 $$ So, the equivalent fraction is $\frac{21}{49}$.