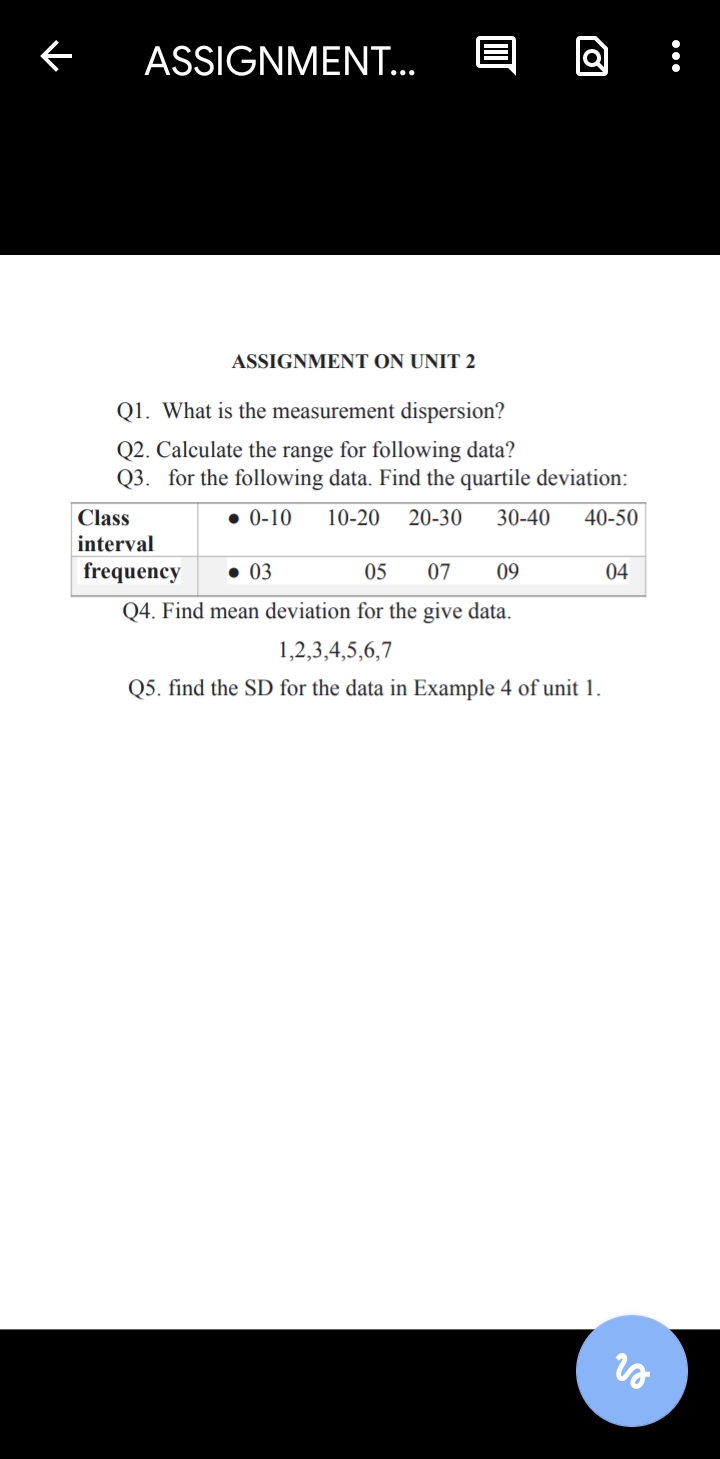
Q1. What is the measurement dispersion? Q2. Calculate the range for following data? Q3. For the following data, find the quartile deviation: Class interval frequency 0-10: 03, 10-2... Q1. What is the measurement dispersion? Q2. Calculate the range for following data? Q3. For the following data, find the quartile deviation: Class interval frequency 0-10: 03, 10-20: 05, 20-30: 07, 30-40: 09, 40-50: 04. Q4. Find mean deviation for the given data: 1,2,3,4,5,6,7. Q5. Find the SD for the data in Example 4 of unit 1. Read more

Steps to Solve

1. Calculate the Range

The range can be calculated by subtracting the smallest value from the largest value.

• The highest class interval is 40-50, with the upper limit of 50.
• The lowest class interval is 0-10, with the lower limit of 0.

So, the range is:

$$ \text{Range} = \text{Max} - \text{Min} = 50 - 0 = 50 $$

2. Find the Quartile Deviation

First, calculate the cumulative frequency and identify the quartiles.

• The frequencies are: 3, 5, 7, 9, 4.
• The total frequency (N):

$$ N = 3 + 5 + 7 + 9 + 4 = 28 $$

Now find (Q_1) (1st quartile) and (Q_3) (3rd quartile):

• (Q_1) is at position ( \frac{N}{4} = \frac{28}{4} = 7 )
• (Q_3) is at position ( \frac{3N}{4} = \frac{3 \times 28}{4} = 21 )

Now find these quartiles using the cumulative frequency table.

3. Cumulative Frequency Calculation

• For class interval 0-10: Cumulative frequency = 3
• For class interval 10-20: Cumulative frequency = 3 + 5 = 8
• For class interval 20-30: Cumulative frequency = 8 + 7 = 15
• For class interval 30-40: Cumulative frequency = 15 + 9 = 24
• For class interval 40-50: Cumulative frequency = 24 + 4 = 28

Now (Q_1) lies in the interval 10-20 and (Q_3) lies in the interval 30-40.

4. Calculate the Quartiles Using the Formula

The formula to find the quartile:

$$ Q_k = L_k + \left( \frac{kN/4 - CF}{f} \right) \times h $$

Where:

• (L_k) = Lower boundary of the class containing the quartile
• (CF) = Cumulative frequency of the class before the quartile class
• (f) = Frequency of the quartile class
• (h) = Class width

For (Q_1):

• Class interval = 10-20, (L_1 = 10), (CF = 3), (f = 5), (h = 10)

$$ Q_1 = 10 + \left( \frac{7 - 3}{5} \right) \times 10 = 10 + \left( \frac{4}{5} \right) \times 10 = 10 + 8 = 18 $$

For (Q_3):

• Class interval = 30-40, (L_3 = 30), (CF = 15), (f = 9)

$$ Q_3 = 30 + \left( \frac{21 - 15}{9} \right) \times 10 = 30 + \left( \frac{6}{9} \right) \times 10 = 30 + \frac{60}{9} \approx 36.67 $$

5. Calculate the Quartile Deviation

Quartile deviation is calculated as:

$$ QD = \frac{Q_3 - Q_1}{2} = \frac{36.67 - 18}{2} = \frac{18.67}{2} \approx 9.34 $$

6. Calculate the Mean Deviation

First, calculate the mean of the given data:

$$ \text{Mean} = \frac{\sum{(f \times x)}}{N} $$

Where (x) is the midpoint of each class interval.

• Midpoints: 5, 15, 25, 35, 45
• Calculate (f \times x):
  • For 0-10: (3 \times 5 = 15)
  • For 10-20: (5 \times 15 = 75)
  • For 20-30: (7 \times 25 = 175)
  • For 30-40: (9 \times 35 = 315)
  • For 40-50: (4 \times 45 = 180)

Total:

$$ \sum{(f \times x)} = 15 + 75 + 175 + 315 + 180 = 760 $$

Now calculate mean:

$$ \text{Mean} = \frac{760}{28} \approx 27.14 $$

Now, mean deviation is:

$$ MD = \frac{\sum{|x - \text{Mean}| \cdot f}}{N} $$

Calculate ( |x - \text{Mean}| ):

For each class interval, sum and substitute to find mean deviation.

7. Calculate Standard Deviation

Using the formula for standard deviation:

$$ SD = \sqrt{\frac{\sum{(f \times (x - \text{Mean})^2)}}{N}} $$

Calculate the squared differences from the mean, multiply by the frequencies, sum them, and divide by (N).