Find the quotient of (4p^6 - 20p^4 + 16p) / (12p).
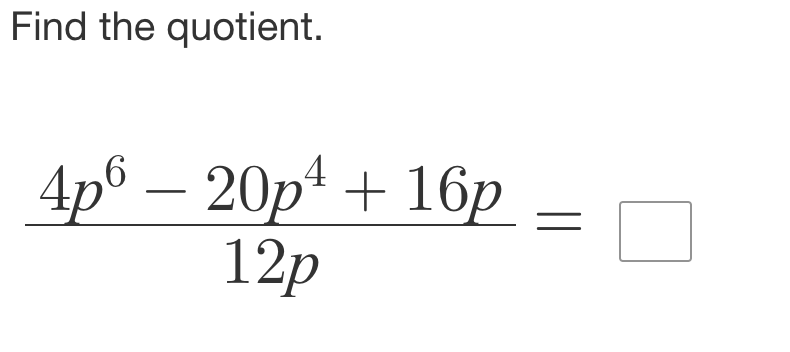
Understand the Problem
The question asks us to find the quotient of the given polynomial expression. We need to divide each term in the numerator by the term in the denominator and simplify.
Answer
$ \frac{1}{3}p^{5} - \frac{5}{3}p^{3} + \frac{4}{3} $
Answer for screen readers
$$ \frac{1}{3}p^{5} - \frac{5}{3}p^{3} + \frac{4}{3} $$
Steps to Solve
-
Rewrite the expression as a sum of fractions We divide each term in the numerator by the denominator: $$ \frac{4p^{6} - 20p^{4} + 16p}{12p} = \frac{4p^{6}}{12p} - \frac{20p^{4}}{12p} + \frac{16p}{12p} $$
-
Simplify each fraction Simplify each term by dividing the coefficients and using the rule $ \frac{p^a}{p^b} = p^{a-b} $: $$ \frac{4p^{6}}{12p} = \frac{1}{3}p^{6-1} = \frac{1}{3}p^{5} $$ $$ \frac{20p^{4}}{12p} = \frac{5}{3}p^{4-1} = \frac{5}{3}p^{3} $$ $$ \frac{16p}{12p} = \frac{4}{3}p^{1-1} = \frac{4}{3} $$
-
Combine the simplified terms Combine the simplified terms to get the final expression: $$ \frac{1}{3}p^{5} - \frac{5}{3}p^{3} + \frac{4}{3} $$
$$ \frac{1}{3}p^{5} - \frac{5}{3}p^{3} + \frac{4}{3} $$
More Information
Polynomial long division is not required here.
Tips
A common mistake is to forget to divide every term in the numerator by the denominator. Also, errors can be made when simplifying the exponents. Remember to subtract the exponent in the denominator from the exponent in the numerator.
AI-generated content may contain errors. Please verify critical information