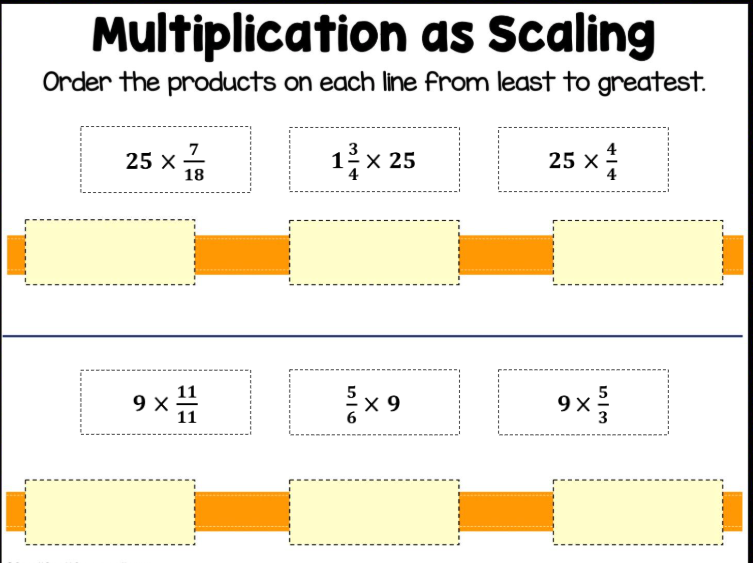
Order the products on each line from least to greatest. Line 1: 25 * (7/18), 1 3/4 * 25, and 25 * (4/4). Line 2: 9 * (11/11), (5/6) * 9, and 9 * (5/3).
Understand the Problem
The question requires ordering the products, following multiplication, from least to greatest on each line. Specifically, the first line involves ordering the results of: 25 * (7/18), 1 3/4 * 25, and 25 * (4/4). The second line involves ordering the results of 9 * (11/11), (5/6) * 9, and 9 * (5/3).
Answer
Line 1: $25 \times \frac{7}{18} < 25 \times \frac{4}{4} < 1\frac{3}{4} \times 25$ Line 2: $\frac{5}{6} \times 9 < 9 \times \frac{11}{11} < 9 \times \frac{5}{3}$
Answer for screen readers
Line 1: $25 \times \frac{7}{18} < 25 \times \frac{4}{4} < 1\frac{3}{4} \times 25$
Line 2: $\frac{5}{6} \times 9 < 9 \times \frac{11}{11} < 9 \times \frac{5}{3}$
Steps to Solve
- Calculate 25 * (7/18)
Multiply 25 by the fraction $\frac{7}{18}$:
$25 \times \frac{7}{18} = \frac{25 \times 7}{18} = \frac{175}{18} \approx 9.72$
- Calculate 1 3/4 * 25
Convert the mixed number $1\frac{3}{4}$ to an improper fraction: $1\frac{3}{4} = \frac{1 \times 4 + 3}{4} = \frac{7}{4}$.
Multiply the improper fraction by 25:
$\frac{7}{4} \times 25 = \frac{7 \times 25}{4} = \frac{175}{4} = 43.75$
- Calculate 25 * (4/4)
$\frac{4}{4}$ simplifies to 1. Multiply 25 by 1:
$25 \times 1 = 25$
-
Order the results of line 1 Order 9.72, 43.75, and 25 from least to greatest:
-
72 < 25 < 43.75
Therefore, $25 \times \frac{7}{18} < 25 \times \frac{4}{4} < 1\frac{3}{4} \times 25$
- Calculate 9 * (11/11)
$\frac{11}{11}$ simplifies to 1. Multiply 9 by 1:
$9 \times 1 = 9$
- Calculate (5/6) * 9
Multiply the fraction $\frac{5}{6}$ by 9:
$\frac{5}{6} \times 9 = \frac{5 \times 9}{6} = \frac{45}{6} = 7.5$
- Calculate 9 * (5/3)
Multiply 9 by the fraction $\frac{5}{3}$:
$9 \times \frac{5}{3} = \frac{9 \times 5}{3} = \frac{45}{3} = 15$
- Order the results of line 2
Order 9, 7.5, and 15 from least to greatest:
- 5 < 9 < 15
Therefore, $\frac{5}{6} \times 9 < 9 \times \frac{11}{11} < 9 \times \frac{5}{3}$
Line 1: $25 \times \frac{7}{18} < 25 \times \frac{4}{4} < 1\frac{3}{4} \times 25$
Line 2: $\frac{5}{6} \times 9 < 9 \times \frac{11}{11} < 9 \times \frac{5}{3}$
More Information
The problem focuses on understanding how multiplication affects scaling, especially when multiplying by fractions. Multiplying by a fraction less than 1 reduces the original number, while multiplying by a fraction greater than 1 increases it. Multiplying by 1 leaves the number unchanged.
Tips
A common mistake is not converting mixed numbers into improper fractions before multiplying. Another mistake is incorrectly simplifying fractions before or after multiplication, leading to incorrect results. For line 2, not recognizing that 11/11 = 1 can lead to mistakes
AI-generated content may contain errors. Please verify critical information