Understand the Problem
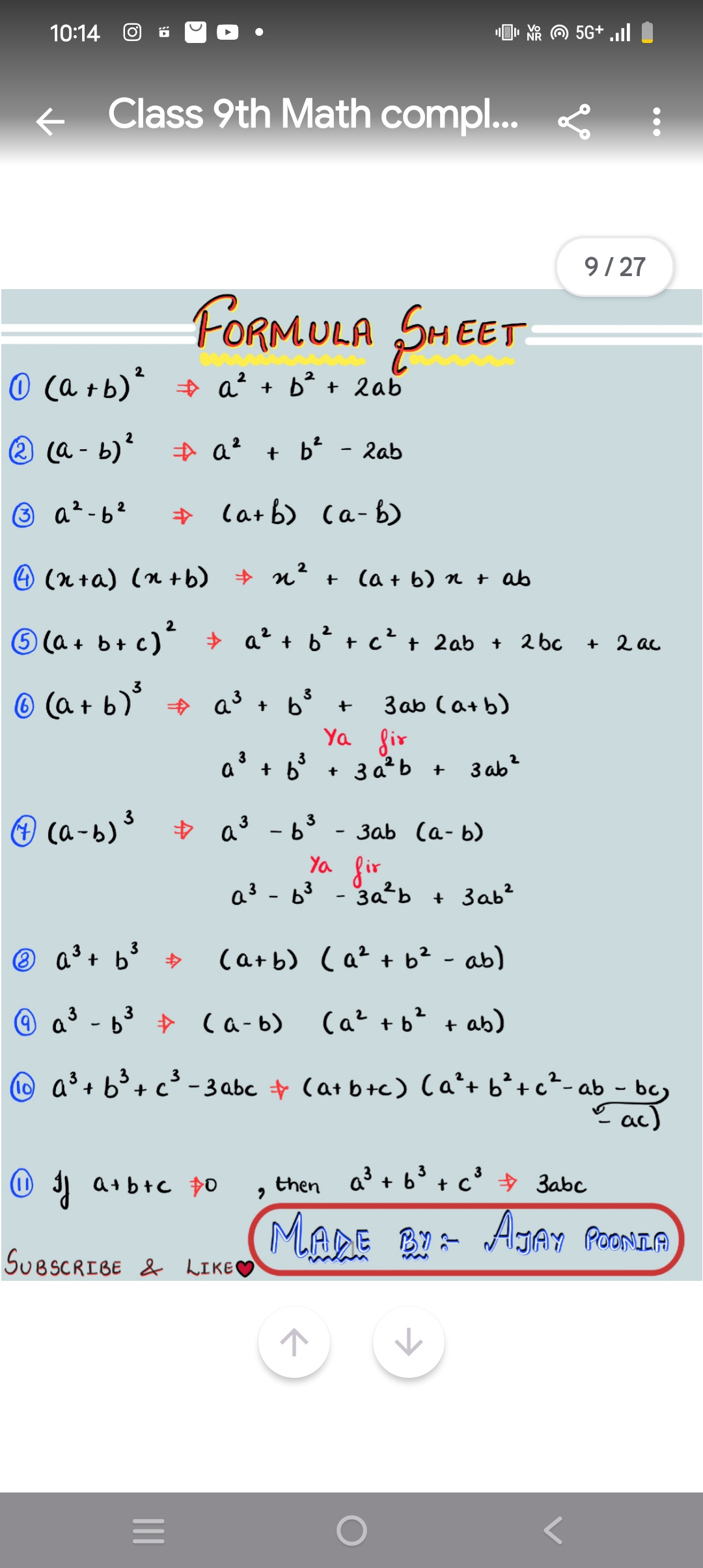
The image contains a collection of mathematical formulas relevant for Class 9 students, covering topics such as algebraic expansions and identities. It lists various identities involving sums and differences of squares and cubes, showcasing how they can be expressed and simplified.
Answer
The mathematical identities provided include expansions and factorization formulas essential for Class 9 algebra, such as $(a + b)² = a² + b² + 2ab$.
Answer for screen readers
The expanded forms and identities for Class 9 in the document are:
- $(a + b)² = a² + b² + 2ab$
- $(a - b)² = a² + b² - 2ab$
- $a² - b² = (a + b)(a - b)$
- $(x + a)(x + b) = x² + (a + b)x + ab$
- $(a + b + c)² = a² + b² + c² + 2ab + 2bc + 2ac$
- $(a + b)³ = a³ + b³ + 3ab(a + b)$
- $(a - b)³ = a³ - b³ - 3ab(a - b)$
- $a³ + b³ = (a + b)(a² - ab + b²)$
- $a³ - b³ = (a - b)(a² + ab + b²)$
- $a³ + b³ + c³ - 3abc = (a + b + c)(a² + b² + c² - ab - bc - ac)$
- If $a + b + c \neq 0$, then $a³ + b³ + c³ = 3abc$
Steps to Solve
- Expand (a + b)²
Using the formula for the square of a binomial:
$$(a + b)² = a² + b² + 2ab$$
- Expand (a - b)²
Similarly, for the difference of a binomial:
$$(a - b)² = a² + b² - 2ab$$
- Recognize the difference of squares
For the difference between two squares, we can use the formula:
$$a² - b² = (a + b)(a - b)$$
- Expand the product (x + a)(x + b)
Using the distributive property:
$$(x + a)(x + b) = x² + (a + b)x + ab$$
- Expand (a + b + c)²
This uses the formula for the square of a trinomial:
$$(a + b + c)² = a² + b² + c² + 2ab + 2bc + 2ac$$
- Expand (a + b)³
Using the binomial expansion formula for cubes:
$$(a + b)³ = a³ + b³ + 3ab(a + b)$$
- Expand (a - b)³
Similar to the previous step but for a minus:
$$(a - b)³ = a³ - b³ - 3ab(a - b)$$
- Factor a³ + b³
This can be factored as:
$$a³ + b³ = (a + b)(a² - ab + b²)$$
- Factor a³ - b³
Using the difference of cubes:
$$a³ - b³ = (a - b)(a² + ab + b²)$$
- Expand a³ + b³ + c³ - 3abc
This relates to the factorization involving three variables:
$$a³ + b³ + c³ - 3abc = (a + b + c)(a² + b² + c² - ab - bc - ac)$$
- Condition for a³ + b³ + c³ = 3abc
If $a + b + c \neq 0$, then:
$$a³ + b³ + c³ = 3abc$$
The expanded forms and identities for Class 9 in the document are:
- $(a + b)² = a² + b² + 2ab$
- $(a - b)² = a² + b² - 2ab$
- $a² - b² = (a + b)(a - b)$
- $(x + a)(x + b) = x² + (a + b)x + ab$
- $(a + b + c)² = a² + b² + c² + 2ab + 2bc + 2ac$
- $(a + b)³ = a³ + b³ + 3ab(a + b)$
- $(a - b)³ = a³ - b³ - 3ab(a - b)$
- $a³ + b³ = (a + b)(a² - ab + b²)$
- $a³ - b³ = (a - b)(a² + ab + b²)$
- $a³ + b³ + c³ - 3abc = (a + b + c)(a² + b² + c² - ab - bc - ac)$
- If $a + b + c \neq 0$, then $a³ + b³ + c³ = 3abc$
More Information
These identities are fundamental for understanding algebra and are often used in solving polynomial equations and simplifying expressions. Mastery of these concepts is essential for progressing in mathematics.
Tips
- Confusing the formulas, especially between squares and cubes.
- Failing to apply the negative sign correctly in binomial expansions.
- Skipping steps in the expansion process, which can lead to errors.
AI-generated content may contain errors. Please verify critical information