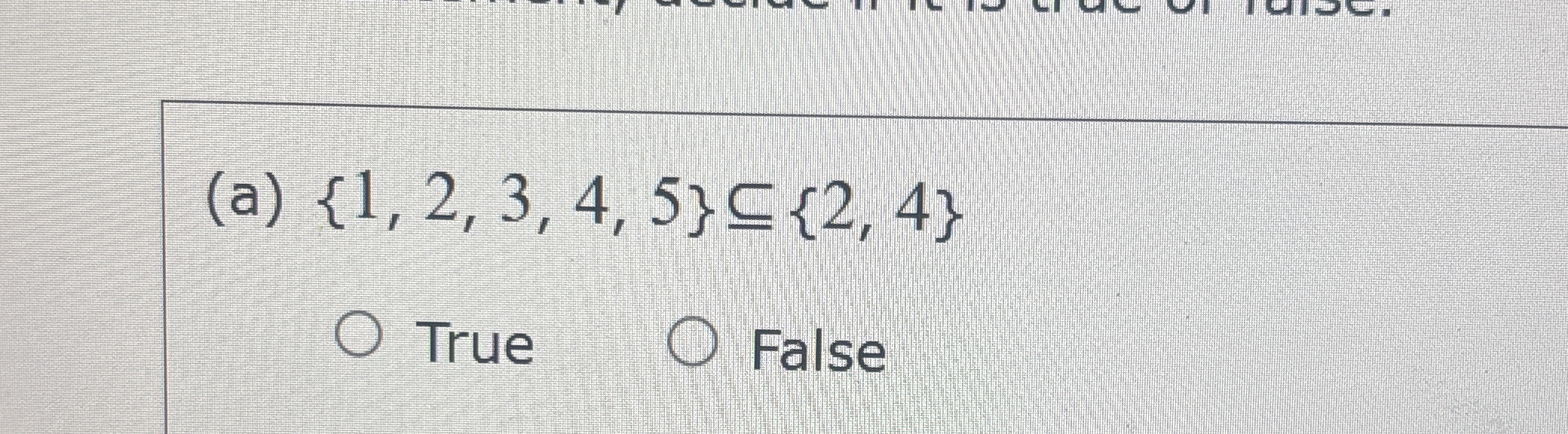
Is {1, 2, 3, 4, 5} a superset of {2, 4}?
Understand the Problem
The question asks us to determine if the set {1, 2, 3, 4, 5} is a superset of {2, 4}. In other words, whether {2, 4} is a subset of {1, 2, 3, 4, 5}.
Answer
True
Answer for screen readers
True
Steps to Solve
- Understand the superset symbol
The symbol $ \supseteq $ means "is a superset of or equal to". In this case, it means that the set on the left ({1, 2, 3, 4, 5}) must contain all the elements of the set on the right ({2, 4}).
- Check if all elements of the second set are in the first set
Examine the elements in the set {2, 4} and see if they are all present in the set {1, 2, 3, 4, 5}. The number 2 is in {1, 2, 3, 4, 5}. The number 4 is in {1, 2, 3, 4, 5}.
- Determine if the statement is True or False
Since all elements of {2, 4} are present in {1, 2, 3, 4, 5}, then {1, 2, 3, 4, 5} is indeed a superset of {2, 4}. Therefore, the statement is true.
True
More Information
A set A is a superset of a set B if all elements of B are also elements of A. In this specific case, {1, 2, 3, 4, 5} is a superset of {2, 4}.
Tips
A common mistake is confusing the direction of the subset/superset symbol. Remembering that the "open" side of the symbol faces the larger set can help avoid this. Another mistake is not checking every element, or incorrectly identifying the elements in either set.
AI-generated content may contain errors. Please verify critical information