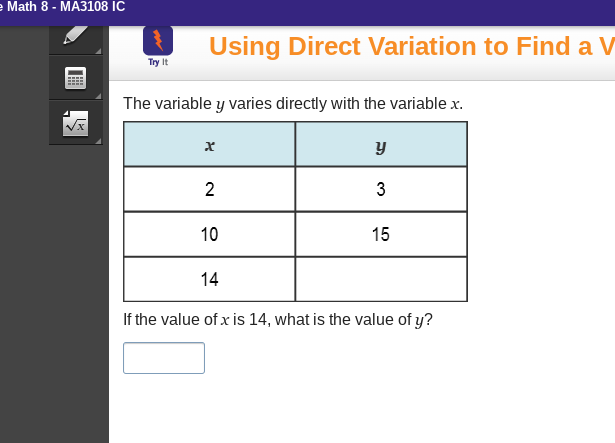
If the value of x is 14, what is the value of y?
Understand the Problem
The question asks us to find the value of y when x is given as 14, based on the direct variation between x and y, using the provided values in the table to deduce the relationship.
Answer
$y = 21$
Answer for screen readers
The value of (y) when (x = 14) is (21).
Steps to Solve
- Identify the direct variation relationship
In direct variation, $y$ can be expressed as $y = kx$, where $k$ is the constant of variation. To find $k$, we can use the values provided in the table.
- Calculate the constant of variation (k)
Using the first set of values, when $x = 2$ and $y = 3$: $$ k = \frac{y}{x} = \frac{3}{2} = 1.5 $$
- Verify the constant with another pair of values
Let's verify with the pair $x = 10$ and $y = 15$: $$ k = \frac{15}{10} = 1.5 $$
This confirms that our constant (k) is consistent.
- Use the constant to find (y) when (x = 14)
Now, substitute (x = 14) into the equation (y = kx): $$ y = 1.5 \times 14 $$
- Calculate (y)
Perform the multiplication: $$ y = 21 $$
The value of (y) when (x = 14) is (21).
More Information
In direct variation, the ratio between the variables remains constant. This means as one variable increases, the other increases proportionally. Here, we found this relationship by using the initial values provided in the problem.
Tips
- Failing to calculate the constant of variation correctly.
- Not verifying the constant with multiple values, which could lead to errors.
AI-generated content may contain errors. Please verify critical information