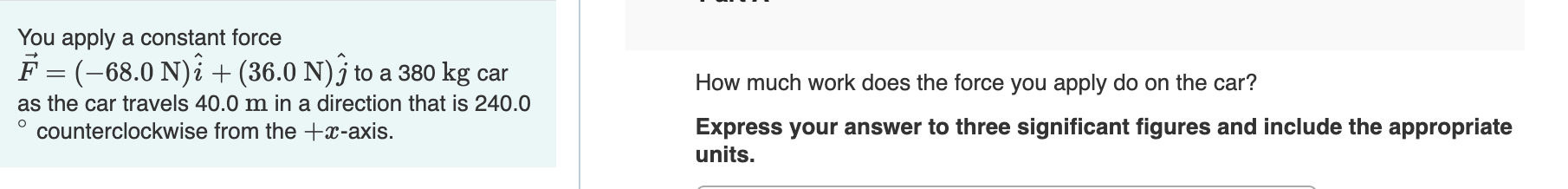
How much work does the force you apply do on the car? Express your answer to three significant figures and include the appropriate units.
Understand the Problem
The question is asking how much work is done by a force applied to a car as it travels a certain distance in a specified direction. To solve this, we will use the formula for work, which is the dot product of the force and the displacement. The force vector and the angle will also need to be taken into account.
Answer
$W \approx 113 \, \text{J}$
Answer for screen readers
$W \approx 113 , \text{J}$
Steps to Solve
- Understand the components of force and displacement
The force vector is given by ( \vec{F} = (-68.0 , \text{N}) \hat{i} + (36.0 , \text{N}) \hat{j} ). The displacement ( d ) is given as 40.0 m at an angle of 240.0° counterclockwise from the ( +x )-axis. We need to find the components of the displacement vector.
- Calculate the components of the displacement vector
Using trigonometry, the ( x ) and ( y ) components of the displacement can be found using:
[ d_x = d \cos(\theta) \quad \text{and} \quad d_y = d \sin(\theta) ]
Where ( d = 40.0 , \text{m} ) and ( \theta = 240.0^\circ ).
Calculating these:
[ d_x = 40.0 \cos(240.0^\circ) = 40.0 \times (-0.5) = -20.0 , \text{m} ] [ d_y = 40.0 \sin(240.0^\circ) = 40.0 \times (-\sqrt{3}/2) = -20.0 \sqrt{3} \approx -34.64 , \text{m} ]
Thus, the displacement vector is ( \vec{d} = (-20.0 , \text{m}) \hat{i} + (-34.64 , \text{m}) \hat{j} ).
- Calculate the work done using the dot product
The work done ( W ) by the force on the car is calculated using the dot product of the force and displacement vectors:
[ W = \vec{F} \cdot \vec{d} = F_x d_x + F_y d_y ]
Substituting in the components:
[ W = (-68.0) \cdot (-20.0) + (36.0) \cdot (-34.64) ]
Calculating each term:
[ W = 1360.0 - 1247.04 = 112.96 , \text{J} ]
- Final answer rounded to three significant figures
The work done on the car is approximately:
( W \approx 113 , \text{J} )
$W \approx 113 , \text{J}$
More Information
Work is defined as the transfer of energy when a force acts along a distance. In this scenario, the force acts at an angle to the displacement, demonstrating the importance of using both components to calculate work accurately.
Tips
- Not using the correct angle: Ensure that the angle used for calculating components is in the proper quadrant, as this can affect the sign of the components.
- Forgetting to convert degrees to radians: If using a calculator that requires radians, validate calculations accordingly.
- Miscalculating dot products: It's important to multiply the corresponding components correctly and sum them up.
AI-generated content may contain errors. Please verify critical information