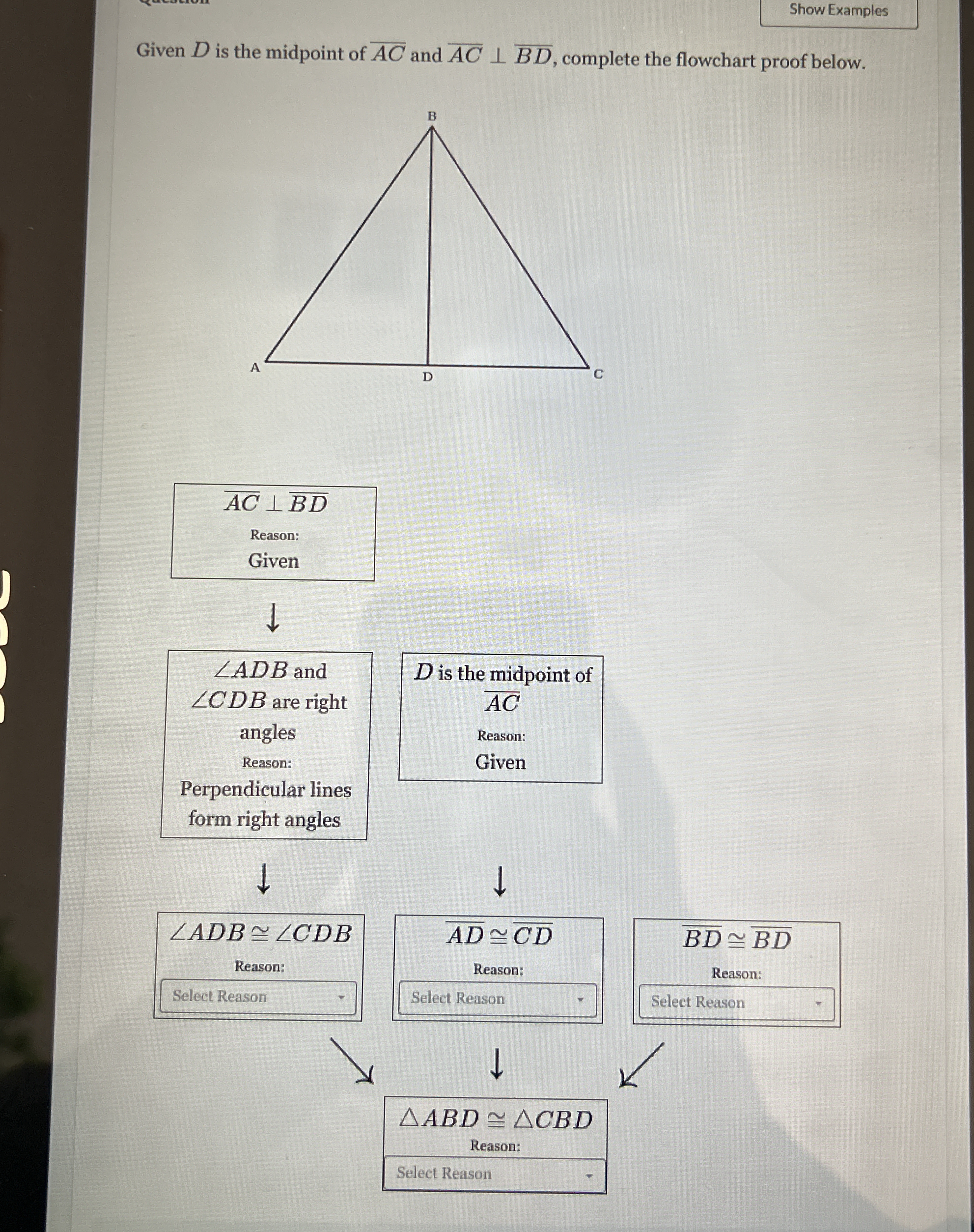
Given D is the midpoint of AC and AC is perpendicular to BD, complete the flowchart proof.
Understand the Problem
The problem asks to complete a flowchart proof given that D is the midpoint of AC and AC is perpendicular to BD. This involves stating the reasons for certain geometric relationships based on the given information and geometric principles. We need to identify the appropriate reasons for each step in the flowchart to prove that triangle ABD is congruent to triangle CBD.
Answer
$\angle ADB \cong \angle CDB$ Reason: All right angles are congruent. $\overline{AD} \cong \overline{CD}$ Reason: Definition of midpoint $\overline{BD} \cong \overline{BD}$ Reason: Reflexive Property $\triangle ABD \cong \triangle CBD$ Reason: SAS
Answer for screen readers
$\angle ADB \cong \angle CDB$ Reason: All right angles are congruent.
$\overline{AD} \cong \overline{CD}$ Reason: Definition of midpoint
$\overline{BD} \cong \overline{BD}$ Reason: Reflexive Property
$\triangle ABD \cong \triangle CBD$ Reason: SAS
Steps to Solve
- Reason for $\angle ADB \cong \angle CDB$
Since $\angle ADB$ and $\angle CDB$ are right angles, and all right angles are congruent, then $\angle ADB \cong \angle CDB$.
- Reason for $\overline{AD} \cong \overline{CD}$
Because $D$ is the midpoint of $\overline{AC}$, by the definition of a midpoint, $\overline{AD} \cong \overline{CD}$.
- Reason for $\overline{BD} \cong \overline{BD}$
$\overline{BD}$ is congruent to itself by the reflexive property.
- Reason for $\triangle ABD \cong \triangle CBD$
We have shown that $\overline{AD} \cong \overline{CD}$, $\angle ADB \cong \angle CDB$, and $\overline{BD} \cong \overline{BD}$. Therefore, by the Side-Angle-Side (SAS) congruence postulate, $\triangle ABD \cong \triangle CBD$.
$\angle ADB \cong \angle CDB$ Reason: All right angles are congruent.
$\overline{AD} \cong \overline{CD}$ Reason: Definition of midpoint
$\overline{BD} \cong \overline{BD}$ Reason: Reflexive Property
$\triangle ABD \cong \triangle CBD$ Reason: SAS
More Information
A flowchart proof uses boxes and arrows to show the logical progression of an argument. Each statement is placed in a box, and arrows connect the boxes to show how each statement leads to the next. The reason for each statement is written below the box.
Tips
A common mistake is incorrectly identifying the congruence postulate or theorem used to prove the triangles congruent. Make sure to carefully examine the sides and angles that have been proven congruent and select the appropriate postulate (e.g., SSS, SAS, ASA, AAS). Also, confusing definitions with theorems.
AI-generated content may contain errors. Please verify critical information