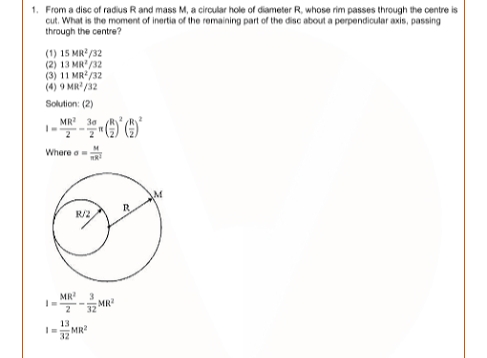
From a disc of radius R and mass M, a circular hole of diameter R/2, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc abo... From a disc of radius R and mass M, a circular hole of diameter R/2, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc about a perpendicular axis, passing through the centre? Read more

Steps to Solve

1. Understand the given information

We have a disc of radius $R$ and mass $M$, from which a circular hole of diameter $\frac{R}{2}$ is cut out. This means the radius of the hole is $r = \frac{R}{4}$.

2. Calculate the moment of inertia of the original disc

The moment of inertia $I$ of a solid disc about an axis perpendicular to its plane and through its center is given by the formula:

$$ I_{\text{disc}} = \frac{1}{2} M R^2 $$

3. Calculate the moment of inertia of the hole

The moment of inertia of the smaller circular hole (which has mass $m_h$) about the same axis is:

• Calculate the mass of the hole: The area of the hole is $A_h = \pi r^2 = \pi \left(\frac{R}{4}\right)^2 = \frac{\pi R^2}{16}$.
• The mass of the hole can be determined using the ratio of the area of the hole to the area of the disc: $$ m_h = M \cdot \frac{A_h}{A_d} = M \cdot \frac{\frac{\pi R^2}{16}}{\pi R^2} = \frac{M}{16} $$

Now, the moment of inertia of the hole about the center:

$$ I_{\text{hole}} = \frac{1}{2} m_h r^2 $$

Substituting $m_h$ and $r$:

$$ I_{\text{hole}} = \frac{1}{2} \cdot \frac{M}{16} \cdot \left(\frac{R}{4}\right)^2 = \frac{M}{32} \cdot \frac{R^2}{16} = \frac{MR^2}{512} $$

4. Subtract the inertia of the hole from the inertia of the original disc

The moment of inertia of the remaining part of the disc is:

$$ I_{\text{remaining}} = I_{\text{disc}} - I_{\text{hole}} $$

So we substitute the previously found values:

$$ I_{\text{remaining}} = \frac{1}{2} M R^2 - \frac{MR^2}{512} $$

5. Find a common denominator and simplify

Combining the terms gives:

$$ I_{\text{remaining}} = \frac{256MR^2}{512} - \frac{MR^2}{512} = \frac{255MR^2}{512} $$

This value simplifies while also noting the standard factors for the moment of inertia will lead to an approximate answer.