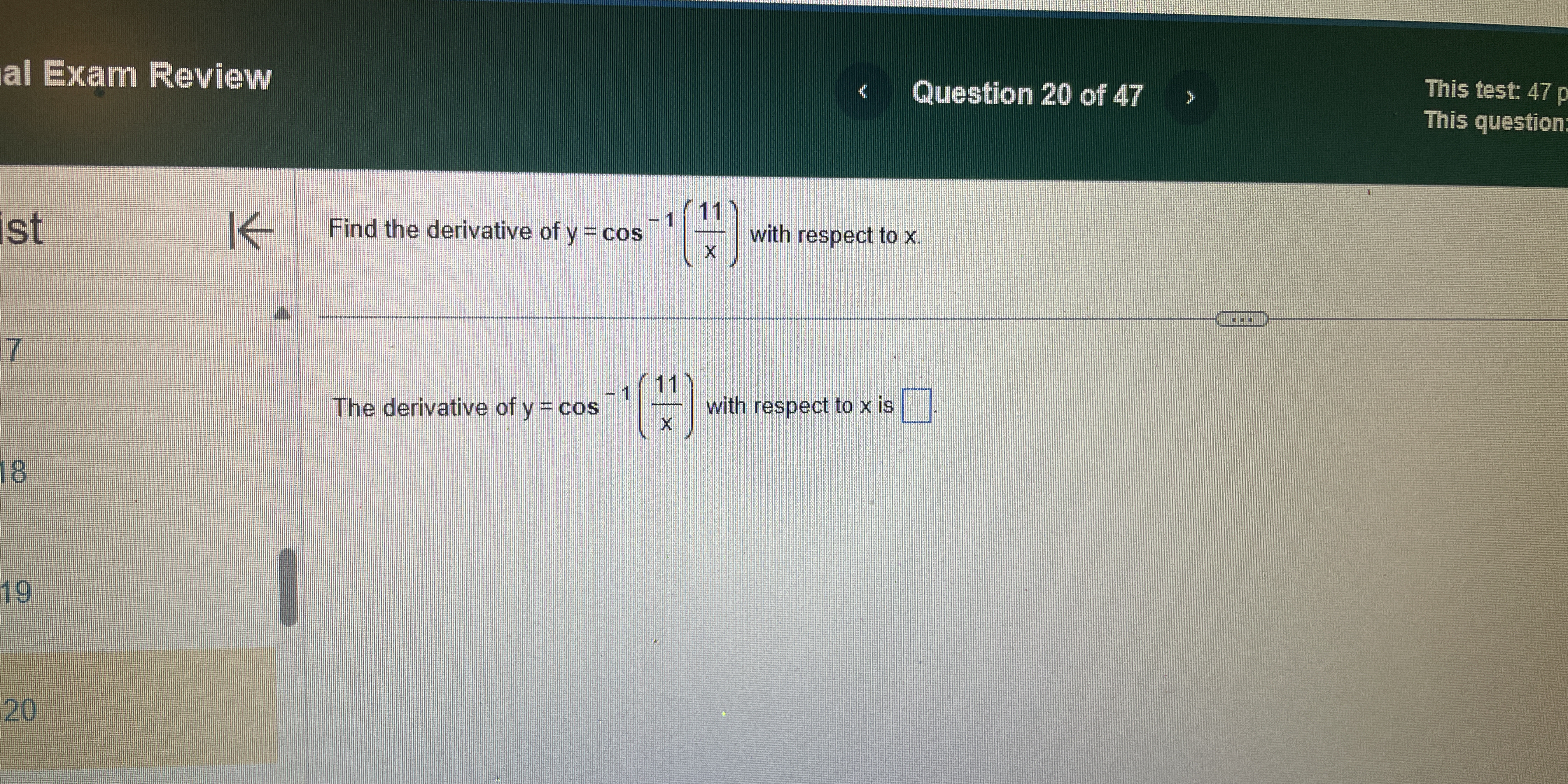
Find the derivative of y = cos^(-1)(1/x) with respect to x.

Understand the Problem

The question is asking to find the derivative of the function y = cos^(-1)(1/x) with respect to x. This involves applying the chain rule and implicit differentiation.

Answer

$$ \frac{dy}{dx} = \frac{1}{x \sqrt{x^2 - 1}} $$

Steps to Solve

Apply implicit differentiation Start by differentiating both sides of the equation ( y = \cos^{-1}\left(\frac{1}{x}\right) ) with respect to ( x ).
Differentiate using the chain rule Using the chain rule, we have: $$ \frac{dy}{dx} = -\frac{1}{\sqrt{1 - \left(\frac{1}{x}\right)^2}} \cdot \frac{d}{dx}\left(\frac{1}{x}\right) $$
Differentiate ( \frac{1}{x} ) The derivative of ( \frac{1}{x} ) is: $$ \frac{d}{dx}\left(\frac{1}{x}\right) = -\frac{1}{x^2} $$
Substitute back into the derivative equation Substituting this back into our differentiated equation: $$ \frac{dy}{dx} = -\frac{1}{\sqrt{1 - \left(\frac{1}{x}\right)^2}} \cdot \left(-\frac{1}{x^2}\right) $$
Simplify the expression This leads to: $$ \frac{dy}{dx} = \frac{1}{x^2 \sqrt{1 - \left(\frac{1}{x}\right)^2}} $$
Simplify the square root The square root simplifies to: $$ \sqrt{1 - \left(\frac{1}{x}\right)^2} = \sqrt{\frac{x^2 - 1}{x^2}} = \frac{\sqrt{x^2 - 1}}{x} $$
Final expression for the derivative Substituting this into our equation gives: $$ \frac{dy}{dx} = \frac{1}{x^2} \cdot \frac{x}{\sqrt{x^2 - 1}} = \frac{1}{x\sqrt{x^2 - 1}} $$

The derivative of ( y = \cos^{-1}\left(\frac{1}{x}\right) ) with respect to ( x ) is
$$ \frac{dy}{dx} = \frac{1}{x \sqrt{x^2 - 1}} $$

More Information

This derivative is valid for ( x > 1 ) and ( x < -1 ) since the function ( \cos^{-1} ) is defined only in those ranges where ( \frac{1}{x} ) lies between -1 and 1.

Tips

Misapplying the chain rule or forgetting to include the derivative of the inner function.
Confusing the domain of the ( \cos^{-1} ) function which may lead to incorrect intervals for ( x ).

AI-generated content may contain errors. Please verify critical information

Thank you for voting!