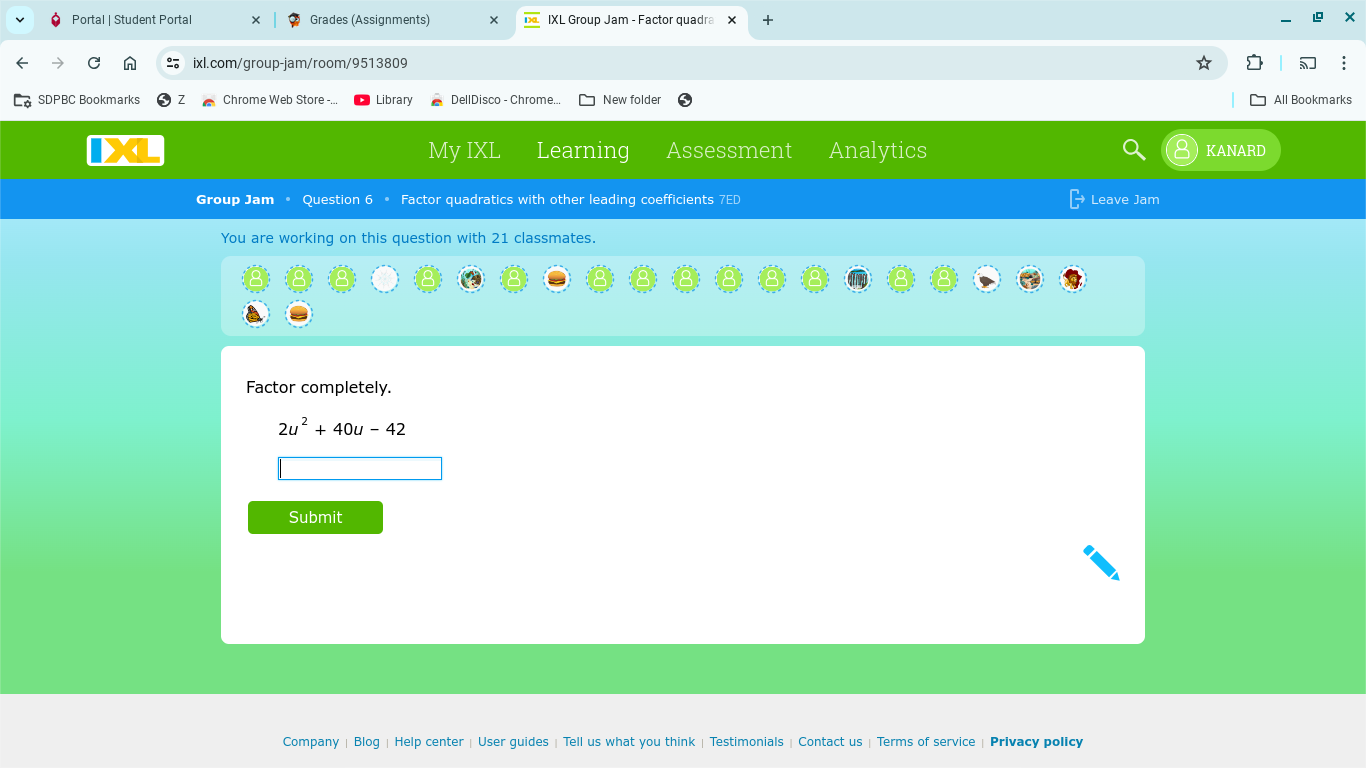
Factor completely: 2u² + 40u - 42
Understand the Problem
The question is asking to completely factor the quadratic expression given, which is 2u² + 40u - 42. This involves applying factoring techniques such as identifying common factors and possibly using methods like the quadratic formula if needed.
Answer
The completely factored form is $2(u + 21)(u - 1)$.
Answer for screen readers
The completely factored form of the expression $2u^2 + 40u - 42$ is:
$$ 2(u + 21)(u - 1) $$
Steps to Solve
- Identify Common Factors
First, look for any common factors in the expression $2u^2 + 40u - 42$. The coefficients are $2$, $40$, and $-42$. The greatest common factor of these numbers is $2$.
- Factor Out the Common Factor
Factor out $2$ from the expression:
$$ 2(u^2 + 20u - 21) $$
- Factor the Quadratic Expression
Next, we need to factor the quadratic $u^2 + 20u - 21$. We want two numbers that multiply to $-21$ (constant term) and add to $20$ (coefficient of $u$).
The numbers that satisfy this are $21$ and $-1$. Therefore, we can rewrite the quadratic factor:
$$ u^2 + 20u - 21 = (u + 21)(u - 1) $$
- Combine All Factors
Now combine all the factors:
$$ 2(u + 21)(u - 1) $$
Thus, the completely factored form of the original expression is:
$$ 2(u + 21)(u - 1) $$
The completely factored form of the expression $2u^2 + 40u - 42$ is:
$$ 2(u + 21)(u - 1) $$
More Information
Factoring quadratics is a crucial skill in algebra. Factoring makes it easier to solve equations and understand graph behavior. The process involves finding integers or expressions that fit the requirements related to multiplication and addition of coefficients.
Tips
- Ignoring the Common Factor: Sometimes students forget to factor out the greatest common factor before factoring the quadratic.
- Incorrect Pairing of Factors: It’s common to overlook the correct pairs that multiply to the constant term; always check both conditions (sum and product).
- Not Double-Checking: After factoring, it's important to expand back to the original expression to ensure correctness.
AI-generated content may contain errors. Please verify critical information