Evaluate the limit expression in image.
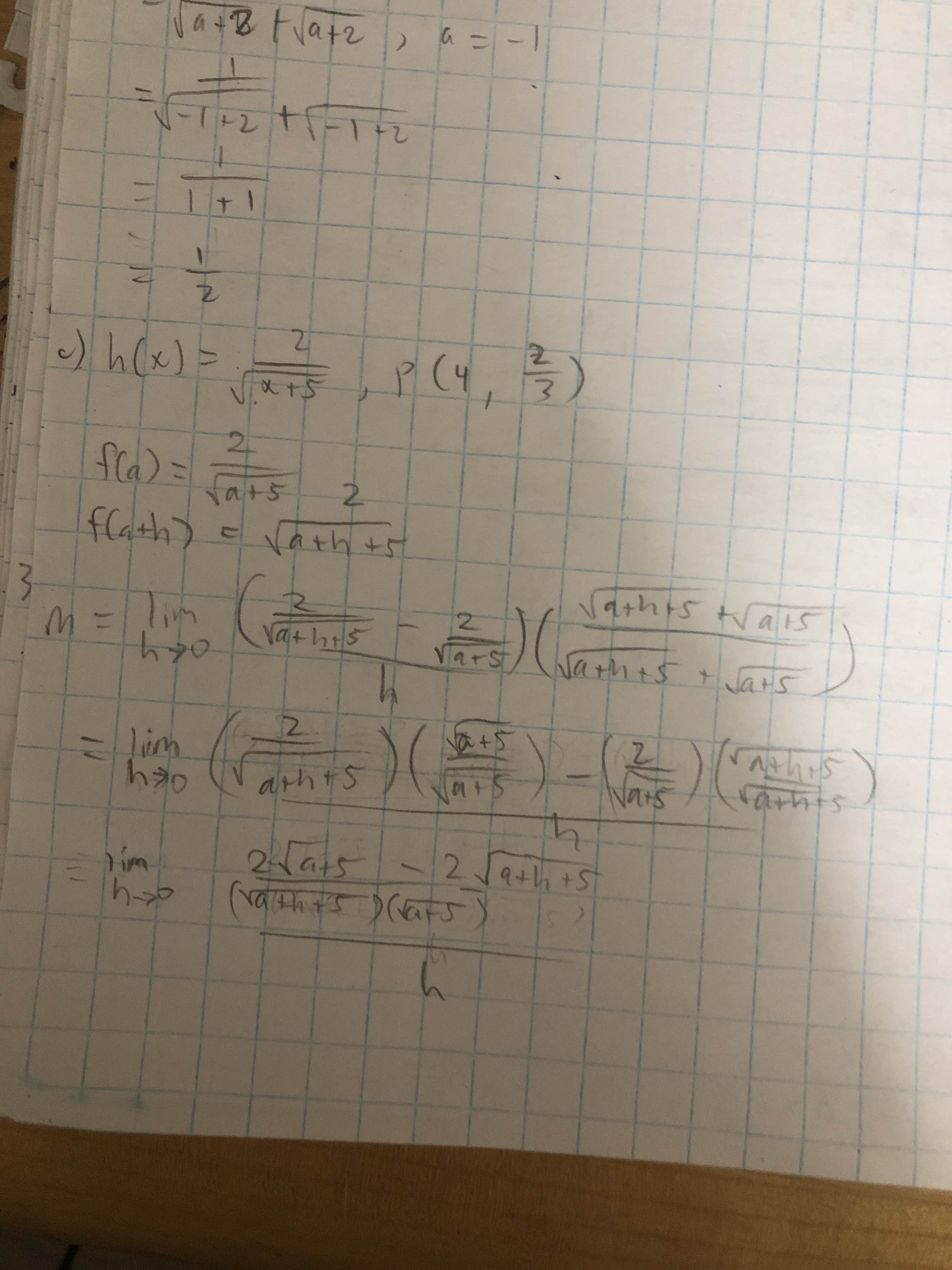
Understand the Problem
The image contains handwritten mathematical expressions and equations. It appears to be evaluating limits of expressions involving square roots. Specifically, it looks like there are two problems, the first one involves evaluating (\sqrt{a+2}) and the second involves finding the derivative of (h(x) = \frac{2}{\sqrt{x+5}}) by using first principles given P(4, 2/3).
Answer
$ -\frac{1}{27} $
Answer for screen readers
$ -\frac{1}{27} $
Steps to Solve
-
Simplify the limit expression by multiplying by the conjugate We are given the limit expression: $$ \lim_{h\to 0} \frac{\frac{2}{\sqrt{a+h+5}} - \frac{2}{\sqrt{a+5}}}{h} $$ Multiply the numerator and denominator by the conjugate of the numerator to get rid of the complex fraction in the numerator: $$ \lim_{h\to 0} \frac{\frac{2}{\sqrt{a+h+5}} - \frac{2}{\sqrt{a+5}}}{h} \cdot \frac{\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}}}{\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}}} $$
-
Simplify the numerator $$ \lim_{h\to 0} \frac{\frac{4}{a+h+5} - \frac{4}{a+5}}{h(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$
-
Combine the fractions in the numerator $$ \lim_{h\to 0} \frac{\frac{4(a+5) - 4(a+h+5)}{(a+h+5)(a+5)}}{h(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$ $$ \lim_{h\to 0} \frac{\frac{4a+20 - 4a-4h-20}{(a+h+5)(a+5)}}{h(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$ $$ \lim_{h\to 0} \frac{\frac{-4h}{(a+h+5)(a+5)}}{h(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$
-
Cancel $h$ and simplify Combining the fractions, $$ \lim_{h\to 0} \frac{-4h}{h(a+h+5)(a+5)(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$ Cancel out $h$: $$ \lim_{h\to 0} \frac{-4}{(a+h+5)(a+5)(\frac{2}{\sqrt{a+h+5}} + \frac{2}{\sqrt{a+5}})} $$
-
Substitute $a = 4$ and $h = 0$ Substitute $a = 4$ and $h = 0$ into the limit $$ \frac{-4}{(4+0+5)(4+5)(\frac{2}{\sqrt{4+0+5}} + \frac{2}{\sqrt{4+5}})} $$ $$ \frac{-4}{(9)(9)(\frac{2}{\sqrt{9}} + \frac{2}{\sqrt{9}})} $$ $$ \frac{-4}{81(\frac{2}{3} + \frac{2}{3})} $$ $$ \frac{-4}{81(\frac{4}{3})} $$ $$ \frac{-4}{108} $$
-
Simplify the answer $$ \frac{-1}{27} $$
$ -\frac{1}{27} $
More Information
The derivative of $h(x)$ at $x=4$ is $-\frac{1}{27}$. This represents the slope of the tangent line to the curve $h(x) = \frac{2}{\sqrt{x+5}}$ at the point $(4, \frac{2}{3})$.
Tips
- Algebraic errors in simplification: When manipulating complex fractions and square roots, it's easy to make algebraic errors. Double-checking each step is crucial.
- Incorrectly applying the conjugate: The conjugate must be multiplied correctly in both the numerator and the denominator.
AI-generated content may contain errors. Please verify critical information