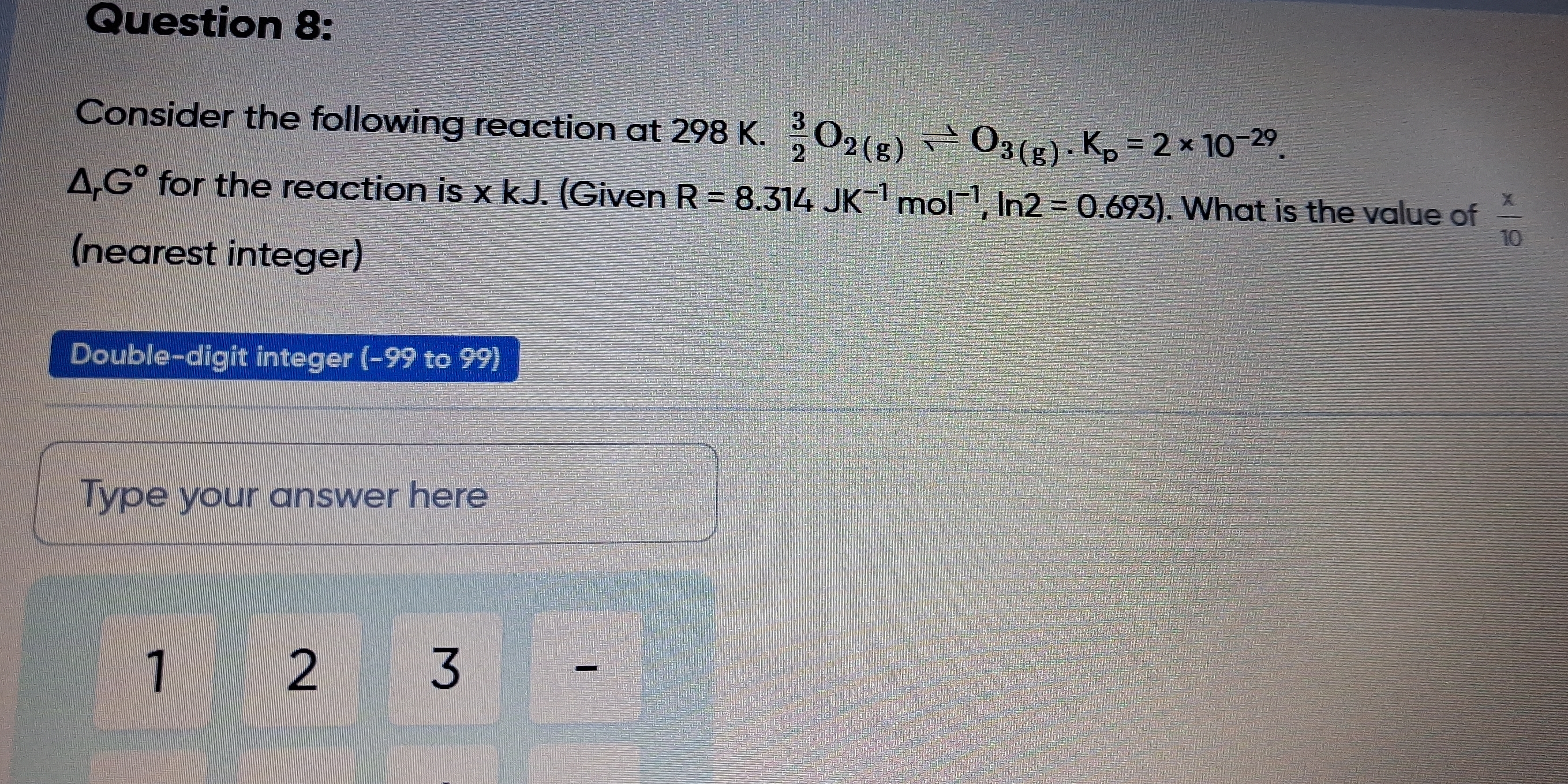
Consider the following reaction at 298 K. ΔG° for the reaction is x kJ. (Given R = 8.314 JK⁻¹ mol⁻¹, ln2 = 0.693). What is the value of x/10 (nearest integer)?

Steps to Solve

1. Identify the given values The equilibrium constant ( K_p = 2 \times 10^{-29} ) and the temperature ( T = 298 , K ). The values for the gas constant are ( R = 8.314 , J , K^{-1} , mol^{-1} ) and ( \ln(2) = 0.693 ).

2. Convert ( K_p ) to the correct units Since ( K_p ) is a pressure equilibrium constant, we will need to use it directly.

3. Calculate ( \Delta G^\circ ) using the equation The equation to calculate standard Gibbs free energy change is:

$$ \Delta G^\circ = -RT \ln K $$

Substituting in the known values:

$$ \Delta G^\circ = - (8.314 , J , K^{-1} , mol^{-1}) \times 298 , K \times \ln(2 \times 10^{-29}) $$

4. Calculate ( \ln K ) Use the properties of logarithms to find ( \ln(2 \times 10^{-29}) ):

$$ \ln(2 \times 10^{-29}) = \ln(2) + \ln(10^{-29}) = 0.693 - 29 \ln(10) $$

Using ( \ln(10) \approx 2.303 ):

$$ \ln(2 \times 10^{-29}) \approx 0.693 - 29 \times 2.303 $$

Now calculate ( 29 \times 2.303 ):

$$ 29 \times 2.303 \approx 66.787 $$

So now we have:

$$ \ln(2 \times 10^{-29}) \approx 0.693 - 66.787 \approx -66.094 $$

5. Substitute ( \ln K ) back into the Gibbs free energy equation Now substitute ( \ln K ) back to find ( \Delta G^\circ ):

$$ \Delta G^\circ \approx - (8.314) \times 298 \times (-66.094) $$

Calculating this:

$$ \Delta G^\circ \approx 8.314 \times 298 \times 66.094 $$

Calculating step-by-step:

• First calculate ( 8.314 \times 298 \approx 2477.572 )

Then multiply by ( 66.094 ):

$$ \Delta G^\circ \approx 2477.572 \times 66.094 \approx 163,323.9 , J = 163.32 , kJ $$

6. Calculate ( \frac{x}{10} ) and round Now divide by 10:

$$ \frac{163.32}{10} \approx 16.332 $$

Rounding to the nearest integer gives ( 16 ).