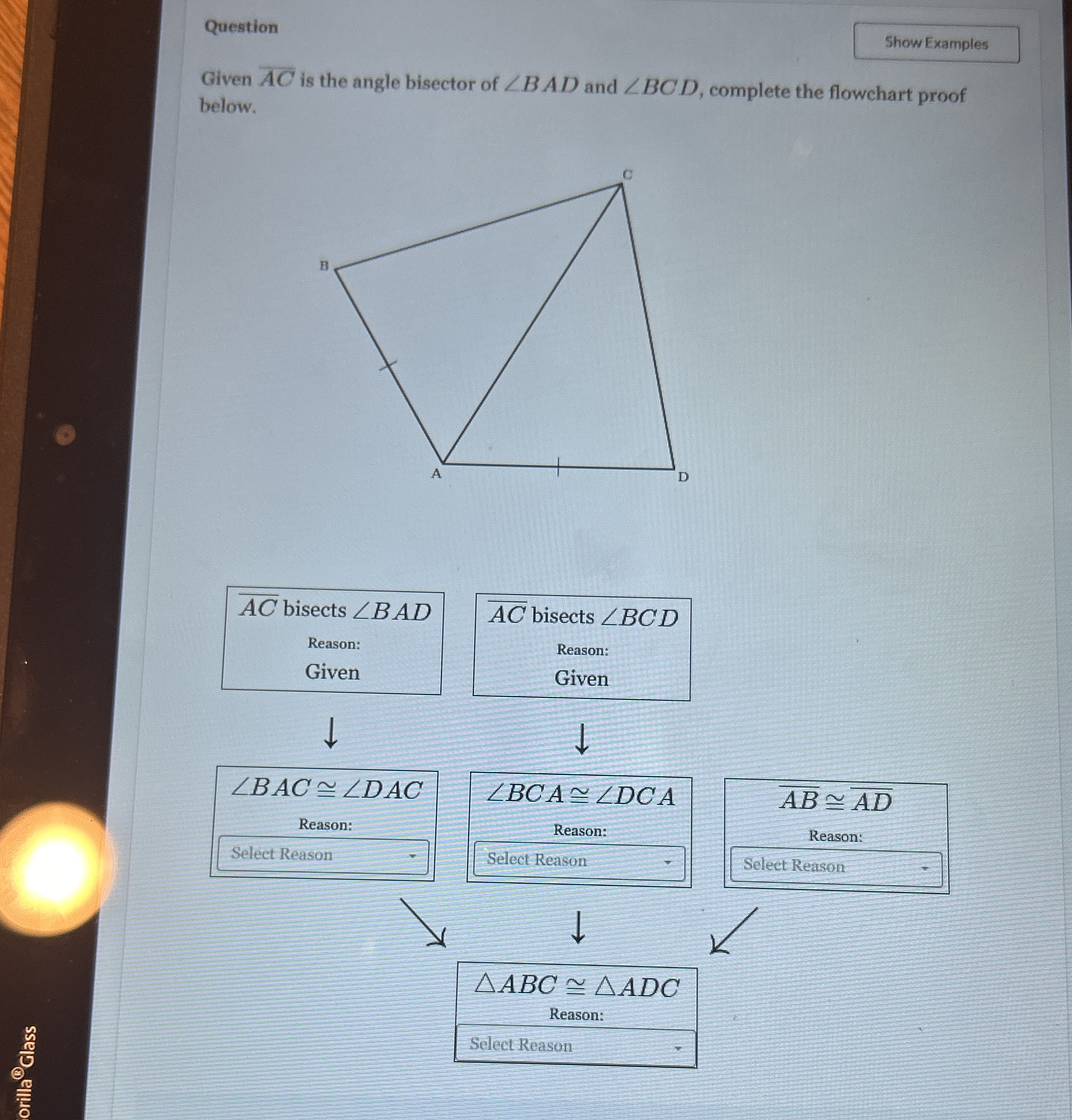
Complete the flowchart proof given that AC is the angle bisector of ∠BAD and ∠BCD.
Understand the Problem
You're asked to complete a flowchart proof, given that AC is the angle bisector of ∠BAD and ∠BCD. This involves identifying the reasons for each step in the proof to show that triangle ABC is congruent to triangle ADC.
Answer
$\angle BAC \cong \angle DAC$ : Definition of an Angle Bisector $\angle BCA \cong \angle DCA$ : Definition of an Angle Bisector $\overline{AC} \cong \overline{AC}$ : Reflexive Property $\triangle ABC \cong \triangle ADC$ : Angle-Side-Angle (ASA)
Answer for screen readers
(\angle BAC \cong \angle DAC) Reason: Definition of an Angle Bisector
(\angle BCA \cong \angle DCA) Reason: Definition of an Angle Bisector
(\overline{AC} \cong \overline{AC}) Reason: Reflexive Property
(\triangle ABC \cong \triangle ADC) Reason: Angle-Side-Angle (ASA)
Steps to Solve
- Determine the reason for $\angle BAC \cong \angle DAC$
Since $\overline{AC}$ bisects $\angle BAD$, it divides the angle into two congruent angles. Therefore, $\angle BAC \cong \angle DAC$ because of the definition of an angle bisector.
- Determine the reason for $\angle BCA \cong \angle DCA$
Similarly, since $\overline{AC}$ bisects $\angle BCD$, it divides the angle into two congruent angles. Thus, $\angle BCA \cong \angle DCA$ because of the definition of an angle bisector.
- Determine the reason for $\overline{AC} \cong \overline{AC}$
$\overline{AC}$ is congruent to itself. This is due to the Reflexive Property
- Determine the reason for $\triangle ABC \cong \triangle ADC$
We have two angles and a side.
$\angle BAC \cong \angle DAC$ $\angle BCA \cong \angle DCA$ $\overline{AC} \cong \overline{AC}$
Therefore, $\triangle ABC \cong \triangle ADC$ by Angle-Side-Angle (ASA) congruence.
(\angle BAC \cong \angle DAC) Reason: Definition of an Angle Bisector
(\angle BCA \cong \angle DCA) Reason: Definition of an Angle Bisector
(\overline{AC} \cong \overline{AC}) Reason: Reflexive Property
(\triangle ABC \cong \triangle ADC) Reason: Angle-Side-Angle (ASA)
More Information
Flowchart proofs are a way of organizing geometric proofs, visually showing the logical flow from given information to the conclusion.
Tips
A common mistake is confusing the Angle-Side-Angle (ASA) and Angle-Angle-Side (AAS) congruence theorems. In ASA, the side must be between the two angles.
AI-generated content may contain errors. Please verify critical information