Calculate the volume of the spheres.
Understand the Problem
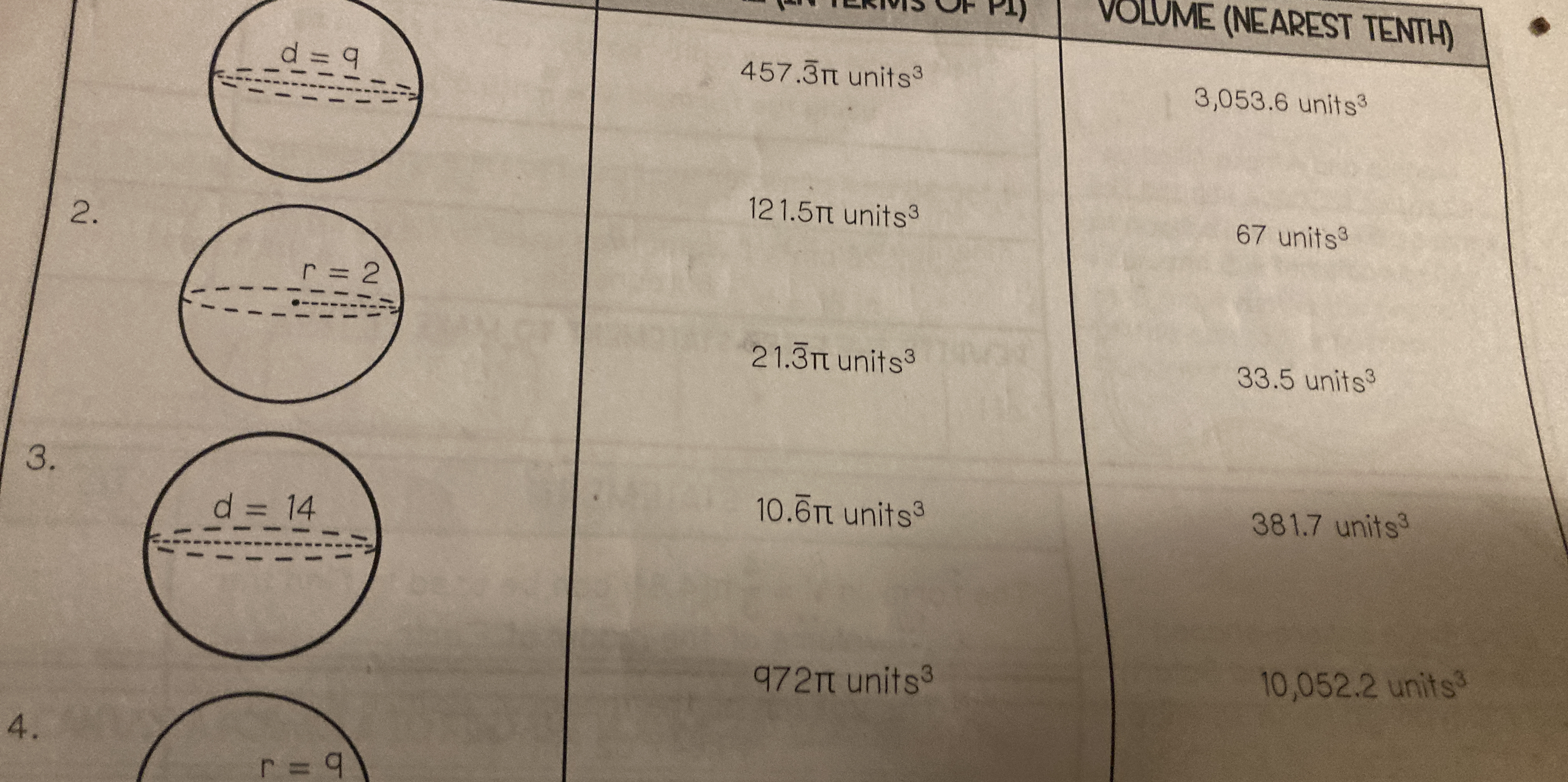
The image presents math problems involving spheres where you're given either the diameter (d) or the radius (r) and the volume of different spheres. The objective is to calculate the volume of these spheres either in terms of pi or to the nearest tenth.
Answer
2. d = 9: $121.5\pi$ units$^3$, $381.7$ units$^3$; r = 2: $10.7\pi$ units$^3$, $33.5$ units$^3$ 3. d = 14: $457.3\pi$ units$^3$, $1436.8$ units$^3$ 4. r = 9: $972\pi$ units$^3$, $3053.6$ units$^3$
Answer for screen readers
d = 9 $121.5\pi$ units$^3$ $381.7$ units$^3$ r = 2 $10.7\pi$ units$^3$ $33.5$ units$^3$ 3. d = 14 $457.3\pi$ units$^3$ $1436.8$ units$^3$ 4. r = 9 $972\pi$ units$^3$ $3053.6$ units$^3$
Steps to Solve
- Find the volume of the sphere given d = 9
Since the diameter is 9, the radius $r$ is $d/2 = 9/2 = 4.5$. The volume of a sphere is given by the formula: $V = \frac{4}{3} \pi r^3$ Substituting $r = 4.5$: $V = \frac{4}{3} \pi (4.5)^3 = \frac{4}{3} \pi (91.125) = 121.5 \pi$ So the volume in terms of $\pi$ is $121.5\pi$ units$^3$.
- Find the volume to the nearest tenth
$V = 121.5 \pi \approx 121.5 \times 3.14159265359 \approx 381.7$ So the volume to the nearest tenth is $381.7$ units$^3$.
- Find the volume of the sphere given r = 2
The radius is given as $r = 2$. The volume of a sphere is given by the formula: $V = \frac{4}{3} \pi r^3$ Substituting $r = 2$: $V = \frac{4}{3} \pi (2)^3 = \frac{4}{3} \pi (8) = \frac{32}{3} \pi \approx 10.666... \pi$ Rounding to the nearest tenth, we have $10.7\pi$ units$^3$
-
Find the volume to the nearest tenth $V = \frac{32}{3} \pi \approx 10.666... \times 3.14159265359 \approx 33.5103216383...$ Rounding this to the nearest tenth gives $33.5$ units$^3$.
-
Find the volume of the sphere given d = 14 Since the diameter is 14, the radius $r$ is $d/2 = 14/2 = 7$. The volume of a sphere is given by the formula: $V = \frac{4}{3} \pi r^3$ Substituting $r = 7$: $V = \frac{4}{3} \pi (7)^3 = \frac{4}{3} \pi (343) = \frac{1372}{3} \pi \approx 457.333... \pi$ Rounding to the nearest tenth, we have $457.3\pi$ units$^3$
-
Find the volume to the nearest tenth $V = \frac{1372}{3} \pi \approx 457.333... \times 3.14159265359 \approx 1436.75504024...$ Rounding this to the nearest tenth gives $1436.8$ units$^3$
-
Find the volume of the sphere given r = 9
The radius is given as $r = 9$. The volume of a sphere is given by the formula: $V = \frac{4}{3} \pi r^3$ Substituting $r = 9$: $V = \frac{4}{3} \pi (9)^3 = \frac{4}{3} \pi (729) = 4 \pi (243) = 972 \pi$ units$^3$
- Find the volume to the nearest tenth $V = 972 \pi \approx 972 \times 3.14159265359 \approx 3053.62805928...$ Rounding to the nearest tenth gives $3053.6$ units$^3$
d = 9 $121.5\pi$ units$^3$ $381.7$ units$^3$ r = 2 $10.7\pi$ units$^3$ $33.5$ units$^3$ 3. d = 14 $457.3\pi$ units$^3$ $1436.8$ units$^3$ 4. r = 9 $972\pi$ units$^3$ $3053.6$ units$^3$
More Information
The volume of a sphere can be calculated if either the radius or the diameter is known. The diameter is simply twice the radius.
Tips
A common mistake is using the diameter instead of the radius in the volume formula. Remember to divide the diameter by 2 to get the radius before plugging it into the formula. Another common mistake is incorrectly rounding to the nearest tenth, which is one decimal place.
AI-generated content may contain errors. Please verify critical information