Base area of prisms?
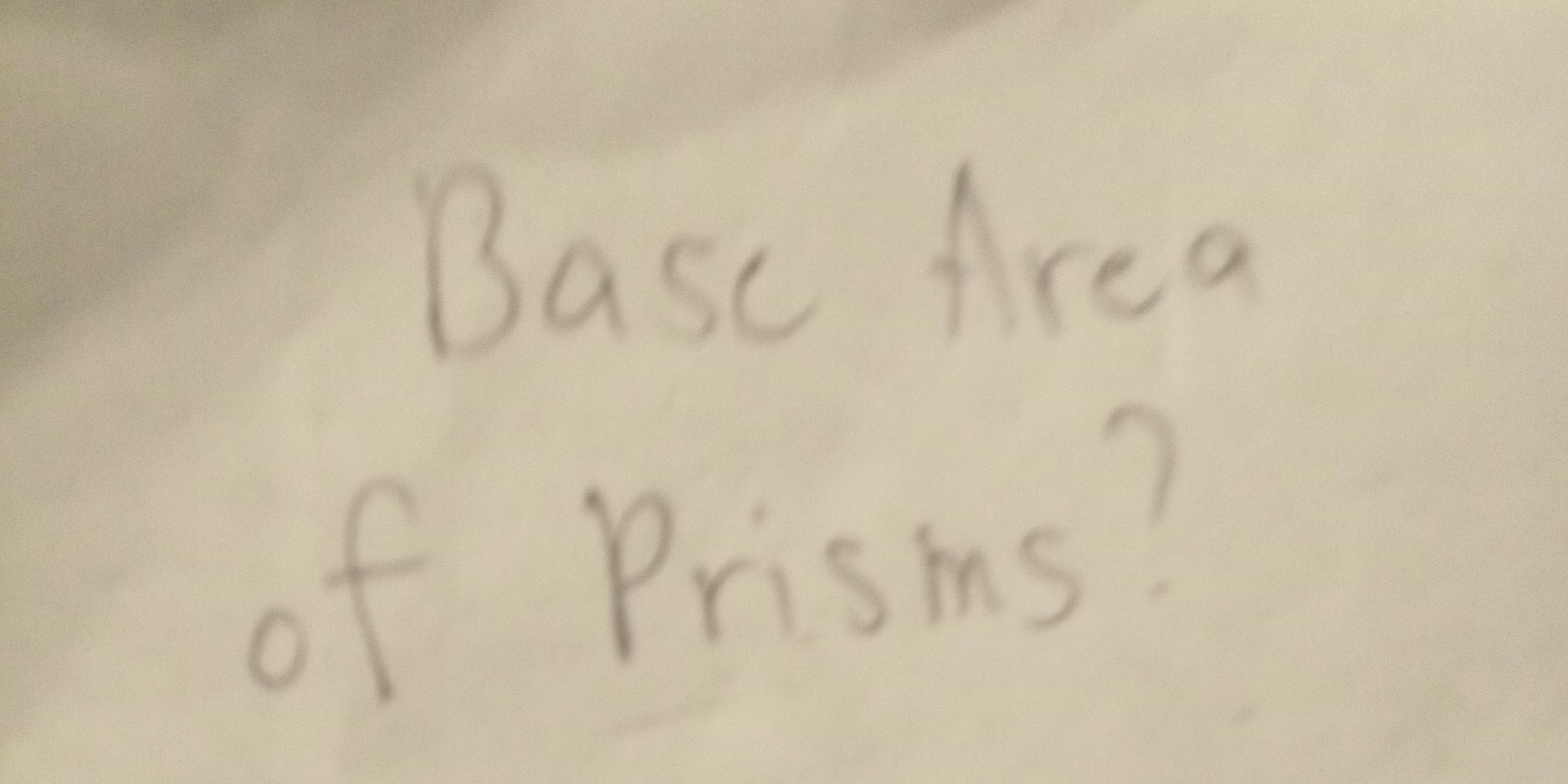
Understand the Problem
The question is asking about the base area of prisms, this means what are the formulas for calculating the base area of different prisms.
Answer
The base area of a prism depends on the shape of its base. Common formulas include $A = \frac{1}{2}bh$ (triangle), $A = s^2$ (square), $A = lw$ (rectangle), $A = \frac{1}{4} \sqrt{5(5+2\sqrt{5})} \cdot a^2$ regular pentagon, $A = \frac{3\sqrt{3}}{2}a^2$ (regular hexagon), and $A = \pi r^2$ (circle).
Answer for screen readers
The base area of a prism depends on the shape of its base. Here are some common formulas:
- Triangle: $A = \frac{1}{2}bh$
- Square: $A = s^2$
- Rectangle: $A = lw$
- Pentagon (regular): $A = \frac{1}{4} \sqrt{5(5+2\sqrt{5})} \cdot a^2$
- Hexagon (regular): $A = \frac{3\sqrt{3}}{2}a^2$
- Circle: $A = \pi r^2$
Steps to Solve
- Identify the base shape of the prism
The base of a prism can be any polygon, such as a triangle, square, rectangle, pentagon, hexagon, etc.
- Determine the area formula for the base shape
The formula to use depends on the shape of the base:
- Triangle: $A = \frac{1}{2}bh$, where $b$ is the base and $h$ is the height.
- Square: $A = s^2$, where $s$ is the side length.
- Rectangle: $A = lw$, where $l$ is the length and $w$ is the width.
- Pentagon (regular): $A = \frac{1}{4} \sqrt{5(5+2\sqrt{5})} \cdot a^2$ where $a$ is the side
- Hexagon (regular): $A = \frac{3\sqrt{3}}{2}a^2$, where $a$ is the side length.
- Circle: $A = \pi r^2$, where $r$ is the radius. Note: a prism with a circular base is usually called a cylinder instead of a prism.
- Calculate the base area
Substitute the given dimensions of the base into the appropriate area formula and calculate the area. The area will be in units squared (e.g., $cm^2$, $in^2$, $m^2$, etc.).
The base area of a prism depends on the shape of its base. Here are some common formulas:
- Triangle: $A = \frac{1}{2}bh$
- Square: $A = s^2$
- Rectangle: $A = lw$
- Pentagon (regular): $A = \frac{1}{4} \sqrt{5(5+2\sqrt{5})} \cdot a^2$
- Hexagon (regular): $A = \frac{3\sqrt{3}}{2}a^2$
- Circle: $A = \pi r^2$
More Information
The base area is essential for calculating the volume of a prism, which is given by the formula $V = Bh$, where $B$ is the base area and $h$ is the height of the prism.
Tips
- Confusing the height of the prism with the height of the base (in the case of a triangular or other non-rectangular base).
- Using the wrong formula for the area of the base shape.
AI-generated content may contain errors. Please verify critical information