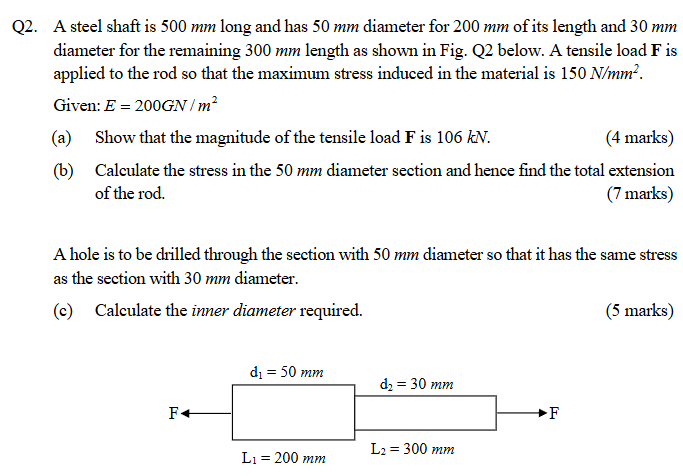
A steel shaft is 500 mm long and has 50 mm diameter for 200 mm of its length and 30 mm diameter for the remaining 300 mm length. A tensile load F is applied to the rod so that the... A steel shaft is 500 mm long and has 50 mm diameter for 200 mm of its length and 30 mm diameter for the remaining 300 mm length. A tensile load F is applied to the rod so that the maximum stress induced in the material is 150 N/mm². Given: E = 200GN/m². (a) Show that the magnitude of the tensile load F is 106 kN. (b) Calculate the stress in the 50 mm diameter section and hence find the total extension of the rod. (c) A hole is to be drilled through the section with 50 mm diameter so that it has the same stress as the section with 30 mm diameter. Calculate the inner diameter required. Read more

Steps to Solve

1. Calculate the cross-sectional area of the narrower section The maximum stress occurs in the narrower section (30 mm diameter). Calculate the area $A_2$ using the formula for the area of a circle: $A = \pi r^2$ or $A = \pi (d/2)^2$. Since we are given the diameter, the second formula is easier here.

$ A_2 = \pi (\frac{d_2}{2})^2 = \pi (\frac{30 \text{ mm}}{2})^2 = \pi (15 \text{ mm})^2 \approx 706.86 \text{ mm}^2 $

2. Calculate the tensile load F Using the stress formula, $\text{Stress} = \frac{\text{Force}}{\text{Area}}$, we can find the force $F$:

$ F = \text{Stress} \times A_2 = 150 \text{ N/mm}^2 \times 706.86 \text{ mm}^2 = 106029 \text{ N} $ Convert Newtons to kilonewtons: $F = 106029 \text{ N} \approx 106 \text{ kN}$

3. Calculate the stress in the wider section First calculate the cross-sectional area of the wider section with diameter 50 mm: $ A_1 = \pi (\frac{d_1}{2})^2 = \pi (\frac{50 \text{ mm}}{2})^2 = \pi (25 \text{ mm})^2 \approx 1963.50 \text{ mm}^2 $

Then calculate the stress $\sigma_1$ in the wider section using the tensile load $F$ and the cross-sectional area $A_1$:

$ \sigma_1 = \frac{F}{A_1} = \frac{106029 \text{ N}}{1963.50 \text{ mm}^2} \approx 54.00 \text{ N/mm}^2 $

4. Calculate the extension in each section The extension $\delta$ can be calculated using the formula $\delta = \frac{FL}{AE}$, where $F$ is the force, $L$ is the length, $A$ is the cross-sectional area, and $E$ is the Young's modulus.

For the wider section: $ \delta_1 = \frac{FL_1}{A_1E} = \frac{106029 \text{ N} \times 200 \text{ mm}}{1963.50 \text{ mm}^2 \times 200 \times 10^3 \text{ N/mm}^2} \approx 0.054 \text{ mm} $

For the narrower section: $ \delta_2 = \frac{FL_2}{A_2E} = \frac{106029 \text{ N} \times 300 \text{ mm}}{706.86 \text{ mm}^2 \times 200 \times 10^3 \text{ N/mm}^2} \approx 0.225 \text{ mm} $

5. Calculate the total extension The total extension $\delta_{\text{total}}$ is the sum of the extensions in both sections: $ \delta_{\text{total}} = \delta_1 + \delta_2 = 0.054 \text{ mm} + 0.225 \text{ mm} = 0.279 \text{ mm} $

6. Determine the required inner diameter Let $d$ be the inner diameter of the hole. The area of the wider section with the hole is $A_{\text{hole}} = \pi (\frac{d_1}{2})^2 - \pi (\frac{d}{2})^2$. We want the stress in this section to be equal to the stress in the narrower section: $ \frac{F}{A_{\text{hole}}} = 150 \text{ N/mm}^2 $ $ \frac{106029 \text{ N}}{\pi (\frac{50 \text{ mm}}{2})^2 - \pi (\frac{d}{2})^2} = 150 \text{ N/mm}^2 $ $ 106029 = 150 \times \pi ((\frac{50}{2})^2 - (\frac{d}{2})^2) $ $ \frac{106029}{150\pi} = (25)^2 - (\frac{d}{2})^2 $ $ 225.16 \approx 625 - \frac{d^2}{4} $ $ \frac{d^2}{4} = 625 - 225.16 = 399.84 $ $ d^2 = 4 \times 399.84 = 1599.36 $ $ d = \sqrt{1599.36} \approx 40.0 \text{ mm} $