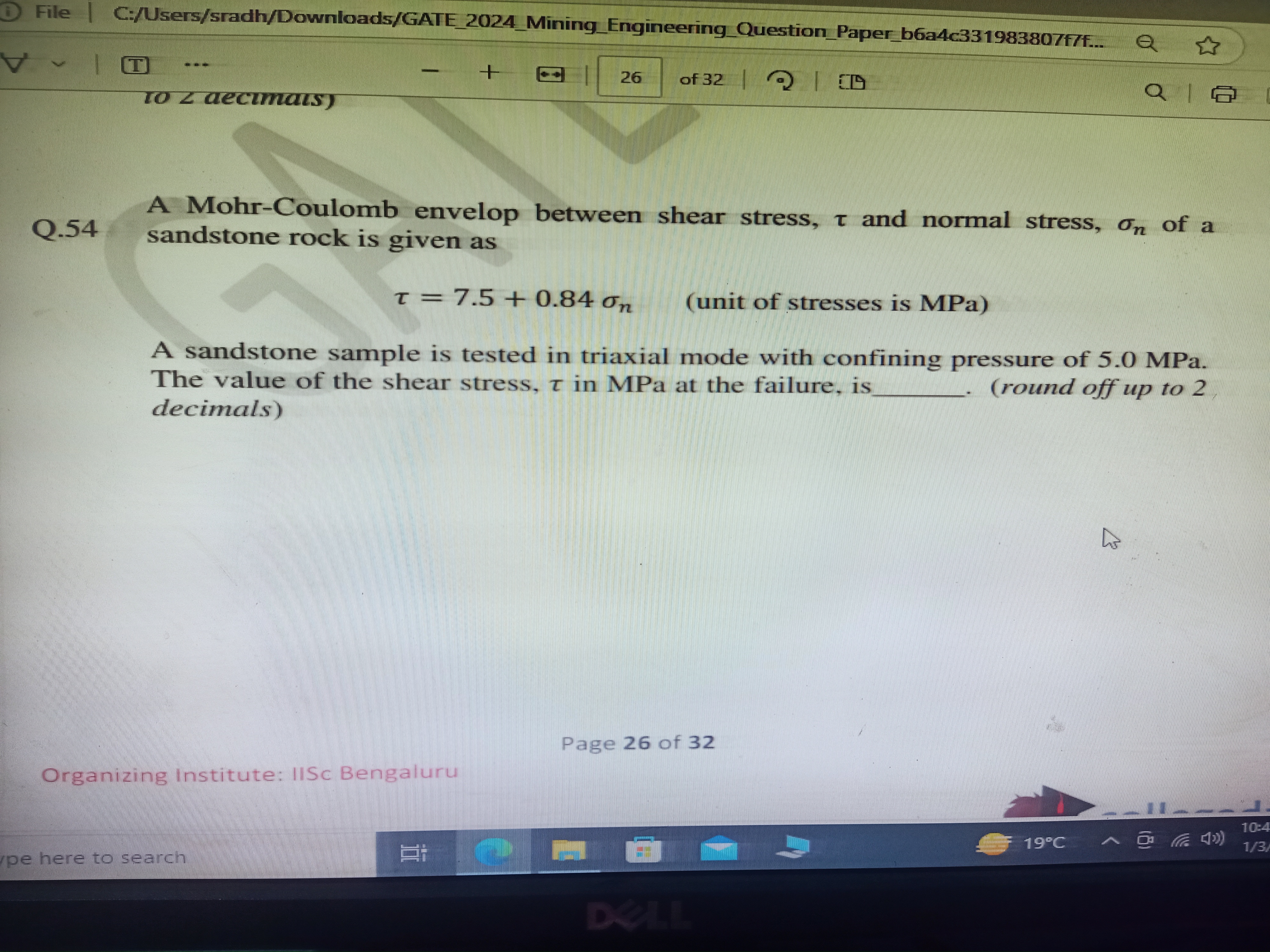
A sandstone sample is tested in triaxial mode with a confining pressure of 5.0 MPa. The value of the shear stress, tau in MPa at the failure, is __________ (round off up to 2 decim... A sandstone sample is tested in triaxial mode with a confining pressure of 5.0 MPa. The value of the shear stress, tau in MPa at the failure, is __________ (round off up to 2 decimals).
Understand the Problem
The question is asking for the value of shear stress in a sandstone sample tested under triaxial mode with a given confining pressure. It references the Mohr-Coulomb failure criteria equation to derive this value.
Answer
The shear stress at failure is $\tau = 11.70 \, \text{MPa}$.
Answer for screen readers
The value of the shear stress, $\tau$, at failure is $11.70 , \text{MPa}$.
Steps to Solve
- Identify given values We are provided with the confining pressure ($\sigma_n$) and the Mohr-Coulomb equation.
- Confining pressure ($\sigma_n$) = 5.0 MPa
- Mohr-Coulomb equation: $\tau = 7.5 + 0.84\sigma_n$
-
Substitute the confining pressure into the equation We need to replace $\sigma_n$ in the equation with the provided value: $$ \tau = 7.5 + 0.84 \times 5.0 $$
-
Calculate the shear stress Now we perform the multiplication and the addition:
- First, compute $0.84 \times 5.0 = 4.2$
- Then, add this value to 7.5: $$ \tau = 7.5 + 4.2 = 11.7 \text{ MPa} $$
- Round off the final answer We need to round the value to two decimal places, but since 11.7 is already at one decimal place, it remains: $$ \tau = 11.70 \text{ MPa} $$
The value of the shear stress, $\tau$, at failure is $11.70 , \text{MPa}$.
More Information
This value represents the shear stress at which the sandstone sample fails under triaxial testing conditions. The Mohr-Coulomb failure criterion is a common model used to describe the failure of materials under shear and normal stress conditions.
Tips
- Incorrect substitution: Ensure that the confining pressure is substituted correctly into the Mohr-Coulomb equation.
- Arithmetic errors: Always double-check calculations, especially during multiplication and addition.
- Rounding errors: When rounding, ensure you are following the specified precision requirements.
AI-generated content may contain errors. Please verify critical information