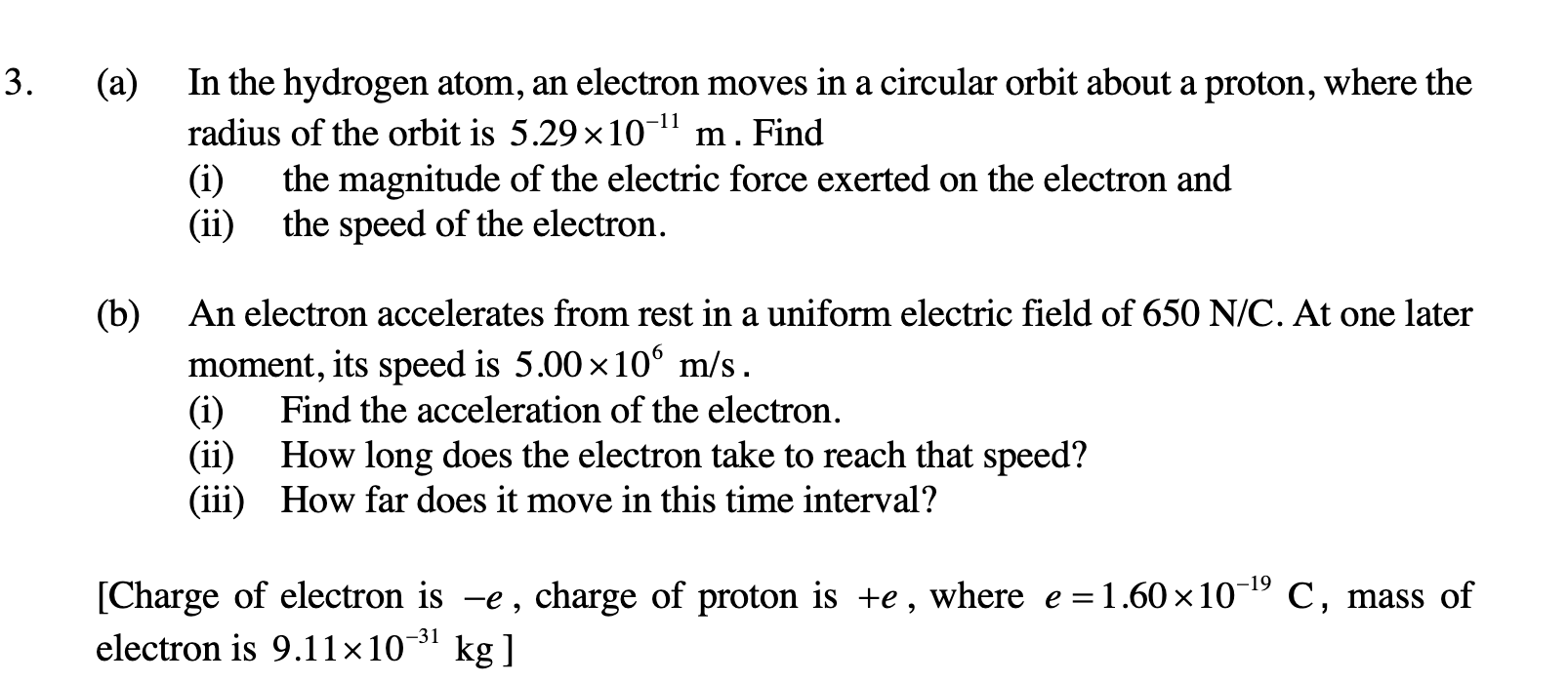
a) In the hydrogen atom, an electron moves in a circular orbit about a proton, where the radius of the orbit is 5.29 x 10^-11 m. Find: i) the magnitude of the electric force exerte... a) In the hydrogen atom, an electron moves in a circular orbit about a proton, where the radius of the orbit is 5.29 x 10^-11 m. Find: i) the magnitude of the electric force exerted on the electron and ii) the speed of the electron. b) An electron accelerates from rest in a uniform electric field of 650 N/C. At one later moment, its speed is 5.00 x 10^6 m/s. i) Find the acceleration of the electron. ii) How long does the electron take to reach that speed? iii) How far does it move in this time interval? [Charge of electron is -e, charge of proton is +e, where e = 1.60 x 10^-19 C, mass of electron is 9.11 x 10^-31 kg] Read more

Steps to Solve

1. Calculate the electric force in part (a)(i)

The magnitude of the electric force between the electron and proton is given by Coulomb's law:

$F = k \frac{|q_1 q_2|}{r^2}$

where $k = 8.9875 \times 10^9 , \text{N m}^2/\text{C}^2$, $q_1 = q_p = +1.60 \times 10^{-19} , \text{C}$, $q_2 = q_e = -1.60 \times 10^{-19} , \text{C}$, and $r = 5.29 \times 10^{-11} , \text{m}$. Substituting these values:

$F = (8.9875 \times 10^9) \frac{(1.60 \times 10^{-19})^2}{(5.29 \times 10^{-11})^2}$

2. Calculate the electron speed in part (a)(ii)

Since the electron moves in a circular orbit, the electric force provides the centripetal force:

$F = \frac{mv^2}{r}$

where $m = 9.11 \times 10^{-31} , \text{kg}$. Solving for $v$:

$v = \sqrt{\frac{Fr}{m}}$

3. Calculate the acceleration of the electron in part (b)(i)

The electric force on the electron due to the electric field is $F = qE$, and using Newton's second law, $F = ma$, we can find the acceleration:

$a = \frac{qE}{m}$

where $E = 650 , \text{N/C}$, $q = -1.60 \times 10^{-19} , \text{C}$ and $m = 9.11 \times 10^{-31} , \text{kg}$.

4. Calculate the time to reach the speed in part (b)(ii)

Using the kinematic equation $v = v_0 + at$, where $v_0 = 0 , \text{m/s}$ (starts from rest) and $v = 5.00 \times 10^6 , \text{m/s}$, we solve for $t$:

$t = \frac{v}{a}$

5. Calculate the distance traveled in part (b)(iii)

Using the kinematic equation $x = v_0t + \frac{1}{2}at^2$ with $v_0 = 0$:

$x = \frac{1}{2}at^2$