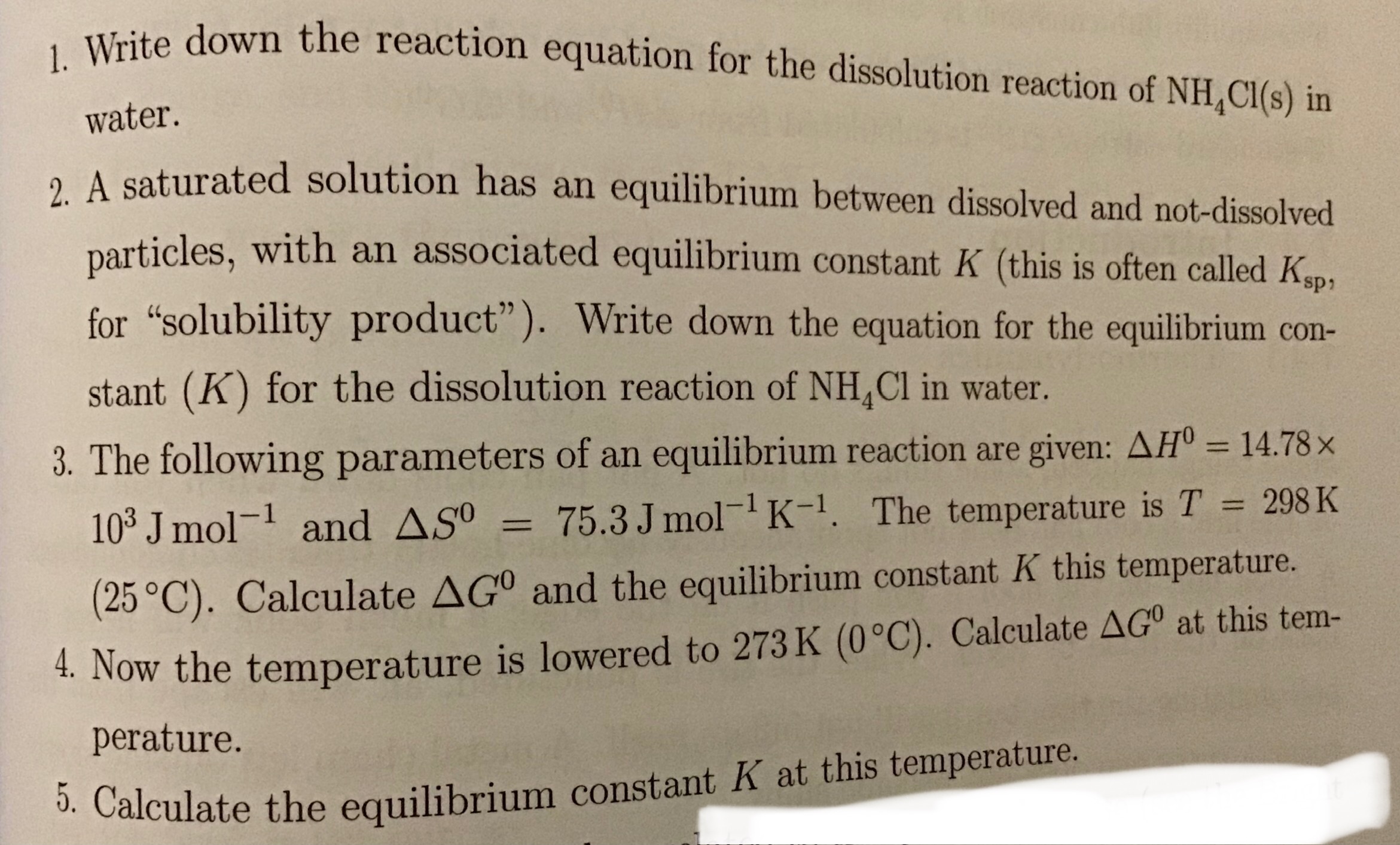
1. Write down the reaction equation for the dissolution reaction of NH4Cl(s) in water. 2. A saturated solution has an equilibrium between dissolved and not-dissolved particles, wit... 1. Write down the reaction equation for the dissolution reaction of NH4Cl(s) in water. 2. A saturated solution has an equilibrium between dissolved and not-dissolved particles, with an associated equilibrium constant K (this is often called Ksp, for "solubility product"). Write down the equation for the equilibrium constant (K) for the dissolution reaction of NH4Cl in water. 3. The following parameters of an equilibrium reaction are given: ΔΗ⁰ = 14.78 × 10^3 J mol^-1 and ΔS⁰ = 75.3J mol^-1 K^-1. The temperature is T = 298 K (25°C). Calculate ΔG and the equilibrium constant K at this temperature. 4. Now the temperature is lowered to 273K (0°C). Calculate ΔG at this temperature. 5. Calculate the equilibrium constant K at this temperature.
Understand the Problem
The questions pertain to the dissolution reaction of ammonium chloride (NH4Cl) in water, focusing on writing the reaction equation, understanding the equilibrium constant, and calculating Gibbs free energy (ΔG) and the equilibrium constant (K) at different temperatures using given thermodynamic parameters.
Answer
1. $NH_4Cl(s) \rightleftharpoons NH_4^+(aq) + Cl^-(aq)$ 2. $K = [NH_4^+][Cl^-]$ 3. $\Delta G^0 = -7659.4 \, J/mol$, $K \approx 22$ 4. $\Delta G^0 = -5776.9 \, J/mol$ 5. $K \approx 12.6$
Answer for screen readers
- $NH_4Cl(s) \rightleftharpoons NH_4^+(aq) + Cl^-(aq)$
- $K = [NH_4^+][Cl^-]$
- $\Delta G^0 = -7659.4 , J/mol$, $K \approx 22$
- $\Delta G^0 = -5776.9 , J/mol$
- $K \approx 12.6$
Steps to Solve
- Write the dissolution reaction of $NH_4Cl(s)$ in water
When ammonium chloride ($NH_4Cl$) dissolves in water, it dissociates into ammonium ions ($NH_4^+$) and chloride ions ($Cl^-$). The reaction can be written as: $NH_4Cl(s) \rightleftharpoons NH_4^+(aq) + Cl^-(aq)$
- Write the equilibrium constant (K) for the dissolution reaction
The equilibrium constant $K$ is the product of the concentrations of the products, each raised to the power of its stoichiometric coefficient. For the dissolution of $NH_4Cl$, the equilibrium constant expression is: $K = [NH_4^+][Cl^-]$
- Calculate $\Delta G^0$ and $K$ at $T = 298 K$
First, calculate $\Delta G^0$ using the formula: $\Delta G^0 = \Delta H^0 - T\Delta S^0$ Given $\Delta H^0 = 14.78 \times 10^3 J/mol$, $\Delta S^0 = 75.3 J/(mol \cdot K)$, and $T = 298 K$, we have: $\Delta G^0 = (14.78 \times 10^3 J/mol) - (298 K)(75.3 J/(mol \cdot K))$ $\Delta G^0 = 14780 J/mol - 22439.4 J/mol = -7659.4 J/mol$
Next, calculate the equilibrium constant $K$ using the formula: $K = e^{-\frac{\Delta G^0}{RT}}$ Where $R = 8.314 J/(mol \cdot K)$. Plugging in the values: $K = e^{-\frac{-7659.4 J/mol}{(8.314 J/(mol \cdot K))(298 K)}}$ $K = e^{\frac{7659.4}{2477.572}} = e^{3.091} \approx 22$
- Calculate $\Delta G^0$ at $T = 273 K$
Use the same formula as before: $\Delta G^0 = \Delta H^0 - T\Delta S^0$ Given $\Delta H^0 = 14.78 \times 10^3 J/mol$, $\Delta S^0 = 75.3 J/(mol \cdot K)$, and $T = 273 K$, we have: $\Delta G^0 = (14.78 \times 10^3 J/mol) - (273 K)(75.3 J/(mol \cdot K))$ $\Delta G^0 = 14780 J/mol - 20556.9 J/mol = -5776.9 J/mol$
- Calculate $K$ at $T = 273 K$
Use the same formula as before: $K = e^{-\frac{\Delta G^0}{RT}}$ Where $R = 8.314 J/(mol \cdot K)$. Plugging in the values: $K = e^{-\frac{-5776.9 J/mol}{(8.314 J/(mol \cdot K))(273 K)}}$ $K = e^{\frac{5776.9}{2279.722}} = e^{2.534} \approx 12.6$
- $NH_4Cl(s) \rightleftharpoons NH_4^+(aq) + Cl^-(aq)$
- $K = [NH_4^+][Cl^-]$
- $\Delta G^0 = -7659.4 , J/mol$, $K \approx 22$
- $\Delta G^0 = -5776.9 , J/mol$
- $K \approx 12.6$
More Information
The dissolution of ammonium chloride is an endothermic process, meaning it absorbs heat from the surroundings. This is reflected in the positive value of $\Delta H^0$. As temperature decreases, the solubility of ammonium chloride decreases as well, which can seen by the decrease of the equilibrium constant $K$.
Tips
- Units: Forgetting to convert all values to consistent units (e.g., J for energy, K for temperature) before calculations.
- Sign Conventions: Incorrectly applying the sign conventions for $\Delta H^0$ and $\Delta S^0$ in the Gibbs free energy equation.
- R Constant: Using wrong value for the ideal gas constant R.
AI-generated content may contain errors. Please verify critical information