Podcast
Questions and Answers
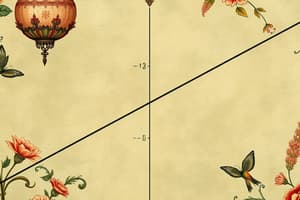
What is the slope of a line that rises from left to right?
What is the slope of a line that rises from left to right?
- Negative
- Undefined
- Zero
- Positive (correct)
A horizontal line has a positive slope.
A horizontal line has a positive slope.
False (B)
What is the formula for finding the slope given two points, (x₁, y₁) and (x₂, y₂)?
What is the formula for finding the slope given two points, (x₁, y₁) and (x₂, y₂)?
m = (y₂ - y₁) / (x₂ - x₁)
In the slope-intercept form, y = mx + b, 'b' represents the ______.
In the slope-intercept form, y = mx + b, 'b' represents the ______.
Match the forms of linear equations with their definitions:
Match the forms of linear equations with their definitions:
To solve the equation 3x + 5 = 20, what is the first step?
To solve the equation 3x + 5 = 20, what is the first step?
In a linear equation, the highest power of the variable is 2.
In a linear equation, the highest power of the variable is 2.
What do you call a line that has an undefined slope?
What do you call a line that has an undefined slope?
If a line passes through points (2, 3) and (4, 7), the slope is ______.
If a line passes through points (2, 3) and (4, 7), the slope is ______.
Which form should be used to write an equation if you have the slope and a point?
Which form should be used to write an equation if you have the slope and a point?
Flashcards
Line
Line
A straight path that extends infinitely in both directions.
Rate of change
Rate of change
The amount a quantity changes over time or in relation to another quantity.
Linear relationship
Linear relationship
A relationship where the rate of change is constant, meaning the line representing it is straight.
Slope
Slope
Signup and view all the flashcards
Positive slope
Positive slope
Signup and view all the flashcards
Negative slope
Negative slope
Signup and view all the flashcards
Zero slope
Zero slope
Signup and view all the flashcards
Undefined slope
Undefined slope
Signup and view all the flashcards
Slope-intercept form
Slope-intercept form
Signup and view all the flashcards
Point-slope form
Point-slope form
Signup and view all the flashcards
Study Notes
Lines and Linear Relationships
- Lines are straight paths that extend indefinitely in both directions.
- Rate of change describes how much a quantity changes over time or relative to another quantity. In a linear relationship, the rate of change is constant.
- Slope measures the steepness of a line. It is calculated as the vertical change (rise) divided by the horizontal change (run).
- Positive slope: line rises from left to right.
- Negative slope: line falls from left to right.
- Zero slope: horizontal line.
- Undefined slope: vertical line.
Creating and Solving Linear Relations
- A linear relation can be expressed in various forms, including:
- Slope-intercept form: y = mx + b, where 'm' is the slope and 'b' is the y-intercept (the point where the line crosses the y-axis).
- Point-slope form: y - y₁ = m(x - x₁), where 'm' is the slope and (x₁, y₁) is a point on the line.
Finding the Equation of a Line
- From the slope and a point: Use the point-slope form.
- From two points: Use the slope formula first, then the point-slope form.
Solving Linear Equations
- Linear equations involve variables to the first power.
- To solve, isolate the variable using inverse operations.
- Example methods include:
- Combining like terms
- Using the distributive property
- Adding or subtracting to both sides of the equation
- Multiplying or dividing to both sides of the equation
Creating Linear Equations
- Given specific conditions, like slope and a point or two points, use the appropriate forms of the linear equation to derive the equation for the line.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.