Podcast
Questions and Answers
What is the primary focus of algebra?
What is the primary focus of algebra?
- Analyzing statistical data
- Performing arithmetic operations
- Solving geometry problems
- Manipulation of symbols, expressions, and equations (correct)
Which of the following is an algebraic expression?
Which of the following is an algebraic expression?
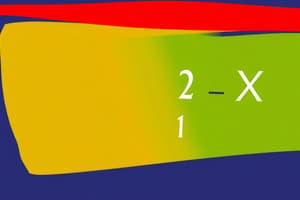
- 6 - x (correct)
- rac{3}{4} imes rac{1}{2}
- 5 + 2
- 2 imes 3
What does it mean to solve an algebraic equation?
What does it mean to solve an algebraic equation?
- Find the value of variables that make the equation true (correct)
- Find the sum of two variables
- Find the product of two variables
- Estimate the result of two variables
In algebra, what do symbols like x, y, and z represent?
In algebra, what do symbols like x, y, and z represent?
What is the first step in dividing fractions?
What is the first step in dividing fractions?
When dividing fractions, why is it necessary to find the reciprocal of a fraction?
When dividing fractions, why is it necessary to find the reciprocal of a fraction?
When dividing fractions \(rac{a}{b} \div \frac{c}{d}\), what operation should be performed?
When dividing fractions \(rac{a}{b} \div \frac{c}{d}\), what operation should be performed?
What is the result of \(rac{3}{4} imes 2\)?
What is the result of \(rac{3}{4} imes 2\)?
What are some fields where algebra is commonly used?
What are some fields where algebra is commonly used?
In dividing fractions, what should you do to the denominators?
In dividing fractions, what should you do to the denominators?
Which mathematical concept forms the foundation for calculus and probability?
Which mathematical concept forms the foundation for calculus and probability?
What are some daily tasks that involve dividing fractions?
What are some daily tasks that involve dividing fractions?
Flashcards are hidden until you start studying
Study Notes
Math: Exploring Algebra and Dividing Fractions
Math has a rich and diverse landscape, encompassing various domains that build upon each other. In this article, we'll delve into two key subtopics within the realm of mathematics: algebra and dividing fractions. By understanding these concepts, we'll be able to tackle more complex mathematical problems and make our way towards an increasingly numerate world.
Algebra
Algebra is a branch of mathematics that focuses on the manipulation of symbols, expressions, and equations. It's a fundamental tool for problem-solving, used to describe the relationships between quantities, solve problems, and make predictions.
Algebraic expressions are formed using numbers and operations like addition, subtraction, multiplication, and division. For instance, (3x + 4) is an algebraic expression, where (x) is a variable. Solving equations means finding the value of one or more variables that make the equation true.
Algebraic symbols and notation, like (x), (y), and (z), are used to represent unknowns. By using these symbols and following algebraic rules, we can describe and solve a wide range of real-world problems.
Dividing Fractions
Dividing fractions is a specific operation in which we find the reciprocal of a fraction and then multiply it by another fraction. For example, to find (\frac{3}{4} \div \frac{1}{2}), we first find the reciprocal of (\frac{1}{2}), which is (2). Then, multiply (\frac{3}{4} \times 2), which equals (\frac{6}{4}), or (\frac{3}{2}).
In general, to divide fractions (\frac{a}{b} \div \frac{c}{d}), multiply the numerators ((a \times c)) and the denominators ((b \times d)). The result will be a single fraction: (\frac{a \times c}{b \times d}).
Applications of Algebra and Dividing Fractions
Algebra and dividing fractions are interconnected and play essential roles in various fields. For instance, algebra is used in engineering, physics, and chemistry to describe physical processes and construct mathematical models. Dividing fractions, on the other hand, is used in numerous daily tasks such as cooking, budgeting, and making comparisons.
By understanding these concepts, we can become more proficient in solving complex problems and make informed decisions in our daily lives. Furthermore, algebra and dividing fractions lay the foundation for more advanced mathematical ideas, like calculus and probability.
Summary
Algebra and dividing fractions are fundamental concepts in mathematics that equip us with powerful problem-solving skills. They form the basis for more complex mathematical ideas and are used in various disciplines. By mastering these concepts, we can tackle real-world problems, make informed decisions, and continue learning new mathematical ideas. So, let's roll up our sleeves and dive into the world of mathematics, where algebra and dividing fractions await us.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.