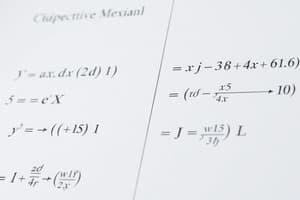Podcast
Questions and Answers
What is the main focus of calculus?
What is the main focus of calculus?
What branch of calculus involves finding the area under a curve using integration?
What branch of calculus involves finding the area under a curve using integration?
What does the derivative dx/dy represent?
What does the derivative dx/dy represent?
Which concept in calculus allows mathematicians to estimate how fast something changes without calculating every step?
Which concept in calculus allows mathematicians to estimate how fast something changes without calculating every step?
Signup and view all the answers
What is the purpose of solving equations involving integrals in calculus?
What is the purpose of solving equations involving integrals in calculus?
Signup and view all the answers
Which type of calculus applies techniques to practical situations like optimization problems?
Which type of calculus applies techniques to practical situations like optimization problems?
Signup and view all the answers
In calculus, what does the derivative measure?
In calculus, what does the derivative measure?
Signup and view all the answers
What allows people to handle difficult math problems in calculus?
What allows people to handle difficult math problems in calculus?
Signup and view all the answers
What makes up the core concept of calculus?
What makes up the core concept of calculus?
Signup and view all the answers
What is the fundamental concept of algebra that involves manipulating symbols and variables within equations and expressions?
What is the fundamental concept of algebra that involves manipulating symbols and variables within equations and expressions?
Signup and view all the answers
How can algebra be defined in simple terms?
How can algebra be defined in simple terms?
Signup and view all the answers
What is the purpose of using variables like 'x' and 'y' in algebraic equations?
What is the purpose of using variables like 'x' and 'y' in algebraic equations?
Signup and view all the answers
What role do properties of operations, equivalence relations, and groups play in algebraic theory?
What role do properties of operations, equivalence relations, and groups play in algebraic theory?
Signup and view all the answers
Which branch of mathematics involves solving problems using polynomials and exponential functions?
Which branch of mathematics involves solving problems using polynomials and exponential functions?
Signup and view all the answers
What mathematical concept is crucial to understand when transitioning from unknown values to known ones in algebra?
What mathematical concept is crucial to understand when transitioning from unknown values to known ones in algebra?
Signup and view all the answers
In algebra, what do fields, rings, and modules help make sense of?
In algebra, what do fields, rings, and modules help make sense of?
Signup and view all the answers
Which aspect of algebra involves determining how fast something changes without calculating every step?
Which aspect of algebra involves determining how fast something changes without calculating every step?
Signup and view all the answers
Study Notes
Calculus is a branch of mathematics concerned with change. It has its roots in geometry, which involves finding areas and volumes, such as finding the area under a curve using integration. In essence, it's about how things move over time and space by taking into account variables like height, distance traveled, etc., all measured with respect to other points along that path.
The most common type of calculus is differential calculus, which deals with derivatives—the rate of change of one variable relative to another. For example, if we have two quantities whose values vary together, say x and y changing simultaneously, the derivative dx/dy tells us how much x changes when y increases by one unit. Another important concept in calculus is limits, which allow mathematicians to estimate just how fast something changes without having to calculate every single step. These tools let people predict future behavior based on what they know now and give them ways to handle difficult math problems.
One key aspect of calculus is solving equations involving integrals, where the goal is to find functions f(x) that satisfy certain conditions given some function g(x). On the higher end, there's calculus of variations, which applies these techniques to practical situations such as optimization problems. As you might imagine, this can lead to very complex results! But thanks to advances in technology, many modern computer programs now perform high-level calculations automatically, making life easier for those interested in applying calculus concepts to real-life problems.
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.
Description
Explore the foundation of calculus, a mathematical branch focused on change, areas, volumes, and rates of change through derivatives. Learn about the significance of limits in estimating change, integral equations, and applications in calculus of variations for practical problem-solving. Discover how technology has streamlined complex calculations in modern times.
