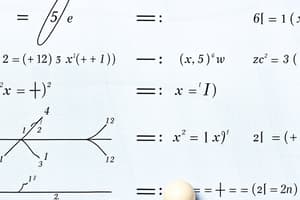Podcast
Questions and Answers
What is the name for all transformations that do not change the shape of the graph?
What is the name for all transformations that do not change the shape of the graph?
Rigid transformation
If a parabola is in standard position, what is the vertex?
If a parabola is in standard position, what is the vertex?
(0,0)
What is the parent function of a parabola in standard position?
What is the parent function of a parabola in standard position?
f(x) = x^2
What type of transformation is represented by f(x) = x^2 + 4?
What type of transformation is represented by f(x) = x^2 + 4?
Signup and view all the answers
What type of transformation is represented by f(x) = (x + 1)^2?
What type of transformation is represented by f(x) = (x + 1)^2?
Signup and view all the answers
What type of transformation is represented by F(x) = 12x^2?
What type of transformation is represented by F(x) = 12x^2?
Signup and view all the answers
What type of transformation is represented by F(x) = -x^2?
What type of transformation is represented by F(x) = -x^2?
Signup and view all the answers
What type of transformation is represented by F(x) = 1/5x^2?
What type of transformation is represented by F(x) = 1/5x^2?
Signup and view all the answers
Determine the values of F(x) = x^2 - 3 for x = -2, -1, 0, 1, 2.
Determine the values of F(x) = x^2 - 3 for x = -2, -1, 0, 1, 2.
Signup and view all the answers
Determine the values of F(x) = (x + 2)^2 for x = -4, -3, -2, -1, 0.
Determine the values of F(x) = (x + 2)^2 for x = -4, -3, -2, -1, 0.
Signup and view all the answers
Study Notes
Rigid Transformation
- Transformations that do not alter the shape of the graph are known as rigid transformations.
Parabola in Standard Position
- The vertex of a parabola in standard position is located at the coordinate point (0,0).
Parent Function of a Parabola
- The equation representing the parent function of a parabola in standard position is f(x) = x².
Vertical Translation
- The function f(x) = x² + 4 indicates a vertical translation of the parabola upward by 4 units.
Horizontal Translation
- The function f(x) = (x + 1)² represents a horizontal translation of the parabola to the left by 1 unit.
Vertical Stretch
- The function F(x) = 12x² shows a vertical stretch of the parabola due to the coefficient 12, making it narrower.
Vertical Reflection
- The function F(x) = -x² reflects the parabola vertically across the x-axis due to the negative coefficient.
Vertical Compression
- The function F(x) = (1/5)x² illustrates a vertical compression of the parabola, as it stretches out due to the coefficient being less than 1.
Specific Function Values
- For the function F(x) = x² - 3, the values of x and their corresponding F(x) outputs are:
- x = -2, F(x) = 1
- x = -1, F(x) = -2
- x = 0, F(x) = -3
- x = 1, F(x) = -2
- x = 2, F(x) = 1
Additional Specific Function Values
- For the function F(x) = (x + 2)², the values of x and their corresponding F(x) outputs are:
- x = -4, F(x) = 4
- x = -3, F(x) = 1
- x = -2, F(x) = 0
- x = -1, F(x) = 1
- x = 0, F(x) = 4
Studying That Suits You
Use AI to generate personalized quizzes and flashcards to suit your learning preferences.
Description
Test your knowledge of algebraic transformations and parabolas with these flashcards. This quiz covers rigid transformations, vertex positions, and parent functions. Perfect for reinforcing your understanding of foundational algebra concepts.
