ସମସ୍ତଙ୍କି ଯୋଗ ଏବଂ ଋତୁସମ୍ବନ୍ଧୀୟ ମାନ ବିଷୟରେ ଯାଆନ୍ତୁ।
Understand the Problem
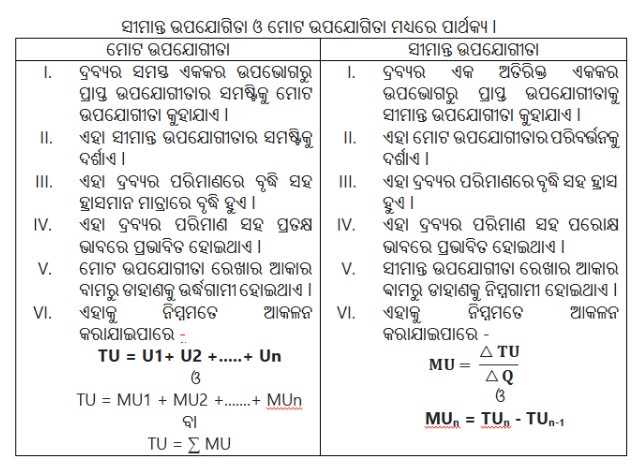
The question appears to involve formulas related to summation and possibly average calculations in a mathematical context. It likely defines terms such as Total Unit (TU), Mean Unit (MU), and other related concepts.
Answer
The formulas for total unit (TU) and mean unit (MU) are: $$ TU = U_1 + U_2 + ... + U_n $$ $$ MU = \frac{TU}{Q} $$
Answer for screen readers
The total unit (TU) is calculated by summing individual units:
$$ TU = U_1 + U_2 + ... + U_n $$
The mean unit (MU) is given by:
$$ MU = \frac{TU}{Q} $$
Steps to Solve
-
Identify total unit (TU) formulation
From the image, the total unit (TU) is defined as:
$$ TU = U_1 + U_2 + ... + U_n $$
This means TU is the sum of all individual units from $U_1$ to $U_n$. -
Understanding mean unit (MU)
The mean unit (MU) can be calculated using:
$$ MU = \frac{TU}{Q} $$
Where $Q$ represents the number of units. You will divide the total unit by the quantity to find the mean. -
Identifying the relation between MU and TU
Using the equations provided, we notice that:
$$ TU = MU_1 + MU_2 + ... + MU_n $$
This indicates that the total unit can also be expressed as the sum of mean units. -
Determine the relation of the previous unit (MU)
The previous unit mean can be expressed as:
$$ MU_{n} = TU_n - TU_{n-1} $$
This shows the relationship between consecutive mean units in the context of total units.
The total unit (TU) is calculated by summing individual units:
$$ TU = U_1 + U_2 + ... + U_n $$
The mean unit (MU) is given by:
$$ MU = \frac{TU}{Q} $$
More Information
The calculations of total and mean units are fundamental concepts in statistics and data analysis. The principle illustrated can be useful in various applications, including academic performance assessment and resource allocation.
Tips
- Neglecting to account for all units: Ensure all individual units are included in the total calculation.
- Incorrectly using Q: Be mindful to use the correct number of units for calculating the mean.
