∫ (x^2 + 4x^3)/(6x) dx
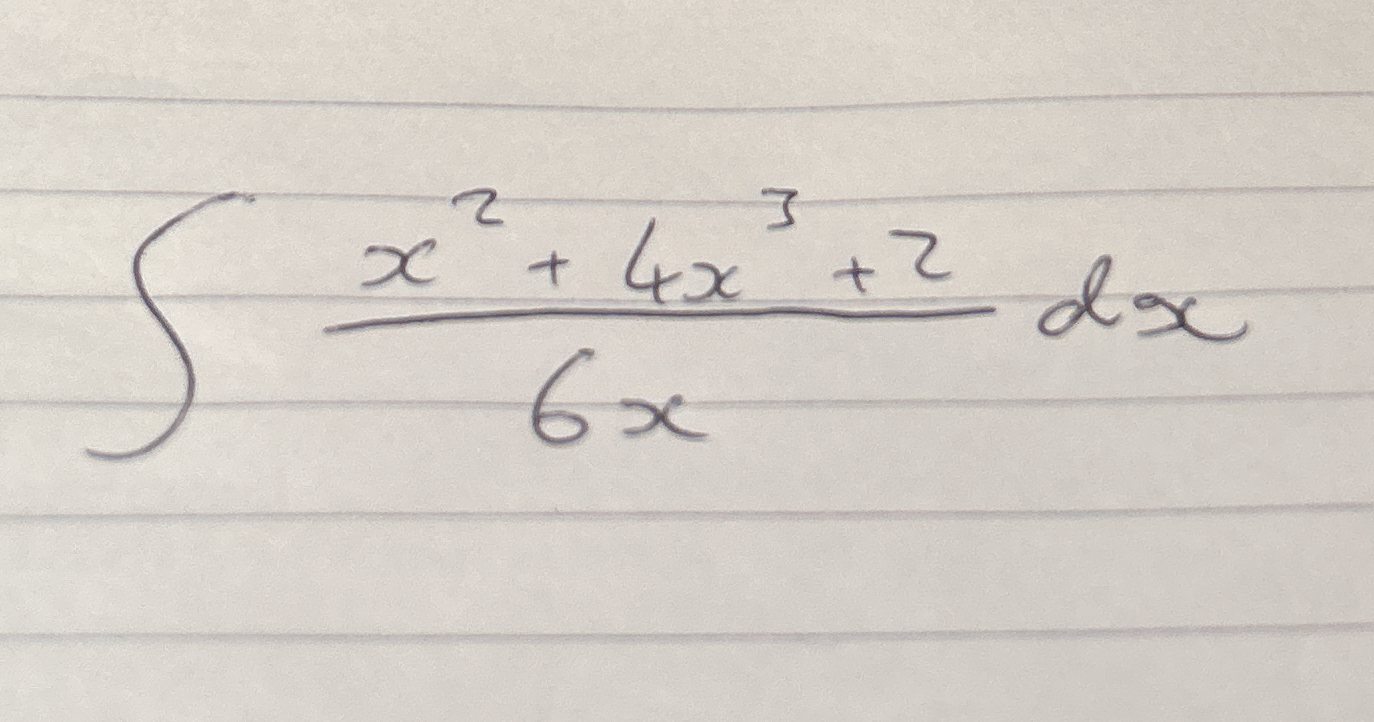
Understand the Problem
The question is asking for the evaluation of a definite integral involving a polynomial in the numerator and a linear function in the denominator. This will involve applying techniques of integration.
Answer
$$ \frac{x^2}{12} + \frac{2x^3}{9} + C. $$
Answer for screen readers
The final answer is
$$ \frac{x^2}{12} + \frac{2x^3}{9} + C. $$
Steps to Solve
- Simplifying the integrand We start with the integral
$$ \int \frac{x^2 + 4x^3}{6x} , dx. $$
We can simplify the fraction by dividing each term in the numerator by the denominator:
$$ \frac{x^2}{6x} + \frac{4x^3}{6x} = \frac{x}{6} + \frac{2x^2}{3}. $$
Thus, the integral becomes:
$$ \int \left(\frac{x}{6} + \frac{2x^2}{3}\right) , dx. $$
-
Integrating term by term Now we can integrate each term separately:
-
For $\frac{x}{6}$, we use the power rule of integration:
$$ \int \frac{x}{6} , dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12}. $$
- For $\frac{2x^2}{3}$, we again apply the power rule:
$$ \int \frac{2x^2}{3} , dx = \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2x^3}{9}. $$
So combining these results, we have:
$$ \int \left(\frac{x}{6} + \frac{2x^2}{3}\right) , dx = \frac{x^2}{12} + \frac{2x^3}{9} + C, $$
where $C$ is the constant of integration.
- Final result Thus, the final answer to the integral is:
$$ \int \frac{x^2 + 4x^3}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + C. $$
The final answer is
$$ \frac{x^2}{12} + \frac{2x^3}{9} + C. $$
More Information
This integral demonstrates the process of simplifying rational functions and integrating polynomial expressions. The constant of integration $C$ accounts for the family of antiderivatives.
Tips
- Not simplifying the integrand: It's easy to proceed without simplifying the fraction, making the integration process more complicated.
- Forgetting the constant C: Always remember to include the constant of integration when evaluating indefinite integrals.
