∫ (x² + 4x + 2) / (6x) dx
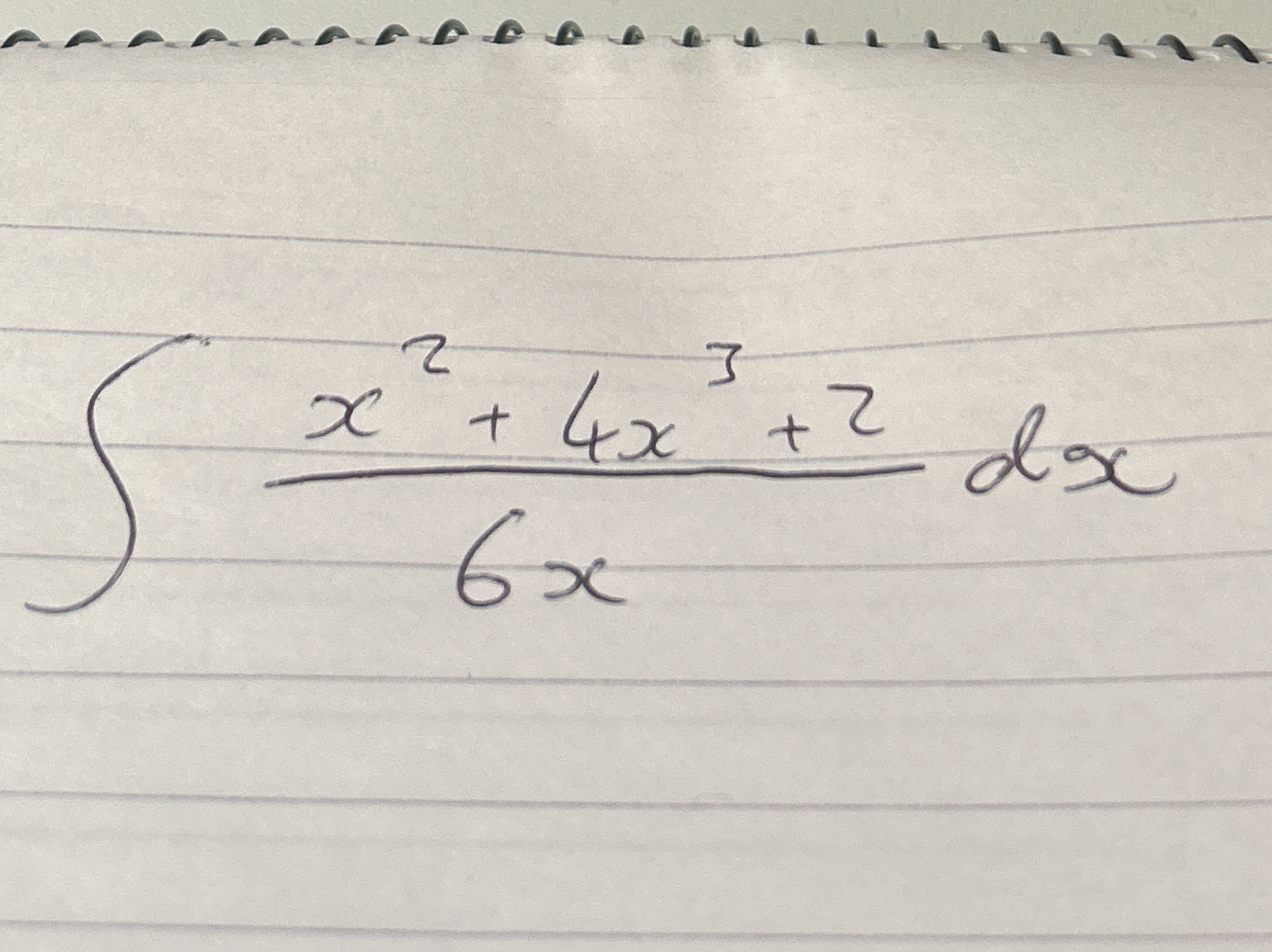
Understand the Problem
The question is asking to perform integration on the function (x^2 + 4x + 2) divided by (6x). This involves applying integral calculus to find the antiderivative of the given expression.
Answer
The integral result is $$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
The final answer is:
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
Steps to Solve
- Simplify the integrand
Split the fraction into individual terms.
$$ \frac{x^2}{6x} + \frac{4x}{6x} + \frac{2}{6x} = \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} $$
- Set up the integral
Now we can write the integral as follows:
$$ \int \left( \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} \right) dx $$
- Integrate each term
Now compute the integral of each term separately:
- The integral of $\frac{x}{6}$ is:
$$ \int \frac{x}{6} , dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} $$
- The integral of $\frac{2}{3}$ is:
$$ \int \frac{2}{3} , dx = \frac{2}{3}x $$
- The integral of $\frac{1}{3x}$ is:
$$ \int \frac{1}{3x} , dx = \frac{1}{3} \ln |x| $$
- Combine the results
Combine all the integrated terms:
$$ \int \left( \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} \right) dx = \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
where $C$ is the constant of integration.
The final answer is:
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
More Information
This integral combines polynomial and logarithmic functions. The methods used here—splitting the integrand and integrating term by term—are common techniques in calculus and can be applied to similar problems.
Tips
- Forgetting to include the constant of integration $C$.
- Misapplying the integral rules, especially for logarithmic functions.
- Not simplifying fractions before integrating.
