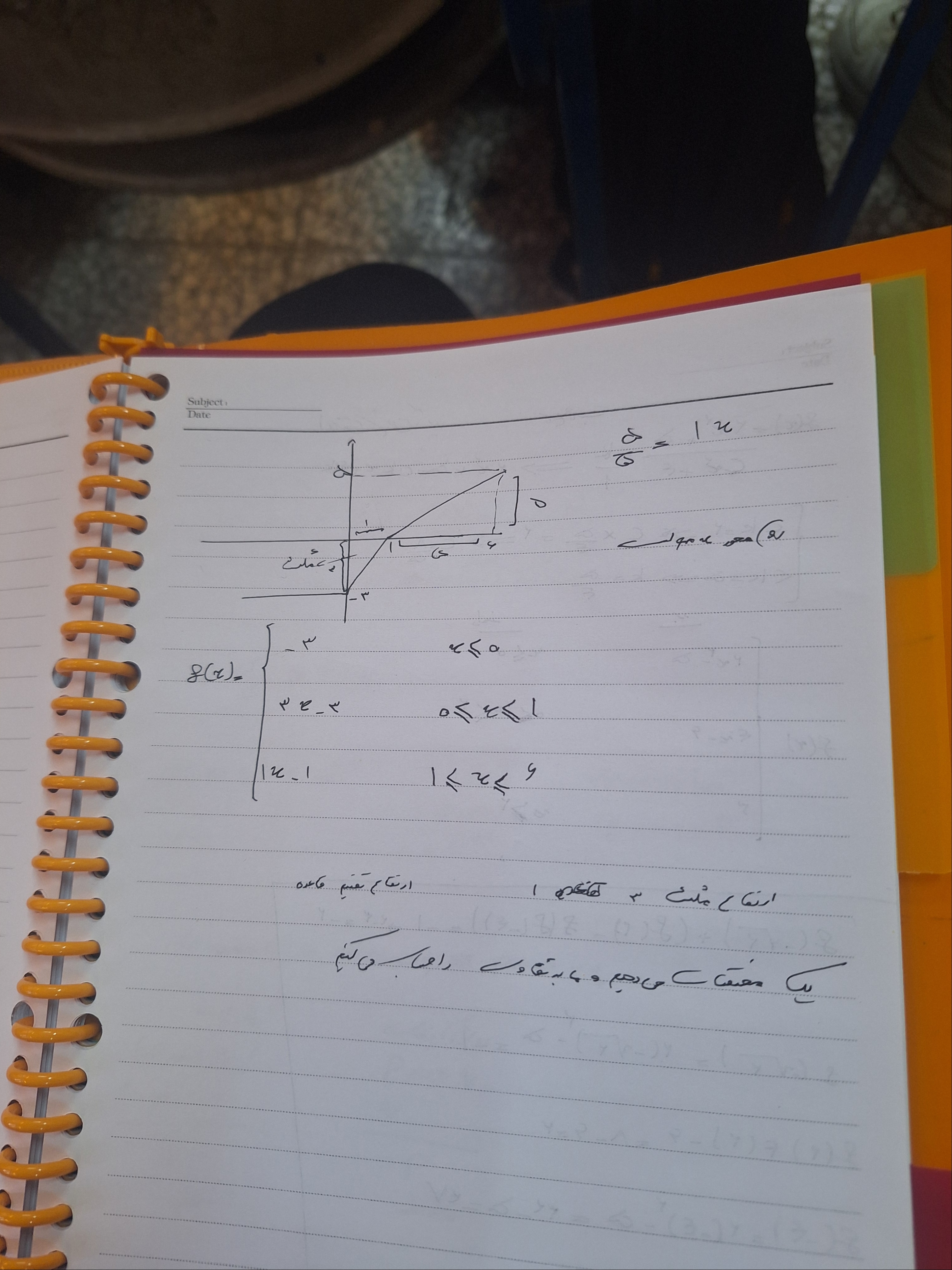
Understand the Problem
The question appears to be related to a mathematical function involving a piecewise definition, likely requiring the evaluation or understanding of that function across different intervals.
Answer
The values are $S(0.2) = 1.8$, $S(0.75) = -2.5$, and $S(1.5) = 3.5$.
Answer for screen readers
For the selected values, we have:
- $S(0.2) = 1.8$
- $S(0.75) = -2.5$
- $S(1.5) = 3.5$
Steps to Solve
-
Identify the Piecewise Function Examine the image to determine the function's definition across different intervals. The function appears to be piecewise defined.
-
Evaluate Each Interval Notice the piecewise definitions:
- For $0 \leq x < 0.5$, $S(x) = 4x + 1$
- For $0.5 \leq x < 1$, $S(x) = -4 + 2x$
- For $1 \leq x < 2$, $S(x) = 3x - 1$
- Insert Value into Appropriate Interval Select a particular $x$ value within each interval and calculate the corresponding $S(x)$:
-
For $x = 0.2$ (in the first interval):
$$ S(0.2) = 4(0.2) + 1 = 0.8 + 1 = 1.8 $$
-
For $x = 0.75$ (in the second interval):
$$ S(0.75) = -4 + 2(0.75) = -4 + 1.5 = -2.5 $$
-
For $x = 1.5$ (in the third interval):
$$ S(1.5) = 3(1.5) - 1 = 4.5 - 1 = 3.5 $$
- Draw Conclusion Summarize the values found for the selected $x$ values in their respective intervals.
For the selected values, we have:
- $S(0.2) = 1.8$
- $S(0.75) = -2.5$
- $S(1.5) = 3.5$
More Information
This piecewise function allows for different expressions depending on the interval in which $x$ falls. Function evaluations can vary significantly within different ranges, showcasing the function's flexibility.
Tips
- Failing to check which interval the $x$ value falls into can lead to incorrect evaluations.
- Misapplying the formula for the corresponding interval is a frequent error. Always verify.
AI-generated content may contain errors. Please verify critical information