x - [3x + (2 - x)] = ?
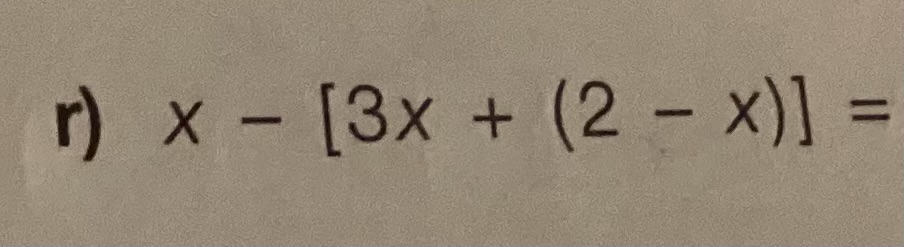
Understand the Problem
The question is asking to solve the equation that involves variables and parentheses. The goal is to simplify and rearrange the equation step by step.
Answer
The simplified expression is: $$ -x - 2 $$
Answer for screen readers
The final answer is:
$$ -x - 2 $$
Steps to Solve
- Distribute the negative sign
We start with the expression:
$$ x - [3x + (2 - x)] $$
Distributing the negative sign through the brackets:
$$ x - 3x - (2 - x) $$
This results in:
$$ x - 3x - 2 + x $$
- Combine like terms
Now we combine the like terms:
$$ (x - 3x + x) - 2 $$
Calculating the coefficients of $x$:
$$ (1 - 3 + 1)x - 2 = -x - 2 $$
- Final simplified expression
Thus, the expression simplifies to:
$$ -x - 2 $$
The final answer is:
$$ -x - 2 $$
More Information
This expression shows a linear relationship with respect to $x$. The constant term ($-2$) indicates a vertical shift downward on a graph.
Tips
- Not distributing the negative sign properly: Always ensure you distribute to all terms inside the brackets.
- Forgetting to combine like terms: Make sure to carefully add or subtract coefficients for like variables.
AI-generated content may contain errors. Please verify critical information