Write the equation of the line graphed below in simplest form.
Understand the Problem
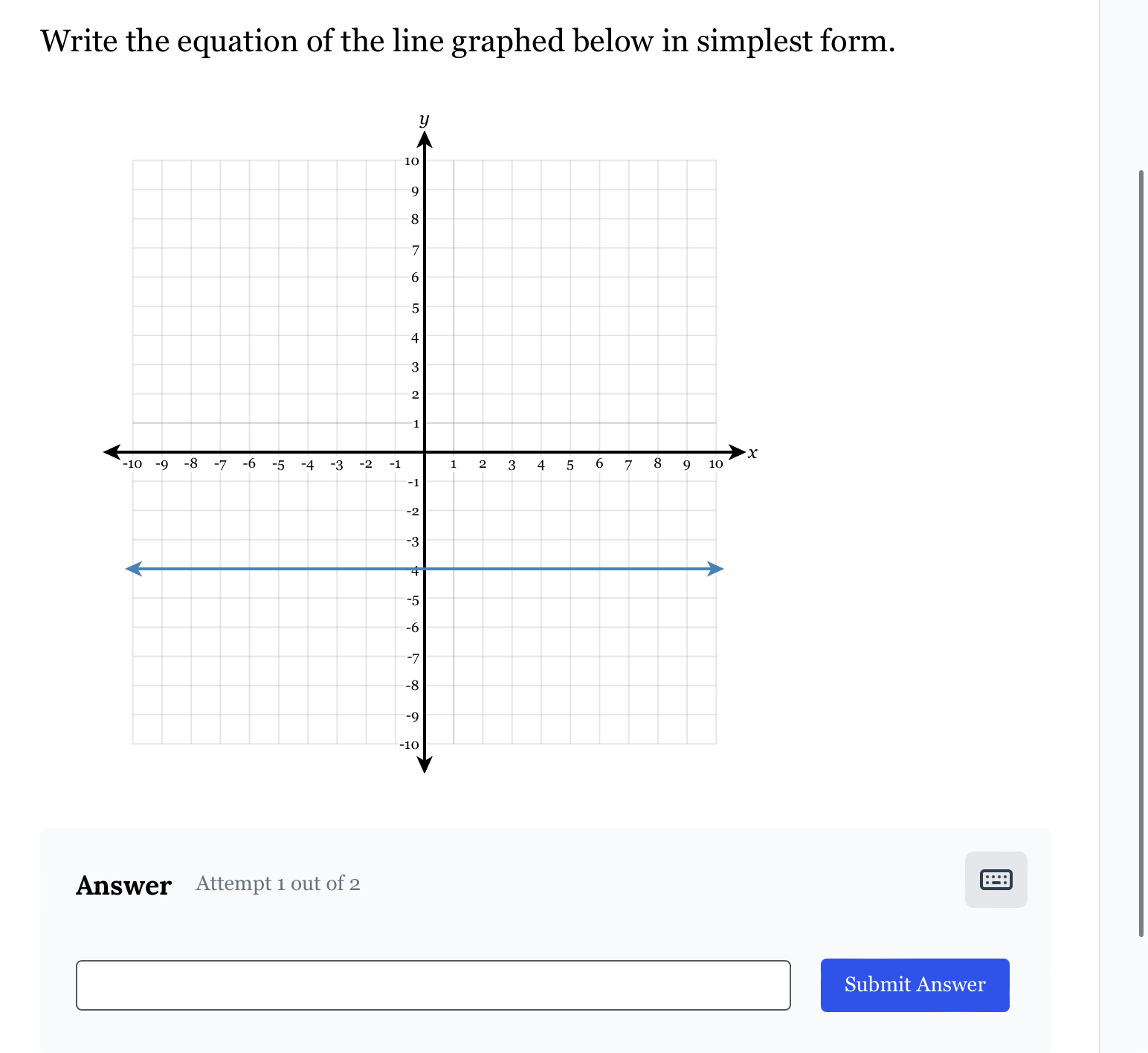
The question is asking for the equation of a line that is represented graphically. To obtain this equation, we typically identify the slope and y-intercept of the line from the graph.
Answer
The equation of the line is $y = -3$.
Answer for screen readers
The equation of the line is $y = -3$.
Steps to Solve
- Identify the slope of the line
From the graph, observe that the line is horizontal. A horizontal line has a slope ($m$) of 0, since it does not rise or fall as $x$ changes.
- Identify the y-intercept
The y-intercept is the point where the line crosses the y-axis. From the graph, the line crosses the y-axis at $y = -3$.
- Write the equation in slope-intercept form
The slope-intercept form of a line is given by the equation:
$$ y = mx + b $$
Where $m$ is the slope and $b$ is the y-intercept. Substituting the values identified:
$$ y = 0x - 3 $$
This simplifies to:
$$ y = -3 $$
The equation of the line is $y = -3$.
More Information
This equation represents a horizontal line that stays at $y = -3$ for all values of $x$. Horizontal lines have a slope of 0 and can be easily identified on a graph.
Tips
- Confusing a horizontal line with other lines: Ensure to recognize that horizontal lines have a slope of zero.
- Misidentifying the y-intercept: Always double-check where the line crosses the y-axis.
