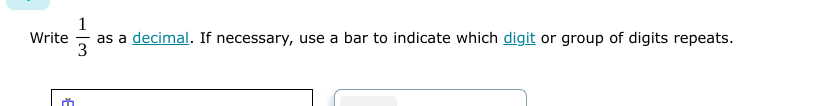
Write 1/3 as a decimal. If necessary, use a bar to indicate which digit or group of digits repeats.
Understand the Problem
The question asks for the fraction 1/3 to be converted into a decimal format. It also specifies that if there are repeating digits, a bar should be used to indicate which digit or group of digits repeats. This implies a conversion involving division and understanding of recurring decimals.
Answer
The decimal for \(\frac{1}{3}\) is \(0.\overline{3}\).
Answer for screen readers
The decimal representation of (\frac{1}{3}) is (0.\overline{3}).
Steps to Solve
-
Performing the division We need to divide 1 by 3. This can be set up as (1 \div 3).
-
Setting up the long division Set up the long division:
- Start with 1.0. Since 1 is less than 3, we can add a decimal point and a zero, making it 10.
- Divide 10 by 3 3 goes into 10 three times (since (3 \times 3 = 9)).
- Write 3 in the decimal place and subtract (9) from (10), leaving a remainder of (1).
-
Continue the division Bring down another (0) to make it (10) again. Again, (3) goes into (10) three times, leading to the same step as before.
-
Identify the repeating decimal Since we keep getting (10) and dividing again by (3) each time, we realize that digit (3) is repeating. Thus, we write it as (0.\overline{3}).
The decimal representation of (\frac{1}{3}) is (0.\overline{3}).
More Information
The decimal (0.\overline{3}) indicates that the digit (3) repeats infinitely. This is a common result when dividing by (3) since (1) cannot be evenly divided by (3), resulting in a recurring decimal.
Tips
- Misplacing the decimal point: Sometimes, students forget to align the decimal during division, leading to incorrect values.
- Not recognizing the repeating digit: It's important to see that the digits repeat indefinitely and denote this with a bar.
AI-generated content may contain errors. Please verify critical information