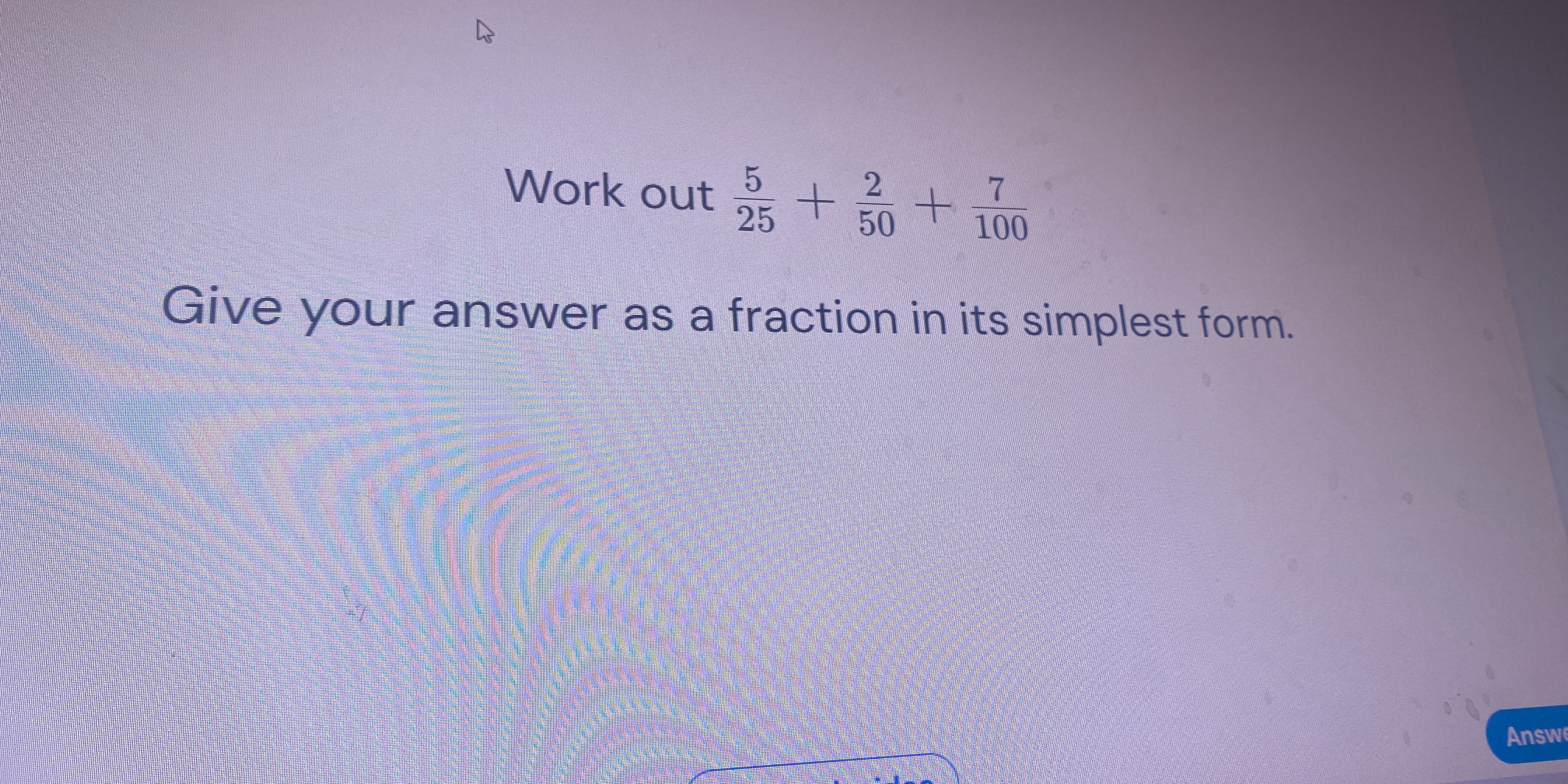
Work out 5/25 + 2/50 + 7/100. Give your answer as a fraction in its simplest form.
Understand the Problem
The question is asking to perform a mathematical operation by adding the given fractions (5/25, 2/50, and 7/100) and then simplifying the result to its simplest form.
Answer
$\frac{31}{100}$
Answer for screen readers
The answer is $\frac{31}{100}$.
Steps to Solve
- Identify a common denominator
To add the fractions $\frac{5}{25}$, $\frac{2}{50}$, and $\frac{7}{100}$, we need to find a common denominator. The denominators are 25, 50, and 100. The least common multiple (LCM) of these numbers is 100.
- Convert fractions to have the common denominator
Next, we convert each fraction to have the common denominator of 100:
-
For $\frac{5}{25}$:
$$ \frac{5}{25} = \frac{5 \times 4}{25 \times 4} = \frac{20}{100} $$
-
For $\frac{2}{50}$:
$$ \frac{2}{50} = \frac{2 \times 2}{50 \times 2} = \frac{4}{100} $$
-
$\frac{7}{100}$ is already in the correct form.
- Add the fractions
Now that all fractions have the same denominator, we can add them together:
$$ \frac{20}{100} + \frac{4}{100} + \frac{7}{100} = \frac{20 + 4 + 7}{100} = \frac{31}{100} $$
- Simplify the result
The fraction $\frac{31}{100}$ is already in its simplest form since 31 and 100 have no common factors other than 1.
The answer is $\frac{31}{100}$.
More Information
The fraction $\frac{31}{100}$ represents the combined value of the three fractions after simplification. It is common to encounter fractions in various contexts, such as measurements or probabilities, where finding a common denominator is essential for addition.
Tips
- Failing to find the least common multiple (LCM) correctly, leading to errors in adding fractions.
- Forgetting to simplify the final answer, which can result in an unnecessarily complex fraction.
AI-generated content may contain errors. Please verify critical information