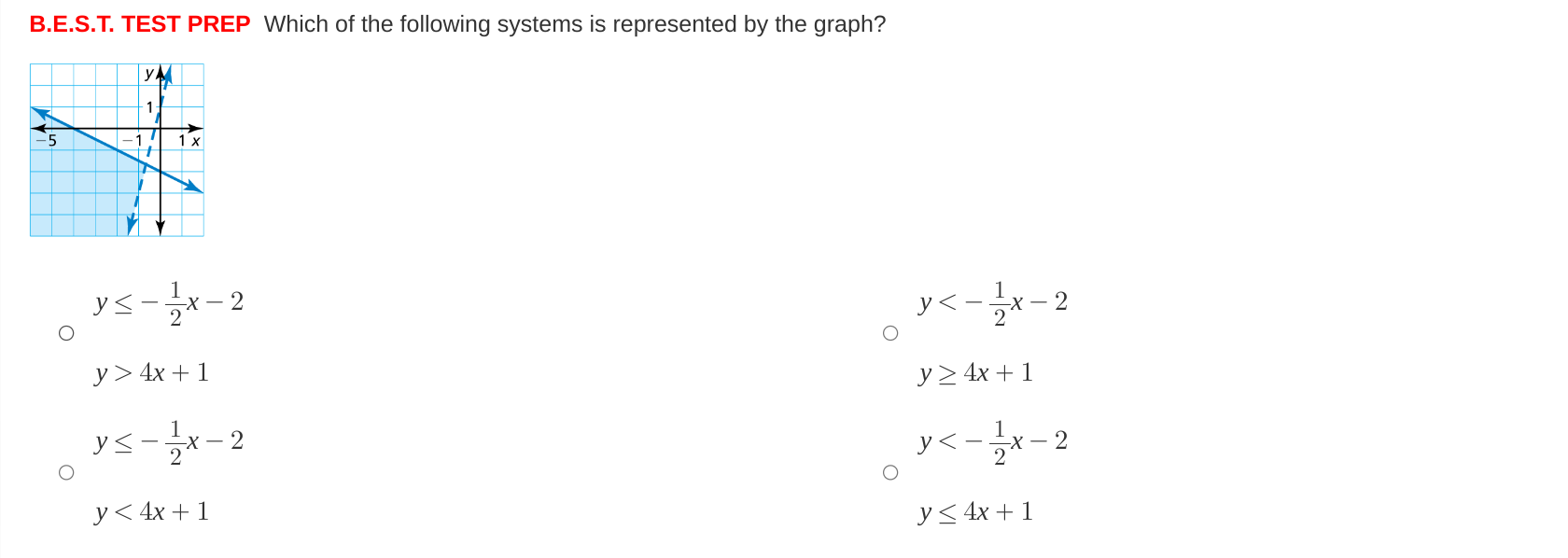
Which of the following systems is represented by the graph?
Understand the Problem
The question is asking to identify which of the provided systems of inequalities correctly corresponds to the graph shown. To solve it, we will analyze the slope and intercepts derived from the graph and compare them with the given options.
Answer
The inequality represented by the graph is $y \leq -\frac{1}{2}x - 2$.
Answer for screen readers
The correct system of inequalities represented by the graph is: $$ y \leq -\frac{1}{2}x - 2 $$
Steps to Solve
- Identify the Slope and Intercept from the Graph
From the graph, observe the line's direction and calculate its slope. The line appears to decrease from left to right. To find the slope, pick two points on the line. The points (0, -2) and (2, -3) can be used.
The slope ( m ) is calculated as follows: $$ m = \frac{y_2 - y_1}{x_2 - x_1} = \frac{-3 - (-2)}{2 - 0} = \frac{-1}{2} $$
Thus, the slope is ( -\frac{1}{2} ).
- Write the Equation of the Line
Use the slope-intercept form ( y = mx + b ) where ( b ) is the y-intercept. From the graph, the y-intercept is -2. Therefore, the equation is: $$ y = -\frac{1}{2}x - 2 $$
- Determine the Inequality Direction
Since the area above the line is shaded, the inequality must be less than (indicating the region below the line). Thus, we have: $$ y \leq -\frac{1}{2}x - 2 $$
- Compare with Given Options
Now, look at the provided multiple-choice answers. The correct inequality corresponding to our analysis is: $$ y \leq -\frac{1}{2}x - 2 $$
The correct system of inequalities represented by the graph is: $$ y \leq -\frac{1}{2}x - 2 $$
More Information
This problem involves understanding how to interpret a graph of linear inequalities. The slope of the line and the shading help determine the correct inequality. Lines that slope downwards typically have a negative slope, and the y-intercept indicates where the line crosses the y-axis.
Tips
- Confusing the direction of the inequality: Ensure to check the shaded region relative to the line.
- Miscalculating the slope or intercept: Always double-check the points used to find the slope and the y-intercept.
AI-generated content may contain errors. Please verify critical information