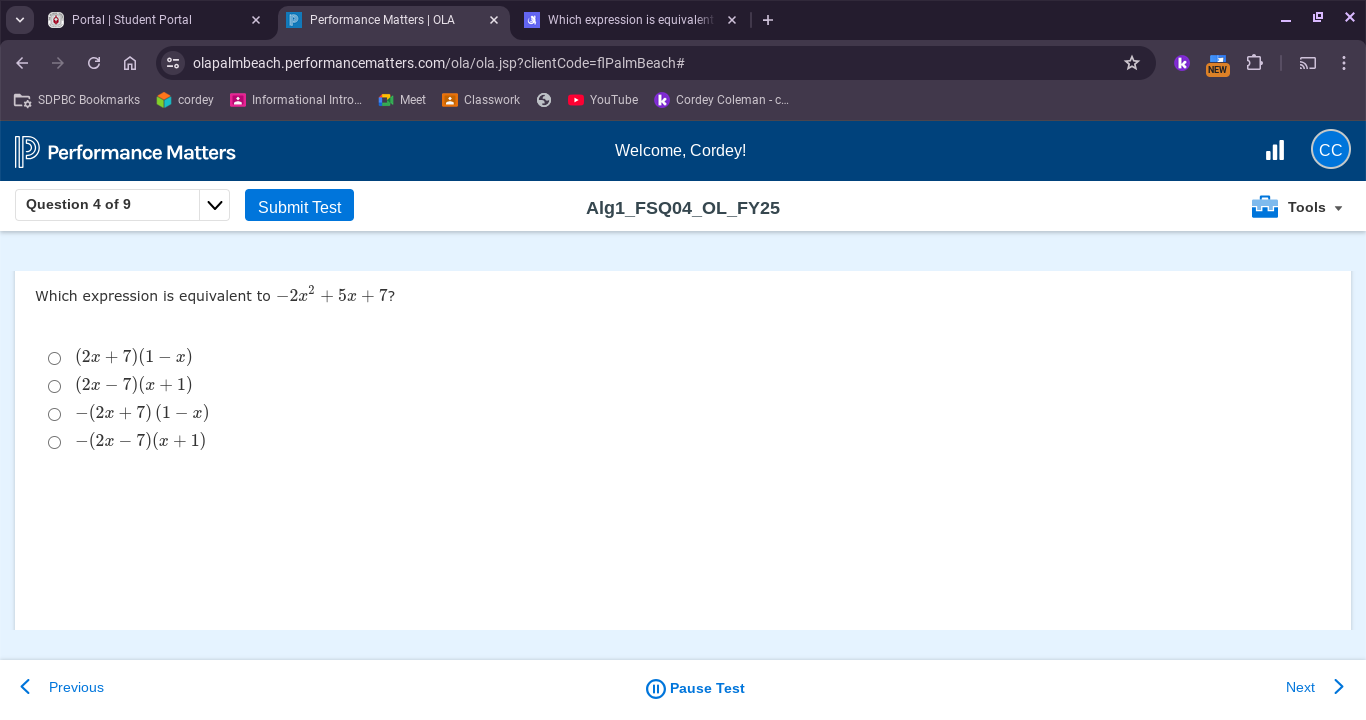
Which expression is equivalent to $-2x^2 + 5x + 7$?
Understand the Problem
The question asks to identify the expression equivalent to the quadratic expression $-2x^2 + 5x + 7$. This requires expanding each of the provided expressions and comparing them to the original quadratic.
Answer
$-(2x-7)(x+1)$
Answer for screen readers
$-(2x-7)(x+1)$
Steps to Solve
- Expand the first expression: $(2x+7)(1-x)$
Multiply the terms using the distributive property (FOIL method): $(2x+7)(1-x) = 2x(1) + 2x(-x) + 7(1) + 7(-x) = 2x - 2x^2 + 7 - 7x = -2x^2 - 5x + 7$
- Expand the second expression: $(2x-7)(x+1)$
Multiply the terms using the distributive property (FOIL method): $(2x-7)(x+1) = 2x(x) + 2x(1) -7(x) - 7(1) = 2x^2 + 2x - 7x - 7 = 2x^2 - 5x - 7$
- Expand the third expression: $-(2x+7)(1-x)$
First, expand $(2x+7)(1-x)$ which we already found to be $-2x^2 - 5x + 7$. Then, multiply by $-1$: $-(-2x^2 - 5x + 7) = 2x^2 + 5x - 7$
- Expand the fourth expression: $-(2x-7)(x+1)$
First, expand $(2x-7)(x+1)$ which we already found to be $2x^2 - 5x - 7$. Then, multiply by $-1$: $-(2x^2 - 5x - 7) = -2x^2 + 5x + 7$
- Identify the equivalent expression
The expanded form of $-(2x-7)(x+1)$ is $-2x^2 + 5x + 7$, which matches the given expression.
$-(2x-7)(x+1)$
More Information
Expanding algebraic expressions is a fundamental skill in algebra, allowing us to manipulate and simplify equations.
Tips
A common mistake is making errors when distributing the negative sign in expressions like $-(2x-7)(x+1)$. For example, forgetting to distribute the negative sign to all terms inside can lead to an incorrect result. Careless arithmetic errors during expansion are also common.
AI-generated content may contain errors. Please verify critical information